(https://publish.obsidian.md/shanesql/Library/Besseliuan+Interpolation)
All PDFs downloadable:
files.aethercosmology.com/s/EclipsePDFs
[https://files.aethercosmology.com/account/shares](https://files.aethercosmology.com/account/shares)

## Preface
What do I have to do to understand eclipse cycles Steve?
(mock interview with this video)
##### PSU math prof: Here's how to predict eclipses
[https://www.youtube.com/watch?v=w9CM_MxG1vQ](https://www.youtube.com/watch?v=w9CM_MxG1vQ)
10,660 views Aug 17, 2017
Steven Bleiler, a professor of mathematics and statistics at Portland State University, explained how eclipses can be predicted by anyone using basic tools found around the house.
Why their model doesnt work with shadows
https://x.com/TedLogan1010/status/1687262038207107073
According to Steve:
To predict eclipses, you'll need:
1. **Observational Tools:**
- A vertical stick or pole.
- A timepiece for accurate timing.
- Angle-measuring devices such as protractors or improvised tools like two sticks with a nail between them.
2. **Astronomical Data (optional)**
- Access to astronomical databases or almanacs containing information on celestial bodies' positions and movements.
3. **Mathematical Understanding:**
- Basic mathematical knowledge to calculate and interpret data.
- Understanding of astronomical cycles like the synodic, anomalistic, and draconic months.
4. **Historical Data:**
- Access to historical records and tables of past eclipses to analyze patterns and trends.
5. **Computational Tools (Optional):**
- Software or computer programs for complex mathematical calculations and modeling.
By combining observational data, mathematical calculations, and historical records, you can predict eclipses accurately. Turns out, ANYONE can do it.
```embed
title: "The Grand Eclipse Archive [ShanesEclipse Walkthrough]"
image: "https://img.youtube.com/vi/B49tP2wk_LE/maxresdefault.jpg"
description: "The Grand Eclipse Archive Shanes [Complete Eclipse Walkthrough]From Lunar Cycles to innos cycles, this has it all. Every single thing I have learned about ec…"
url: "https://www.youtube.com/watch?v=B49tP2wk_LE"
```
### Historicity of Solar and Lunar Cycles as Measures of Time
The Lunar Cycle offers a significant advantage as it precisely determines the succession of years and days. For example, the Ancient Hebrew Solar Cycle commenced with the year 1722. Consequently, every 285th year must mirror the first in terms of the dates of the Sabbath days. The consistency of historical dates with this pattern serves as solid evidence of an accurate and consecutive record. Moreover, it's noteworthy that the first year of the Cycle alternately ends with the digits 2 or 7.
In the context of Antediluvian times, the Lunar Cycle's systematic precision spans 133 years. This intricately designed cycle inspires admiration for its grand work. Rather than doubting its efficacy, we should embrace and appreciate the remarkable harmony it brings to timekeeping and historical records.
The histories of Babylon and Assyria align well with the Lunar Cycle and are closely related to Scripture, implying a level of credibility. However, Chinese history, specifically events before 1796 A.M. (anno mundi, Latin for "in the year of the world"), is considered purely mythological, meaning it contains legendary or fictional elements rather than factual historical events.
The Lunar Cycle's association with historical records, particularly in Babylon and Assyria, provides a systematic way of validating the accuracy and chronological consistency of accounts. On the other hand, Chinese history, prior to the mentioned date, is regarded as more mythological in nature, possibly due to its reliance on ancient legends, folklore, and oral traditions that lack solid verifiable historical evidence.
As the moon revolves around the earth in twenty nine and one-half days, the twelve months of the year must alternately possess thirty and twenty-nine days in order to keep up her movements—twelve lunations or months (Moon-eths,) in a year. This is a system now followed by the Jews, Turks and Chinese, w ho use the original Lunar Year, the months of which are more astronomical than our Pagan, Solar year, which, although a perfect measure of time, can begin and end anywhere, and have months of irregular length. The Lunar year, and each month of the year, must begin with a new moon.
# Introduction - Shane's piece
(in the words of shane)
We are going to take a look at the complicated series of Saros cycles' that completely break down the celestial cycle into its simplest form. This knowledge has been forgotten and occulted, then weaponized against us. I think it is pivotal that we can understand the cyclical nature of the sky, but before we can understand the macro, we must understand the micro. The lunar cycle or saros is the smallest part of a larger collection of cycles within cycles, that by their appearance in the heavens, mark periods of time for all to know. The sky functions as a perfect clock, except most of the world has forgotten how to read it.
I think the concept of time is the one of the most thoroughly messed with concepts throughout all of history. I think with things like intercalary days, leap years, time zones, 12 vs 24 hour clocks, daylight savings time, etc, it has become almost impossible to keep up with the exact procession of days, hours, and minutes as they continuously flow. We have naturally grown dependent on technology to tell us the time and important events associated with future time, but there has also been a decidedly unnatural massive push to remove this from our everyday lives. I won't go into detail here, that isn't the point of this particular presentation, but it will be the subject of the next one.
Because it has grown so difficult to for most people to keep up with the changes to the ways we keep time, we have acclimated to not being able to discern it for ourselves by looking into the heavens. This, my friends, is what has made us so susceptible to the deceptions of the modern age. Since we are so dependent on other men to tell us what time it is, we have allowed them to also tell us how long it has been, and how long there has ever been. This just means, like when the victor writes the history of a war, the results become skewed. Essentially, all of human history has now been filtered through this process, and our current collective knowledge of our entire past is in jeopardy. All of the events of our past, right down to those in the bible are relayed with chronologic markers intended for us to be able to track them backward through time from our current positions. However, as you may have noticed, that has become extremely difficult. We not only have so many small amendments to figure out and apply, but giant sweeping changes, like complete calendar changes, or switching from a solar form of time keeping to a lunar form, and re dating all past events in line with the new system. Anyway, the point is, if you aim to lie about the history of a people, it is tremendously helpful to be able to change the dates of certain events around to better suit your current standing or goals. In fact, it would be impossible to lie about history without also lying about time. This is why I put such importance on the understanding of the celestial clock.
This presentation is soley about the celestial cycles. I will try to remain focused and concise as we go through an absurd amount of information relating to the saros cycle, but there are many components required for a full understanding. We will touch briefly on things that should rightly be an entire course at colleges, and I am going to keep it as high level as possible to make sure everyone to understand the conclusion.
Here.... we.... go....
# The Basics - The sun and The Moon
First, we need to go over the celestial bodies and what they do.
The Sun.
The Moon.
Ever since Keplar, man has taken notice of the celestial bodies and their travels across the sky. Of course, Newton came along and fucked that up by inserting mass and adding gravity, but that is a whole different presentation.
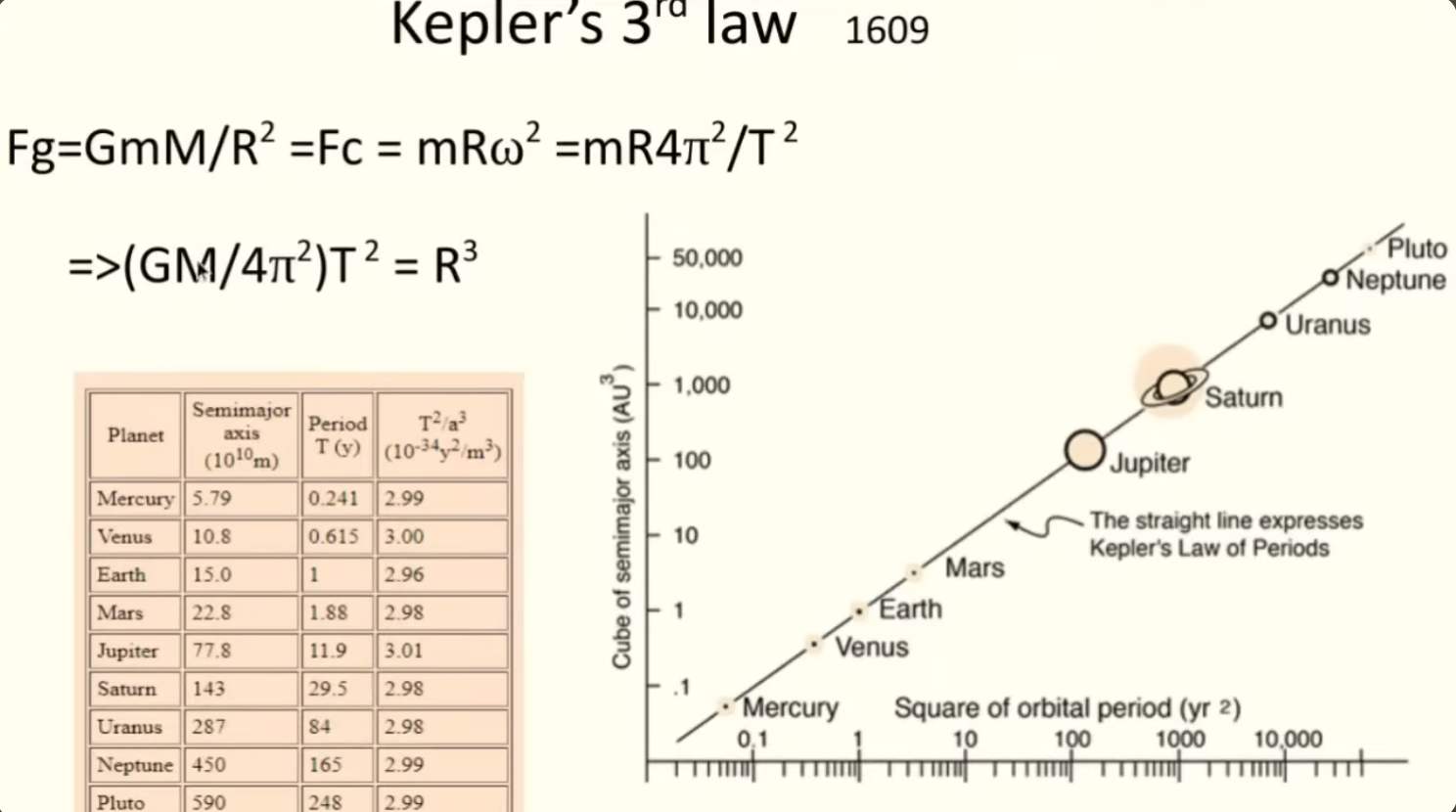
For more on that, check out [https://publish.obsidian.md/shanesql/Kinematics+vs+Dynamics](https://publish.obsidian.md/shanesql/Kinematics+vs+Dynamics)
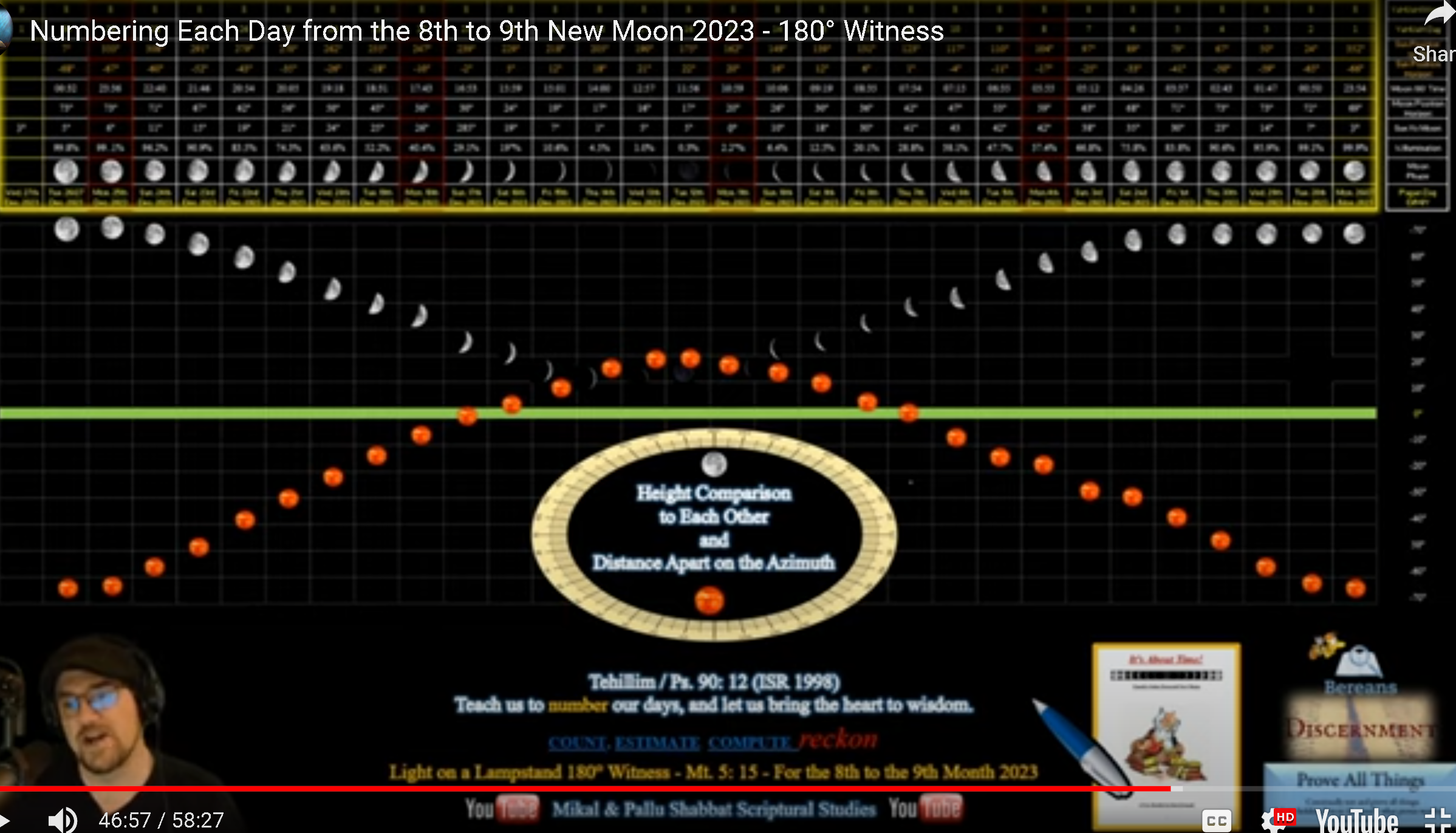
The path of the sun and moon create every cycle on earth. Our concept of time is directly dependent on the sun and the day night cycle. The complicated geometry that results from the paths of the sun and moon as they process over earth is a simple premise that ends up creating very complicated patterns.
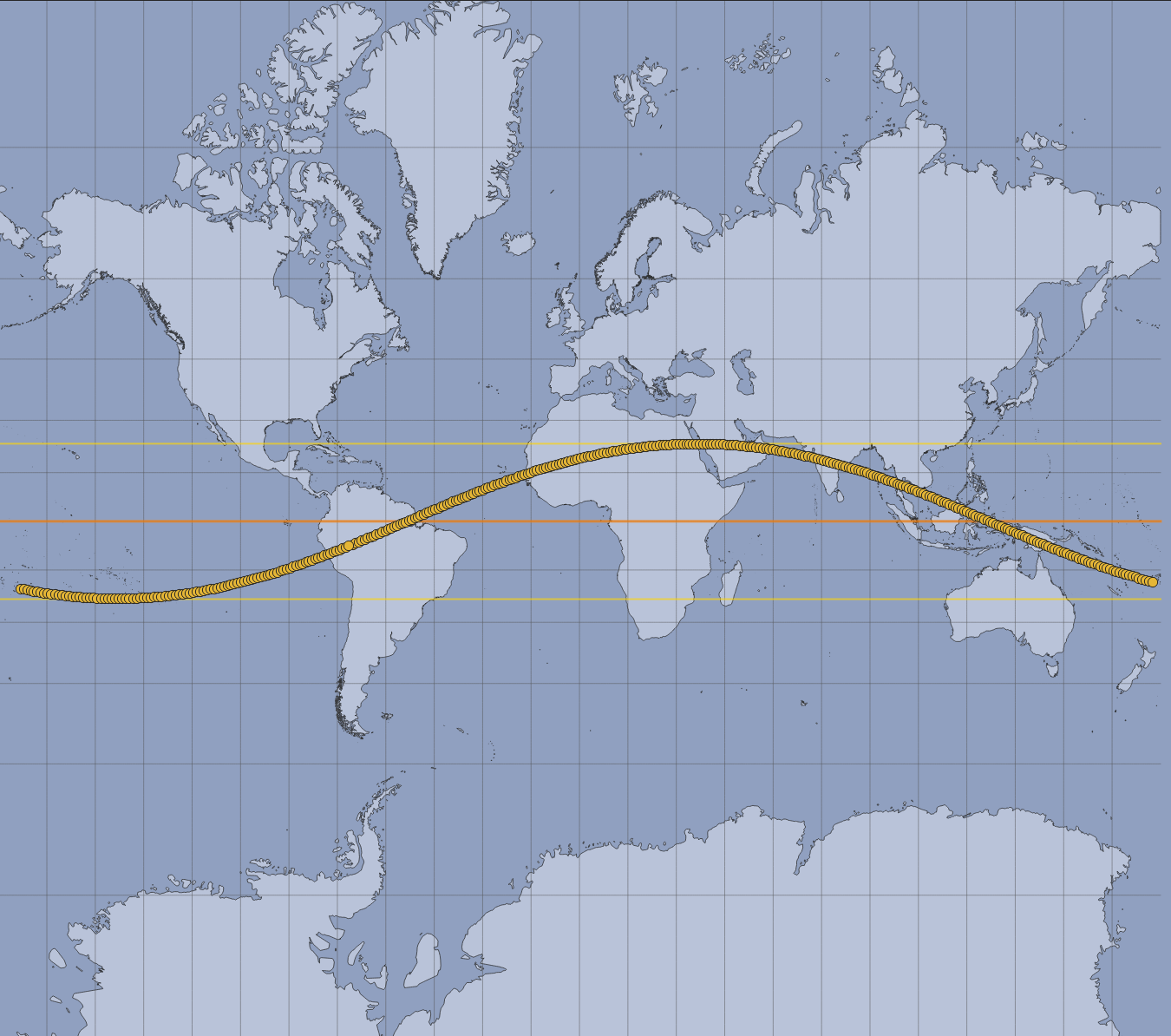
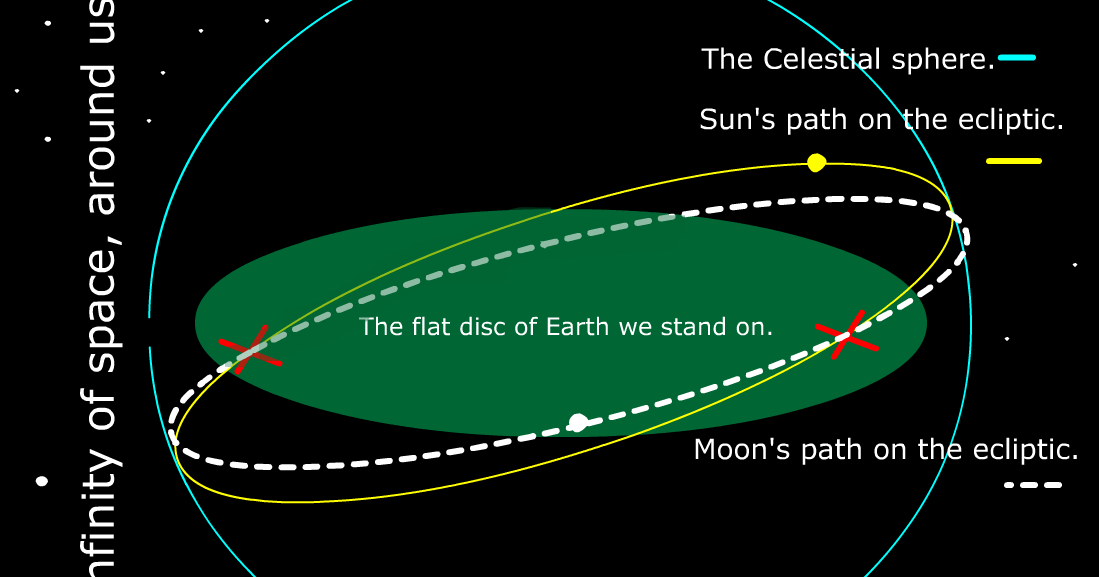
Basic Path of sun and Moon in reality:
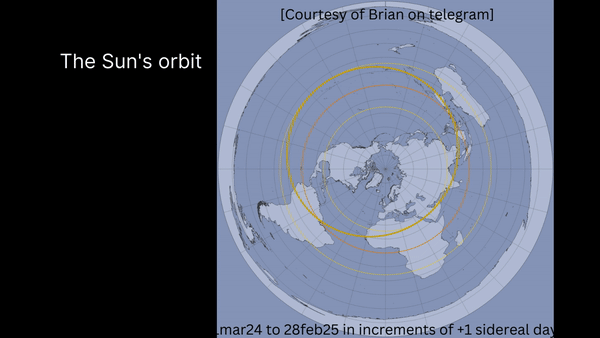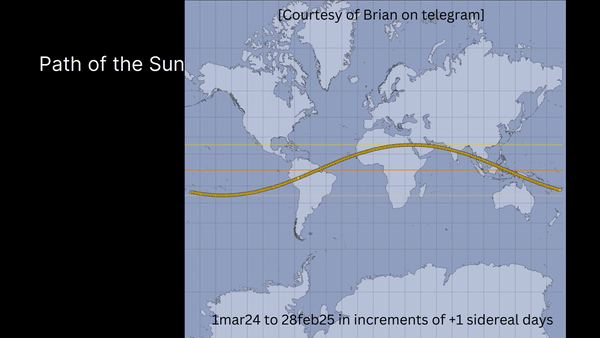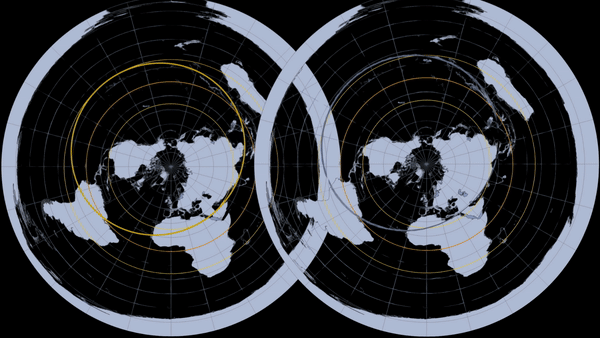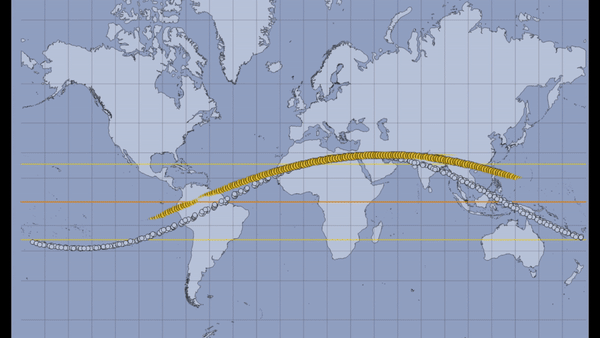
Path of the Sun
.png)
Path of the moon
Although this represents the path in 2d, it does not do it justice. Here is what it looks like in reality.
#### Ecliptic
The intersection of these planes defines two points or nodes on the celestial sphere. The node where the Moon's path crosses the ecliptic from south to north is the ascending node, while the node where the Moon's path crosses the ecliptic from north to south is the descending node. The draconic month is defined as one revolution of the Moon about its orbit with respect to the ascending node. The mean length of this nodical period is 27.21222 days (27d 05h 05m 36s). However, the actual duration can vary by over 6 h from the mean.
Also, remember the equation of time = a direct result of the suns eccentricity and its not quite whole solar year.
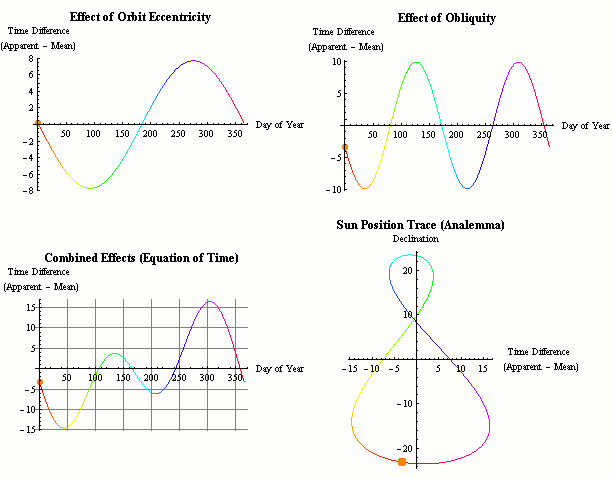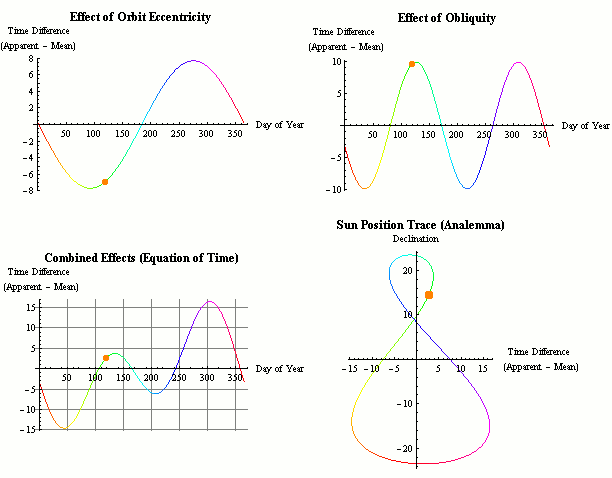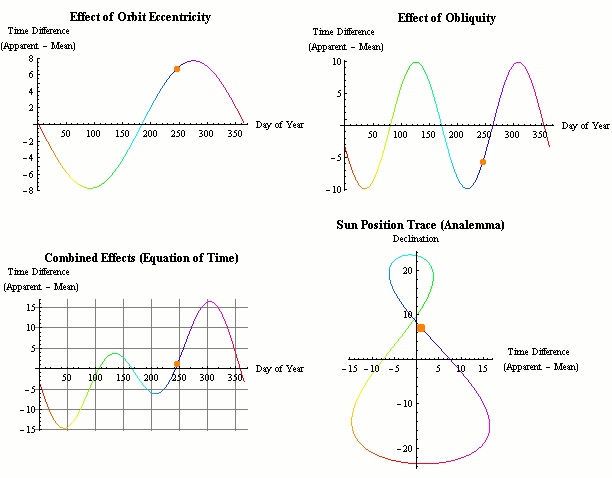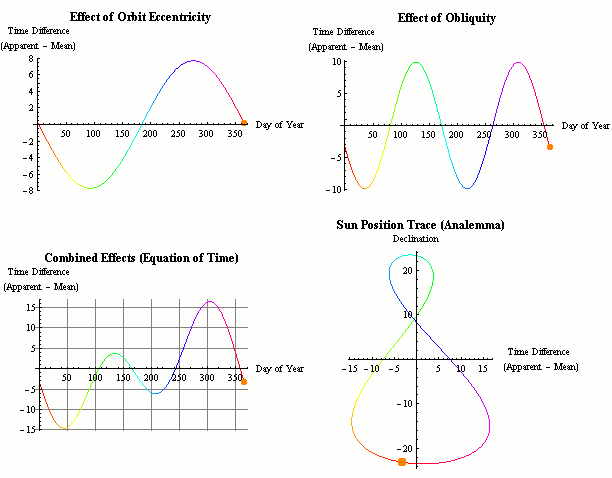
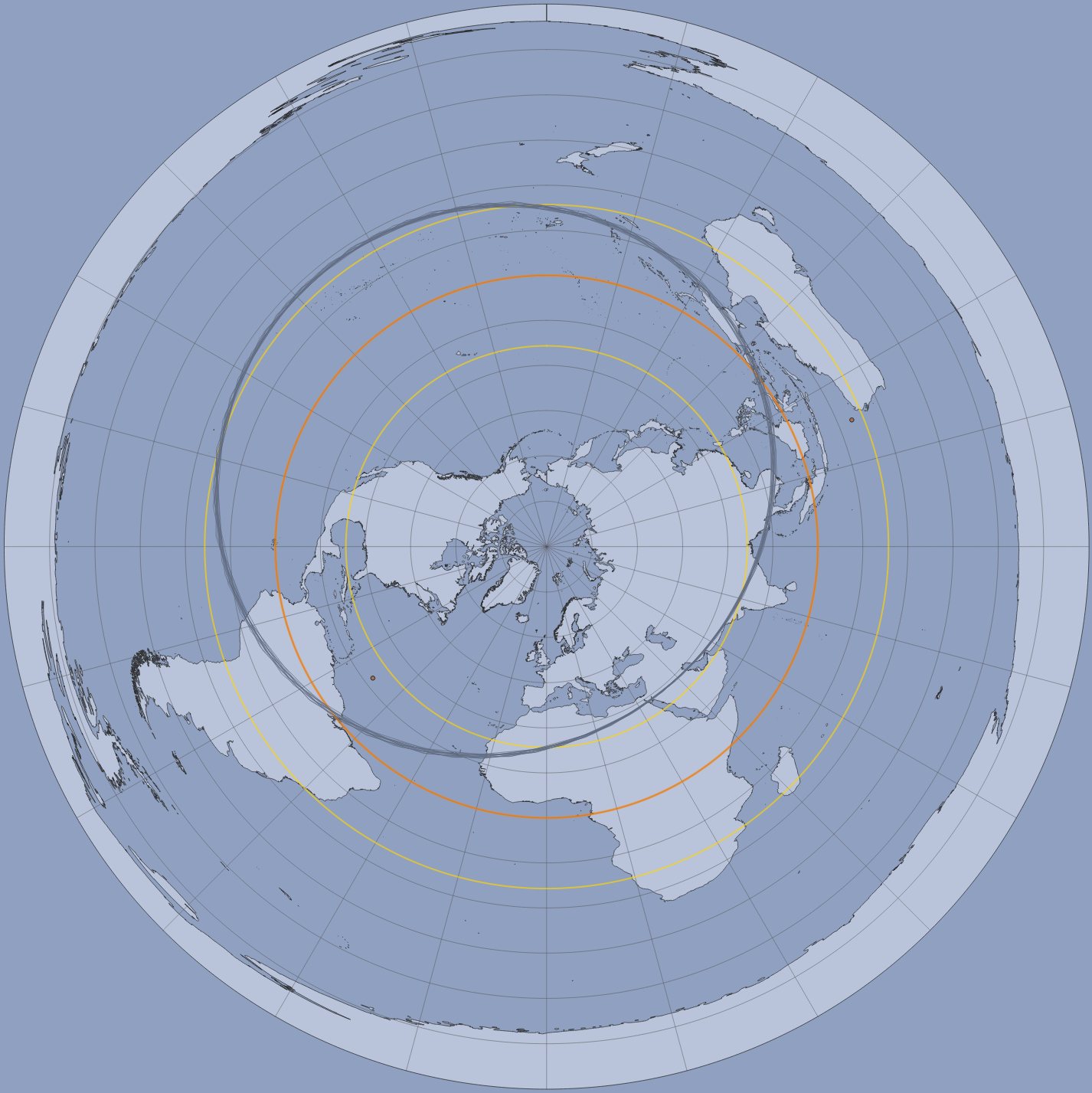
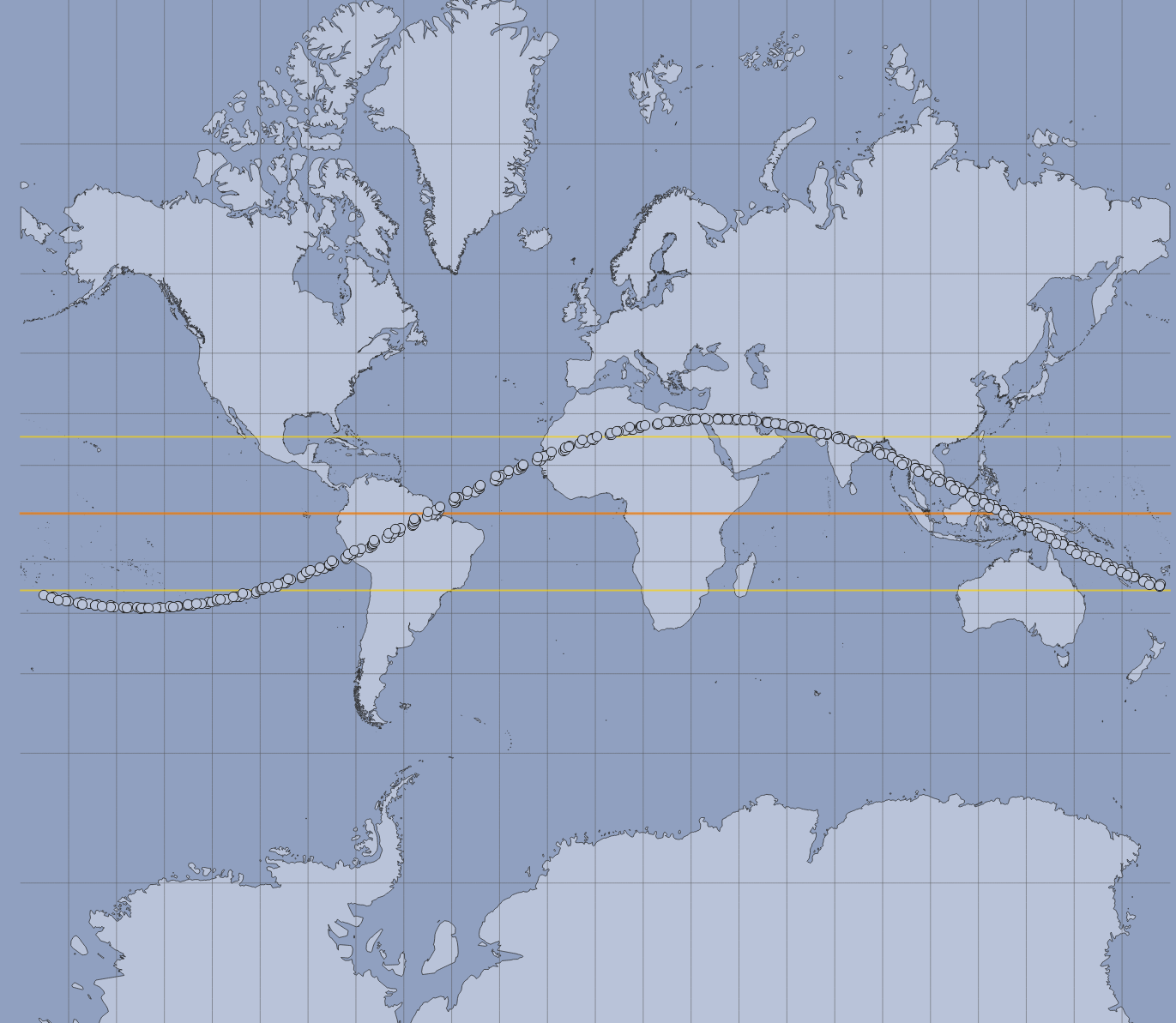
There are many results of the eccentricity of the path of the sun and the moon. Don't forget, the attempts of the mainstream to account for this manifest in "eccentricity of earths orbit" because of their inverted frame of reference, Heliocentric v geocentric.

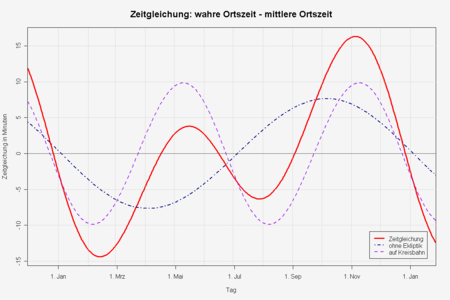
Equation of time (red solid line) and its two main components plotted separately, the part due to the obliquity of the ecliptic (mauve dashed line) and the part due to the Sun's varying apparent speed along the ecliptic due to eccentricity of the Earth's orbit (dark blue dash & dot line)
Think about tides... they call it a frequency because of this:
[Tides - Waves, lunar and Solar Tides, Tidal periods and Amplitudes, Tidal Forces, and Tides in Ocean Basins](https://publish.obsidian.md/shanesql/Tides+-+Waves%2C+lunar+and+Solar+Tides%2C+Tidal+periods+and+Amplitudes%2C+Tidal+Forces%2C+and+Tides+in+Ocean+Basins)
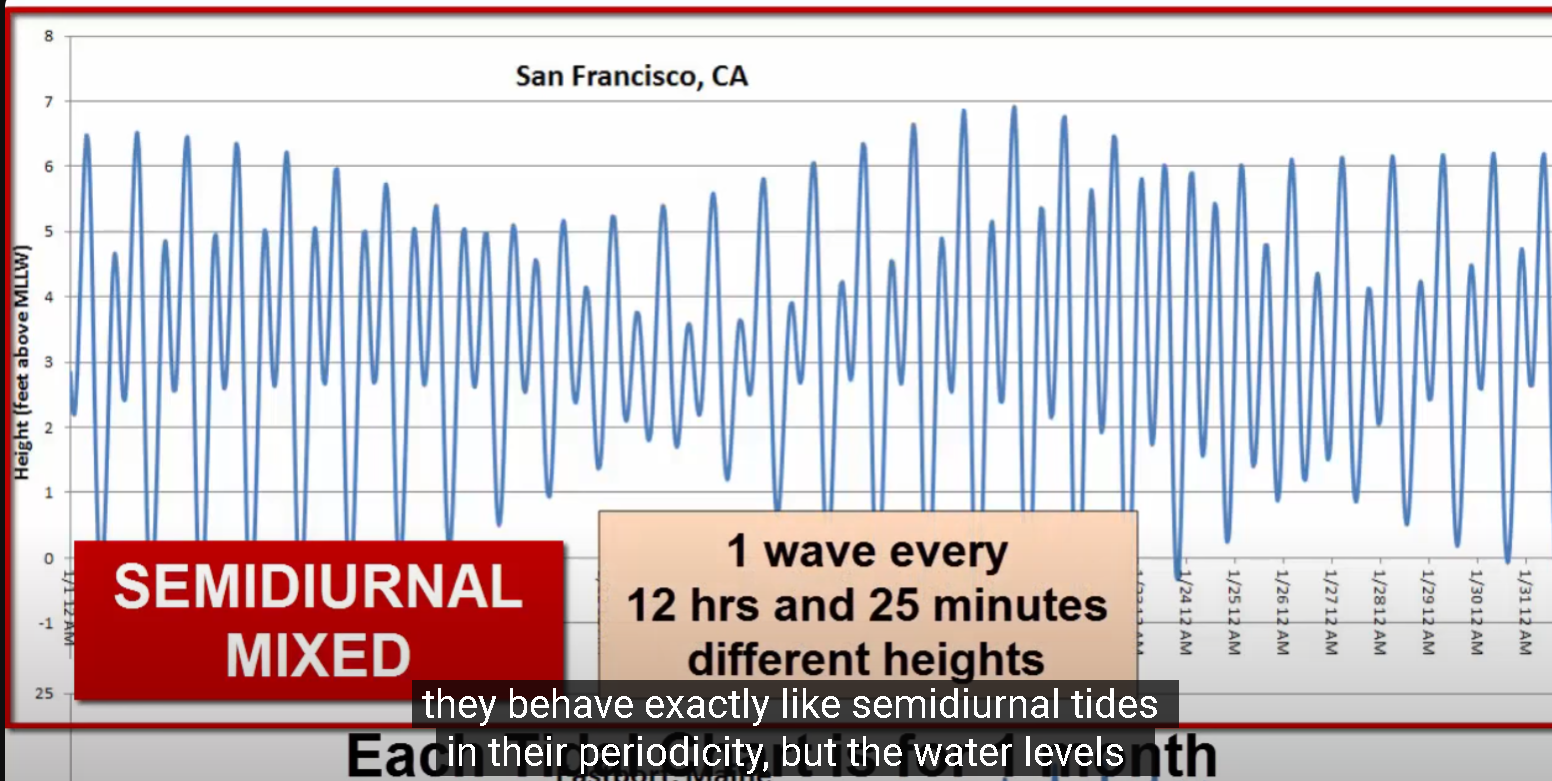
If one has looked into tides and tidal patterns you would be familiar with Harmonic constituents that drive tides and allow us to predict tidal harmonics. [https://marinenavigation.noaa.gov/forecasts.html](https://marinenavigation.noaa.gov/forecasts.html)
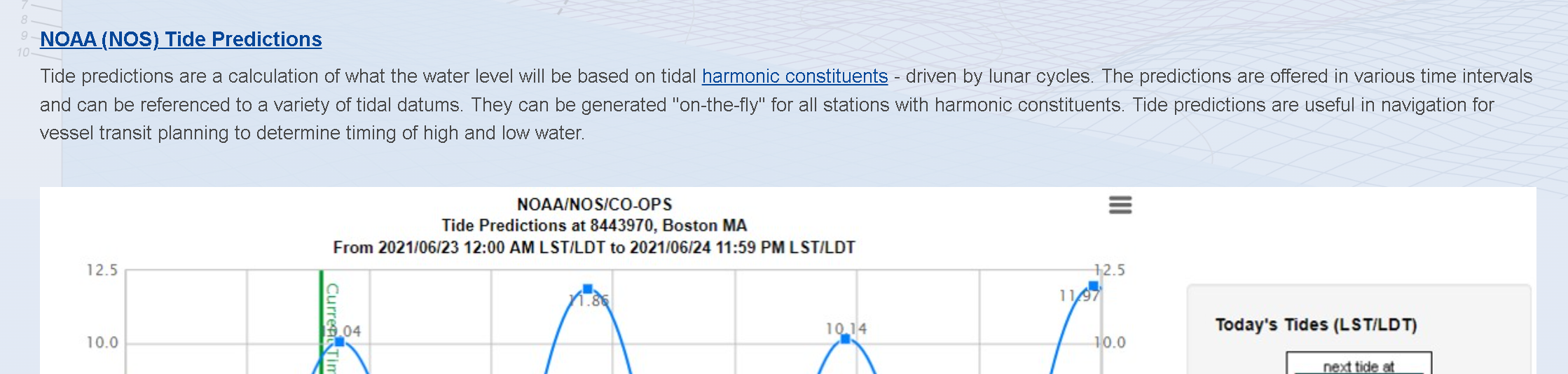

[https://tidesandcurrents.noaa.gov/harmonic.html](https://tidesandcurrents.noaa.gov/harmonic.html)
It just so happens that the same thing that creates all of the above, the eccentricity of the sun, also creates the eclipse cycle. The paths of the sun and the moon are directly responsible for the eclipses.... weather you think they are physical objects, stay in place by gravity, or are projected up there for just you to see, it does not matter. The proximity of these two celestial bodies are what causes eclipses, no matter which way you spin it, the real question is HOW. We won't answer that today, we will be busy tracking the times they intersect and create eclipses. The other very important factor is the phase of the moon. There cannot be a solar eclipse while the moon is NOT in its new moon state. Just like there cannot be a lunar eclipse while the moon is NOT in its full state.
The path of the celestial bodies is important, because the varying speeds at which they travel is the catalyst for almost every pattern we recognize here on earth. The paths of the sun and the moon also have large interactions with the tides on earth, and its for many of the same reasons.
All of this is essentially just a product of the harmonic dance between the sun and the moon.

## The Lunar Cycle
The smallest building block of the greater celestial cycle in the sky.
Which is faster The sun or the moon? The sun appears faster, and appears to lap the moon.
The Sun makes one complete circuit of the ecliptic in 365.24 days, so its average angular velocity is 0.99° per day. At this rate, it takes 34.5 days for the Sun to cross the 34° wide eclipse zone centered on each node. Because the Moon's orbit with respect to the Sun has a mean duration of 29.53 days, there will always be one and possibly two solar eclipses during each 34.5-day interval when the Sun passes through the nodal eclipse zones. These time periods are called eclipse seasons. The mid-point of each eclipse season is separated by 173.3 days which is the mean time for the Sun to travel from one node to the next. The period is a little less that half a calendar year because the lunar nodes slowly regress westward by 19.3° per year.
Duration: The lunar phase cycle (from new moon to new moon) lasts about 29.53 days (a synodic month).
The path of the moon. It takes the moon 29.5 days (29.5 360° rotations of the sun) to make ONE 360° rotation. When the sun has completed 354 360° rotations the moon has comp
Analemma of the Moon
The moon also has an analemma, but it is very different than the sun's version. Because it simply does not exist for a portion of its cycle, that missing portion is very visible. And, it migrates. That moving period of non visibility rotates around the figure 8 perpetually. I just think that is neat.
The nodes of the moon, which revolve around its orbit in a direction opposite to its progress around the Earth, cause these two eclipses of the same character to repeat after an 18-year period, albeit not at the same time of the year due to their progressive motion.
1. The Sun appears to be overtaken by the Moon. This is backwards to the appearance of the 28 day Moon 'cycle' where the Sun leaves the Moon behind and then catches up with it again at the end of the 'cycle'.
2. The Sun appears to be rotating clockwise over time. When seen from the northern 'hemisphere', it should be the opposite.
%20'Sap%20Moon'.jpeg)
![[Pasted image 20250114191628.png]]
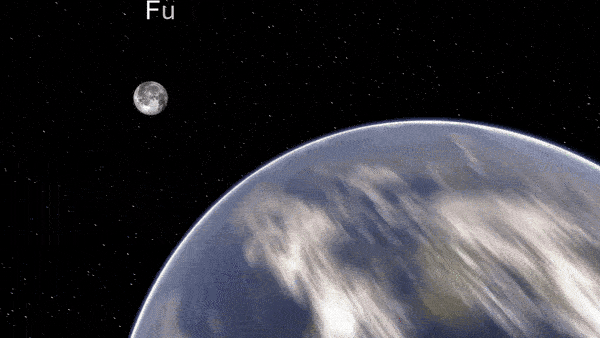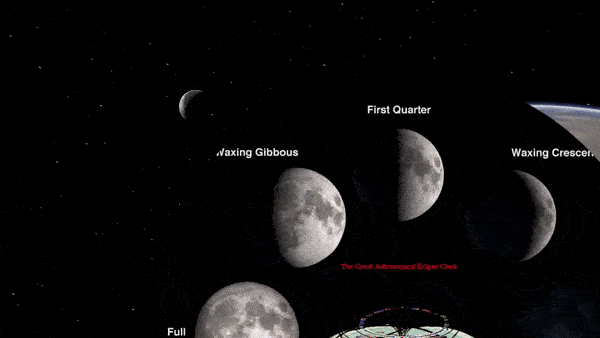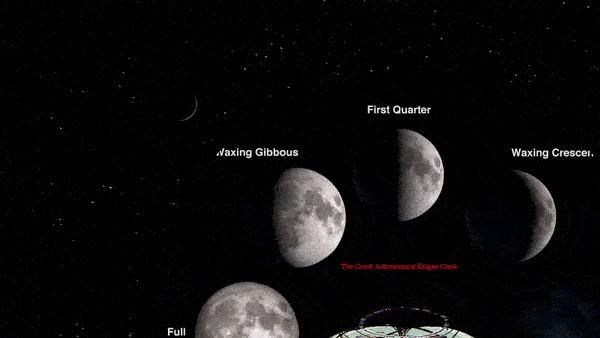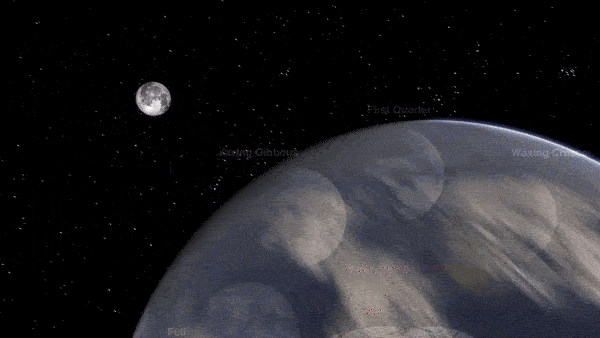
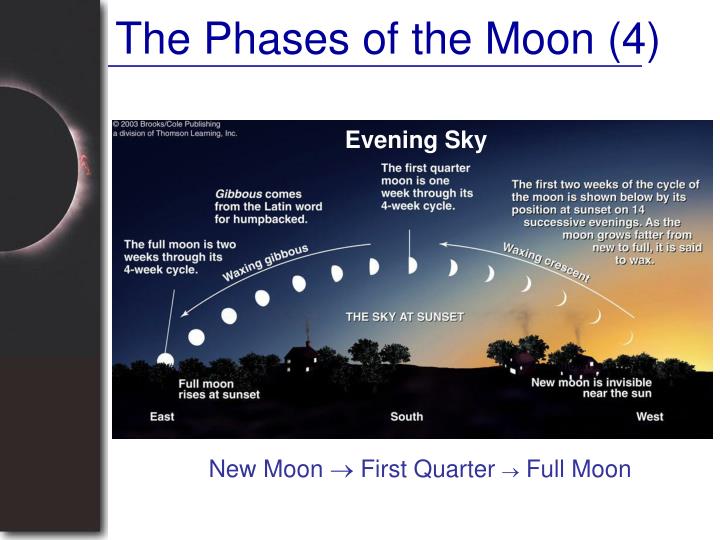
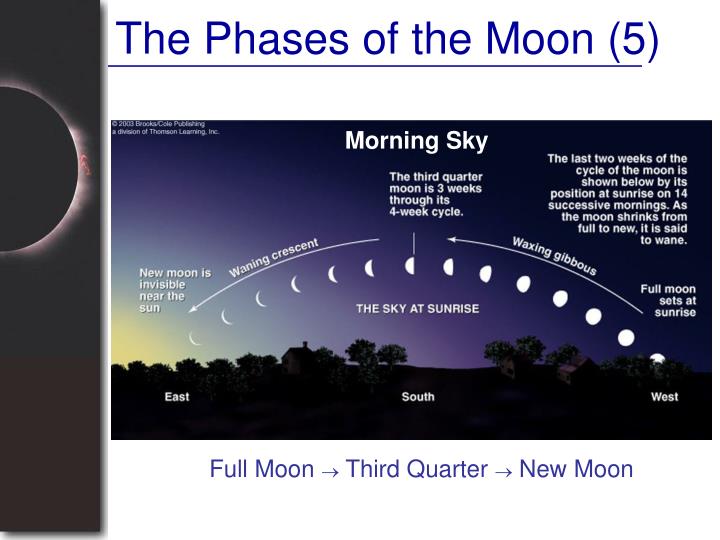
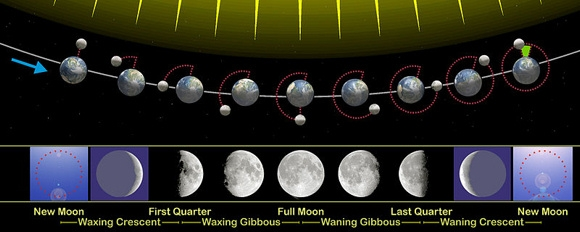
### Phases of the Moon
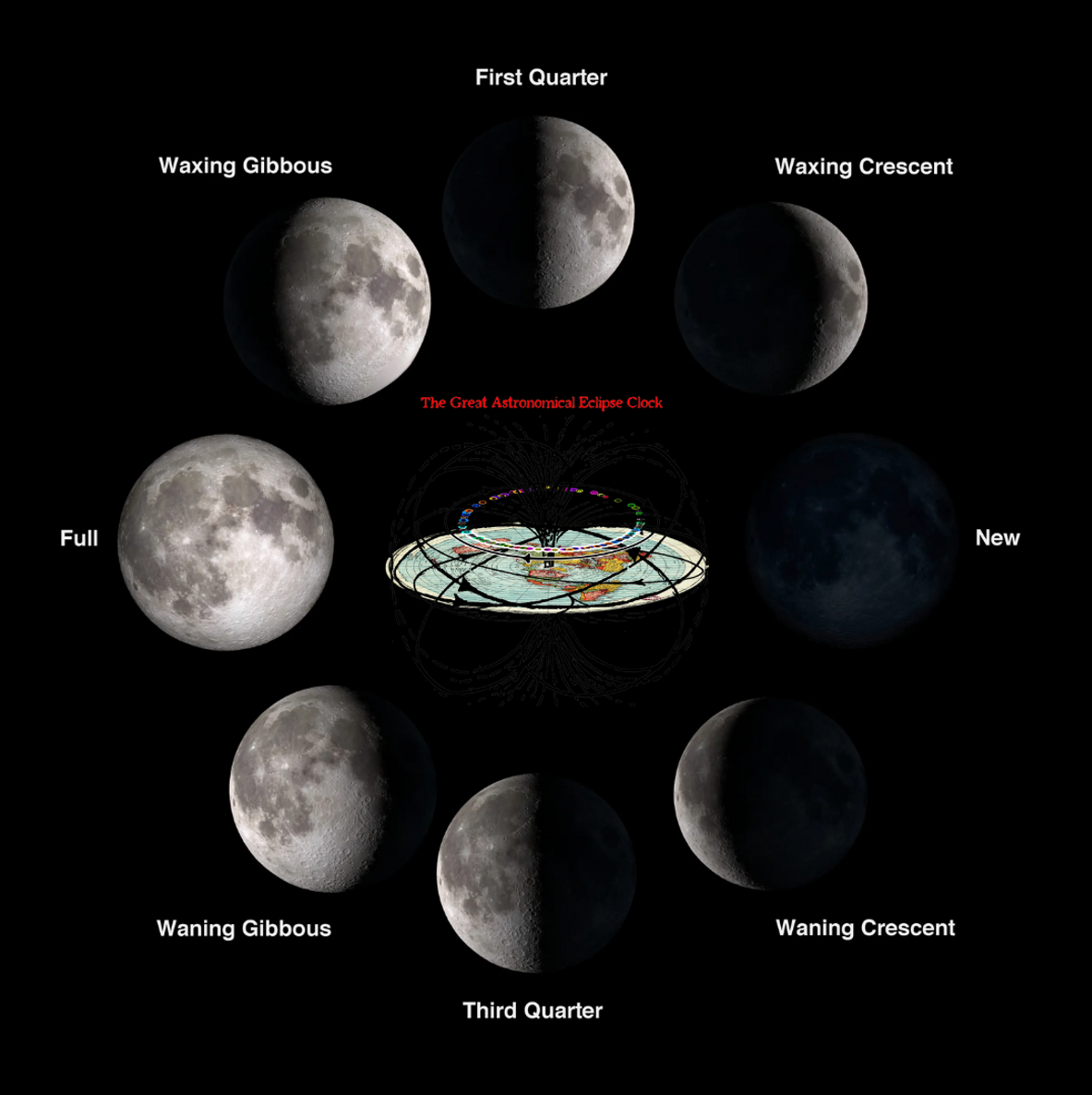
The cycle begins with the new moon, which appears larger until it reaches the full moon phase. Then, the moon appears thinner until the waning crescent disappears and becomes the new moon. Then, the cycle begins again.
1. **New Moon**: The Moon is between the Earth and the Sun, so its only lit by light reflected from Earth.
2. **Waxing Crescent**: The thin crescent thickens.
3. **First Quarter**: Half of the Moon is lit because the Moon is 90 degrees relative to the Sun. This phase is called “first quarter” because the Moon is a quarter of the way through the cycle.
4. **Waxing Gibbous**: The Moon is more than half lit.
5. **Full Moon**: The moon is 180 degrees (in a line with the Earth and Sun) and is fully lit.
6. **Waning Gibbous**: Over half the lunar surface is lit, but less is visible each night.
7. **Third Quarter**: Half the Moon is lit. This is the half that was dark at first quarter.
8. **Waning Crescent**: The sliver of the Moon thins.
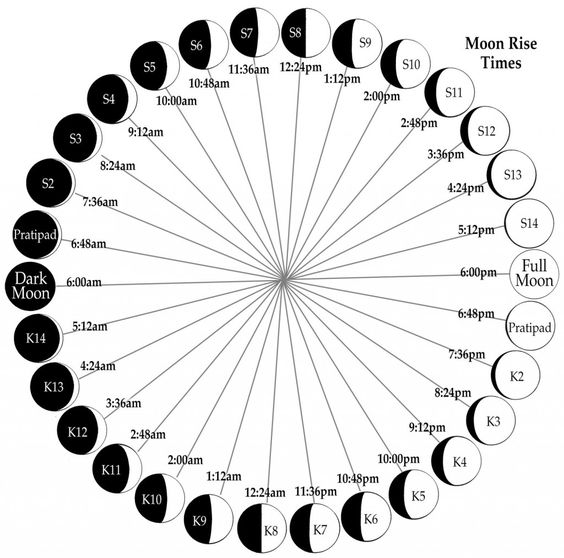
#### Phases of the Moon in the Northern and Southern Hemisphere
No matter where you are on Earth, the phase of the Moon at any given time is the same. The same portion of the Moon is illuminated. So, the phase of the Moon is the same in the Southern Hemisphere as it is in the Northern Hemisphere. However, the orientation of the Moon is different. The Moon appears upside down in the Southern Hemisphere compared to its appearance in the Northern Hemisphere, which means the waxing and waning of the Moon appears to start from a different direction.
[https://www.mreclipse.com/Special/SEprimer.html](https://www.mreclipse.com/Special/SEprimer.html)

# Eclipses
## Types of Eclipses
All photos sourced from Fred: [https://www.mreclipse.com/SEphoto/TSE2001/TSE2001galleryB.html](https://www.mreclipse.com/SEphoto/TSE2001/TSE2001galleryB.html)
### Lunar Eclipses
Heliocentric Explanation
A lunar eclipse occurs when the Earth passes between the Sun and the Moon, causing the Earth's shadow to fall on the Moon. The Moon moves into the Earth's shadow, leading to a dimming or darkening of the lunar surface.
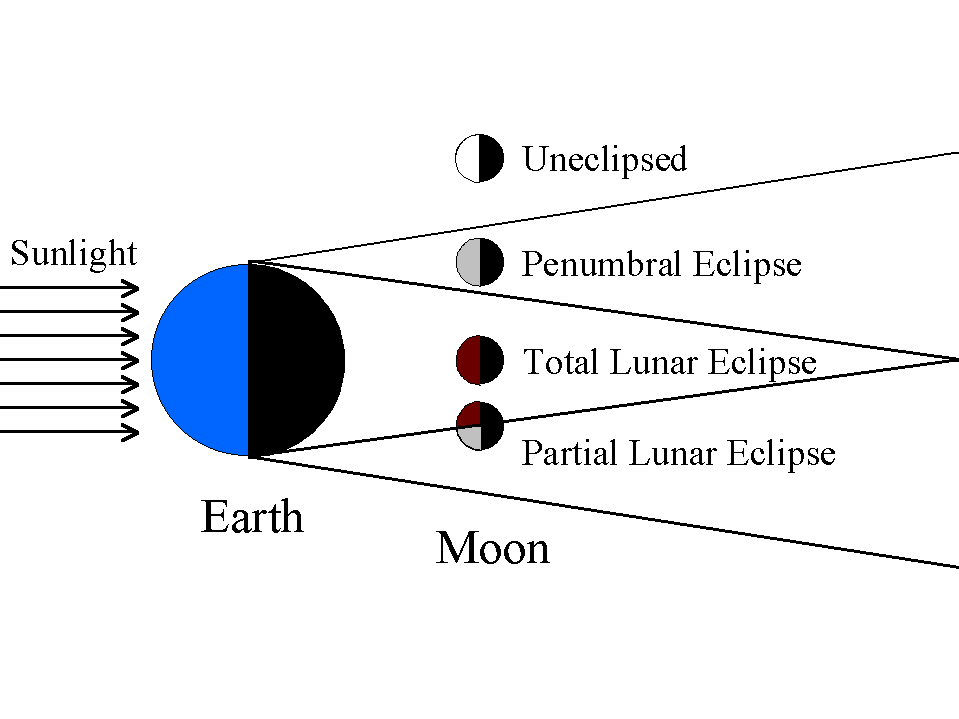
There are three different types of lunar eclipses: total, partial and penumbral



#### Total lunar eclipse
The Moon moves into the inner part of Earth’s shadow, or the umbra. Some of the sunlight passing through Earth’s atmosphere reaches the Moon’s surface, lighting it dimly. Colors with shorter wavelengths ― the blues and violets ― scatter more easily than colors with longer wavelengths, like red and orange. Because these longer wavelengths make it through Earth’s atmosphere, and the shorter wavelengths have scattered away, the Moon appears orangish or reddish during a lunar eclipse. The more dust or clouds in Earth’s atmosphere during the eclipse, the redder the Moon appears

#### Partial lunar eclipse
An imperfect alignment of Sun, Earth and Moon results in the Moon passing through only part of Earth's umbra. The shadow grows and then recedes without ever entirely covering the Moon.

#### Penumbral eclipse
If you don’t know this one is happening, you might miss it. The Moon travels through Earth’s penumbra, or the faint outer part of its shadow. The Moon dims so slightly that it can be difficult to notice.

......Problem?
Why does the 'shadow' switch directions midway through the occultation?
Here it is occulting from the bottom left and finishing from left to right. Did the Moon turn abruptly?
Here are 3 examples:
Super Blood Moon | Lunar Eclipse | Time Lapse in 4K |
Occulted from lower right to top left, finishes from bottom to top.
2018 Total Lunar Eclipse Timelapse | Griffith Observatory
Occulting from the top left to bottom right, but finishes from bottom left to top right.
January 2019 lunar eclipse time lapse. Entire event from start to moonset. 4K resolution
Occults from left to right, finishes from top left to bottom right.
[![ArtUniverse][https://www.youtube.com/watch?v=LK_44AbfH2Q&ab_channel=ArtUniverse]
So if lunar eclipses are caused by a shadow, what is changing direction abruptly immediately after total lunar eclipse is achieved? Question for the people who know everything but have done no investigating it would seem (heliocentrists) ......
**Geocentric Explanation**: _WARNING Your explanation may vary_
A lunar eclipse is when the sun and moon are opposite each other or full moon.
Since the sun and the moon are both luminaries, and the moon is a lesser light, when the moon approaches the sun, it passes right through the connection between the source and the source projection, and this creates the effect known as an eclipse. This causes their light bodies to react with other and creates the red umbra “blood moon” effect. Full moons happen every month but a lunar eclipse occurs when the moon is in its FULL phase and crosses the Sun's ecliptic when the sun is close enough.
### Solar Eclipse
**

##### Total Solar Eclipse

A total solar eclipse happens when the Moon passes between the Sun and Earth, completely blocking the face of the Sun. People located in the center of the Moon’s shadow when it hits Earth will experience a total eclipse. The sky will darken, as if it were dawn or dusk. Weather permitting, people in the path of a total solar eclipse can see the Sun’s corona, the outer atmosphere, which is otherwise usually obscured by the bright face of the Sun. A total solar eclipse is the only type of solar eclipse where viewers can momentarily remove their eclipse glasses (which are not the same as regular sunglasses) for the brief period of time when the Moon is completely blocking the Sun. The next total solar eclipse in the U.S. will be on April 8th 2024. [Personal Eclipse Predictions](https://publish.obsidian.md/shanesql/Personal+Eclipse+Predictions)
Total Solar Eclipse (Timelapse + Soundtrack) 2017 (HD)
[
##### Annular Eclipse (solar):

An annular solar eclipse happens when the Moon passes between the Sun and Earth, but when it is at or near its farthest point from Earth. Because the Moon is farther away from Earth, it appears smaller than the Sun and does not completely cover the Sun. As a result, the Moon appears as a dark disk on top of a larger, bright disk, creating what looks like a ring around the Moon.
Annular Solar Eclipse Time-Lapse May 22, 2012 Ring of Fire!!
[
##### Partial Solar Eclipse
A partial solar eclipse happens when the Moon passes between the Sun and Earth but the Sun, Moon, and Earth are not perfectly lined up. Only a part of the Sun will appear to be covered, giving it a crescent shape. During a total or annular solar eclipse, people outside the area covered by the Moon’s inner shadow see a partial solar eclipse.
Time-lapse of the partial solar eclipse 2017
#### Hybrid Solar Eclipse
Because celestial sphere in which the celestials travel is curved, sometimes an eclipse can shift between annular and total as the Moon’s shadow moves across the globe. This is called a hybrid solar eclipse.
Total Solar Eclipse - Time Lapse - Casper, WY
![Attachments/Pasted image 20240319015859.png]
(https://publish-01.obsidian.md/access/f65db462c005bb547bb3878805fb0f4d/Attachments/Pasted%20image%2020240319015859.png)

The 8 Phases of a solar eclipse.
PARTIAL SOLAR ECLIPSE - ONE MINUTE TIME LAPSE | OCTOBER 14, 2023 | GRIFFITH OBSERVATORY

Solar Eclipse 2017 Flat Earth 1

Geocentric Explanation
A Solar eclipse is when the sun passes behind the moon, and you cannot see it briefly. This is the one that may be most similar between models. Mythologically, This is where the dragon eating it’s tail, or ouroboros comes from. It’s literally the sun eating the moon as it passes. E**very New moon is a mini eclipse, but when they are lined up perfectly at the nodes ( where they cross pathways so to speak) is when there is a total eclipse.
### Selenelion (not really a type of eclipse, but we will allow it)
[](https://en.wikipedia.org/wiki/File:Lunar_eclipse_at_sunrise_Minneapolis_October_2014.png)
[October 2014 lunar eclipse](https://en.wikipedia.org/wiki/October_2014_lunar_eclipse "October 2014 lunar eclipse") viewed from [Minneapolis](https://en.wikipedia.org/wiki/Minneapolis "Minneapolis") during [sunrise](https://en.wikipedia.org/wiki/Sunrise "Sunrise"). Both the Moon and Sun were visible at that time
A _selenelion_ or _selenehelion_, also called a _horizontal eclipse_, occurs where and when both the Sun and an eclipsed Moon can be observed at the same time.
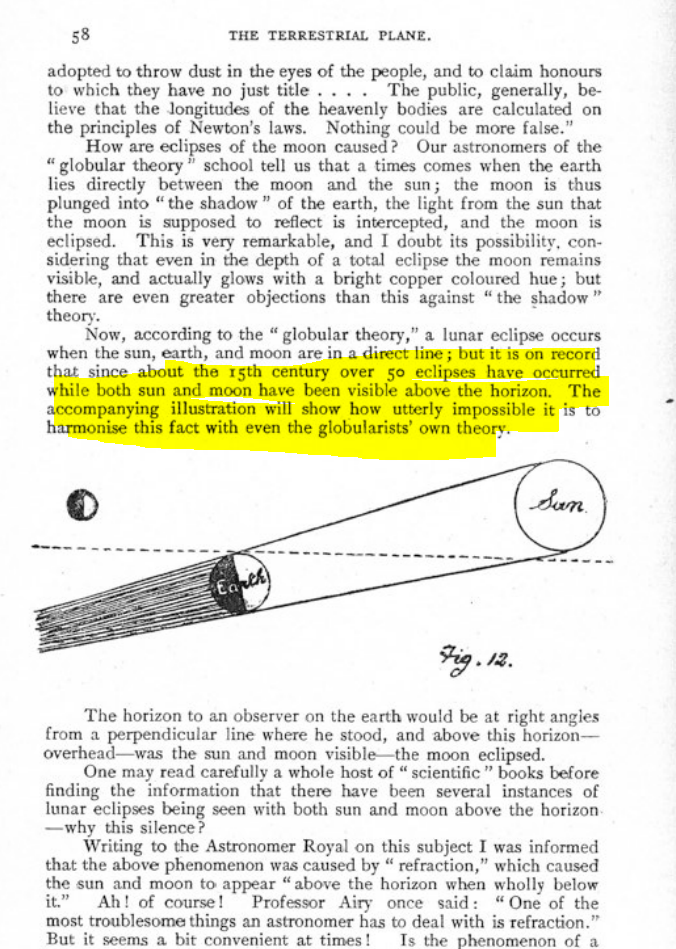
How then, is it possible to have an eclipse where the Sun and Moon are both visible at opposite ends of your horizon?
The mainstream explanation for lunar eclipses is the shadow cast by the earth onto the moon, but....
But this explanation has already been refuted by Frederick Henry Cook. In his book, The Terrestrial Plane, he writes,
"according to the new theory, the false one, a lunar eclipse occurs when the sun and earth and moon are in a straight line**. But it's documented that since the 15th century, more than 50 eclipses have occurred while both the sun and moon were still visible above the horizon**. In other words, the sun and the moon were visible at the same time in the sky while the moon was eclipsed. And this is the kind of thing that just gave me goosebumps. While the moon was eclipsed, for example, on July 19th, 1750 in Paris, observers saw an eclipsed moon while the sun was clearly seen in the sky above the horizon. The same thing happened in the eclipses of April 20th in 1837, September 20th, 1700 June 16th, 1666. November 3rd, 1648. and July 17th of 1590. Also, a recent documented event occurred August 16th of 1989 in Hawaii, where the partially eclipsed moon could be seen rising while the sun was still setting. "
That means the Sun, the Earth, and the Moon were not aligned while the lunar eclipse occurred. So it's impossible that the eclipse was caused by the Earth's shadow being projected onto the Moon. Another thing they say is that the solar eclipse occurs when the Moon stands between the Earth and the Sun.
July 19, 1750,
September 20, 1700
June 16, 1666
November 3, 1648
July 17, 1590
August 16th, 1989.
Paris April 20, 1837
See page 57


Selenellion explained by myself and others
# Lunar Nodes
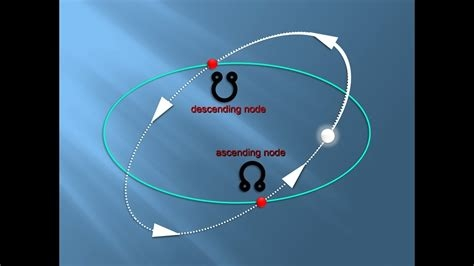
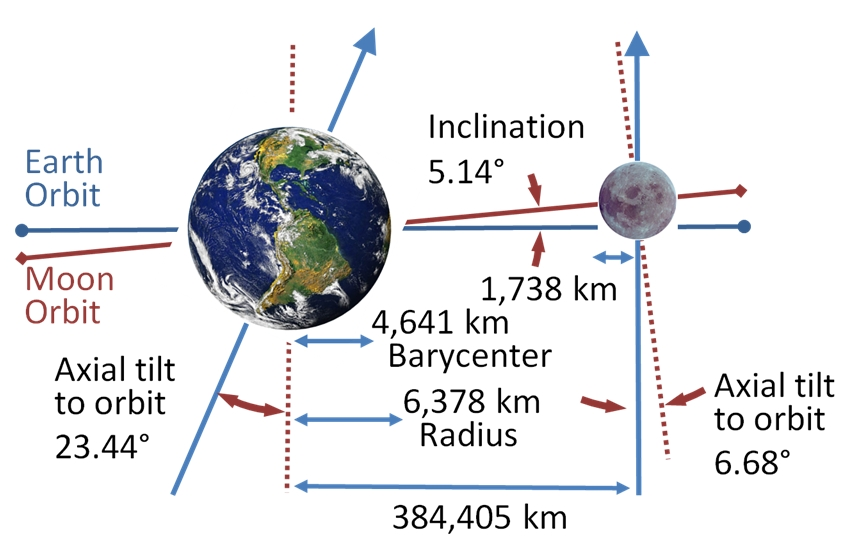
A lunar node is either of the two orbital nodes of the Moon, that is, the two points at which the orbit of the Moon intersects the ecliptic. The ascending (or north) node is where the Moon moves into the northern ecliptic hemisphere, while the descending (or south) node is where the Moon enters the southern ecliptic hemisphere.
Motion The Moon crosses the same node every 27.2122 days, an interval called the draconic month (or draconitic month). The line of nodes, the intersection between the two respective planes, has a retrograde motion: for an observer on Earth, it rotates westward along the ecliptic with a period of 18.6 years or 19.3549° per year. When viewed from the celestial north, the nodes move clockwise around Earth, opposite to Earth's own spin and its revolution around the Sun.
The lunar nodes also process around the ecliptic, completing one revolution (called a draconic period or nodal period) in 18.612958 years (6,798.383 days). (This is not the same duration as a saros.)
.png)

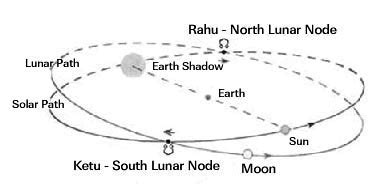
#### Rau and Ketu
Rāhu (Sanskrit: राहु, ☊) is one of the nine major celestial bodies (navagraha) in Hindu texts. Unlike most of the others, Rahu is a shadow entity, one that causes eclipses and is the king of meteors.[1] Rahu represents the ascension of the moon in its precessional orbit around the earth. Rahu is the north lunar node (ascending) and it along with Ketu is a "shadow planet" that causes eclipses. Rahu has no physical shape. It is an imaginary planet but considering the importance of Rahu in astrology, it has been allocated the status of the planet by Rishis
A common myth among the Indian Hindu society is its solar and lunar eclipses are caused by Rahu and Ketu. They are also sometimes referred as the Chhaya graha, meaning shadowy planets. Ancient Hindu scriptures tell us that once Lord Vishnu became angry with the demon Rahu for stealing the celestial liquor and beheaded him with his Sudarshan Chakra. Thereafter, his head started to move across the sky and eventually ended up eating the sun and the moon, causing their eclipse. This story was told to the people in ancient India, as they were not interested in the scientific or the real meaning of Rahu and Ketu. They are nothing but two imaginary astronomical points called the ascending and descending lunar nodes, respectively.
To understand the nodes, let us first understand the ecliptic plane. It is the plane containing the orbit of the Earth around the sun or in a geocentric perspective. Ecliptic plane is the plane containing the orbit of the sun around the Earth. Though both of them means the same thing, just differing in the frame of reference. The moon also revolves around the Earth in 27.3 days, so it must also have a plane containing its own orbit around the Earth. Now these two planes are not the same. The plane of the orbit of moon is tilted by an angle of 5.14 degrees from the ecliptic plane, keeping the center of Earth as their common center. You can imagine the ecliptic as a surface of water kept in a bucket and the plane of moon as a disk, which is half immersed in water at an angle of 5.14 degree from the water surface. Now, the circumference of the disk will intersect the water surface at two points, or in other words, the orbit of the moon intersects the plane of the sun at two points, two opposite points. As during revolution, the moon crosses the ecliptic twice because half of its journey in its orbit is below the ecliptic and in the other half above the ecliptic. As the center of Earth is the common center for both the sun Earth plane and the Earth moon plane. These two points are the lunar nodes. One is called the ascending node, as after crossing it, the moon goes above the ecliptic. This ascending node is called Rahu. The point lying 180 degree opposite to the ascending node is called the descending node. As the moon goes below the ecliptic after crossing this point in its orbit. This point is called K2 in Indian astronomy, the line joining Rahu and Ketu, or the ascending and descending node, is called the line of nodes. Now, Astrodynamics has proven that this line of nodes is not stationary due to precession of the lunar orbit. The line of nodes also revolves around the Earth and completes one revolution in every 18.59, nine 9 or 18.6 years. In other words, the lunar nodes keep sweeping along the orbit of moon and completes one revolution around Earth in 18.6 years. Now we come to the relation of Rahu and Ketu with eclipses. Remember, solar eclipse occurs when the shadow of the moon falls on Earth, and lunar eclipse occurs when the shadow of the Earth falls on the moon as light travels in straight line in space to cast shadow on each other, the sun, moon, and Earth have to form a straight line, or in other words, they have to be in the same plane. But these two heavenly bodies are not in the same plane with respect to Earth, except at the lunar nodes. Now, for eclipses to occur, two conditions have to be satisfied simultaneously one, the Earth, moon, and the sun have to be in a straight line. And number two is that moon must be at one of the nodes. This means that the moon must be present at one of the nodes at the time of full moon or new moon for eclipses to occur. This explains why we don't experience eclipse at every new moon or full moon, because the shadow of the Moon or Earth misses each other, as at that time the moon is either above or below the ecliptic plane.
This also explains why we don't experience eclipse whenever the moon crosses the nodes, because again, the shadow of Earth and Moon will miss each other because they are not aligned in a straight line. Therefore, an eclipse can occur only when the moon is at either of its nodes at the time of New moon or full moon, so that the sun, moon, and Earth are exactly aligned in a straight line from every angle in space.
At the time of New Moon. The moon is between Earth and the sun, and by chance, if it is at one of its nodes, it will cast its shadow on Earth, and people on Earth in that part will not be able to see the sun, or in other words, witness a solar eclipse. Similarly, at the time of full moon, the Earth is between the sun and the moon, and if the moon at that time is present at one of the nodes, Earth will cast its own shadow on the moon, and we on Earth will not be able to see the moonlight, because the moon will not get any light from the sun to shine. In other words, a lunar eclipse would occur. This is the whole story about Rahu and Ketu. They are not at all related to astrology, but are important components of astronomy as they are nothing but the ascending and descending nodes of lunar orbit. Only their myths and stories can be left for astrology.
Okay, now answer one question in the comment section. There is a famous phrase in astronomy that an eclipse never comes alone, as it is always seen that whenever there is a lunar eclipse 14 days later, there is always a solar eclipse in some part of the Earth, and vice versa. What is the reason for this regularity?
[http://www.jgiesen.de/saros/index.html](http://www.jgiesen.de/saros/index.html)
|Period|Solar years|Days|
|---|---|---|
|**Saros**|18.03 years|6,585.3211 days|
|**Nodes draconic**|18.612958 years|6,798.383 days|
|**Nodes sidereal**|18.599525 years||


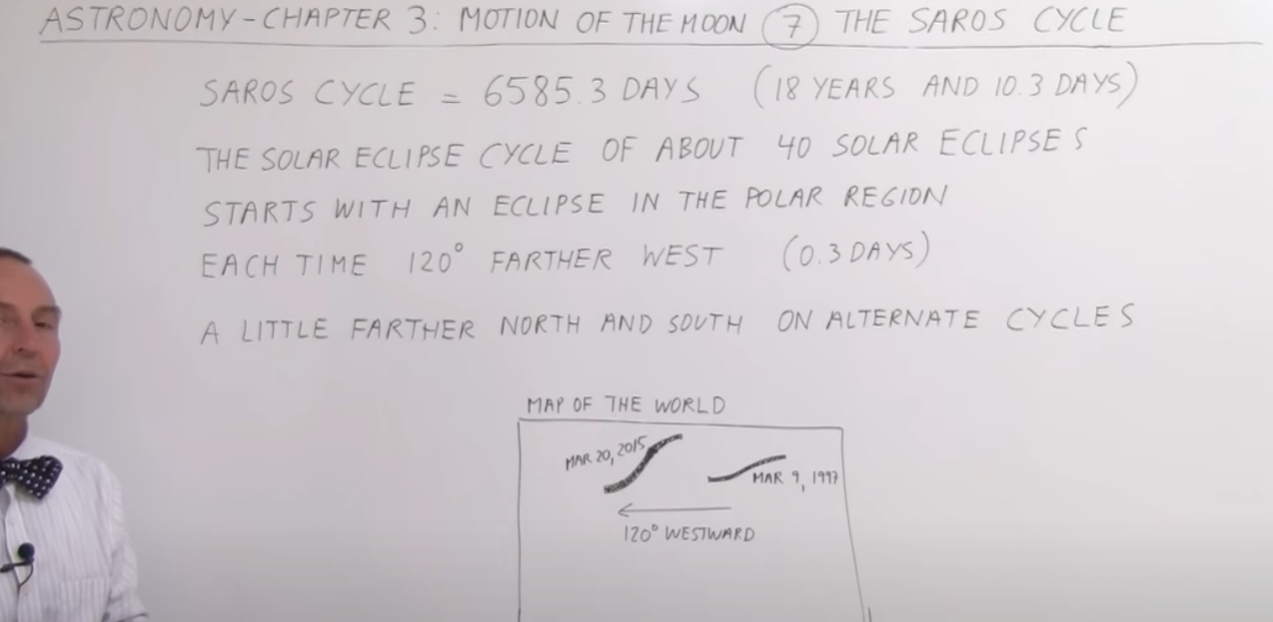
The nodes of the moon, which revolve around its orbit in a direction opposite to its progress around the Earth, cause these two eclipses of the same character to repeat after an 18-year period, albeit not at the same time of the year due to their progressive motion.
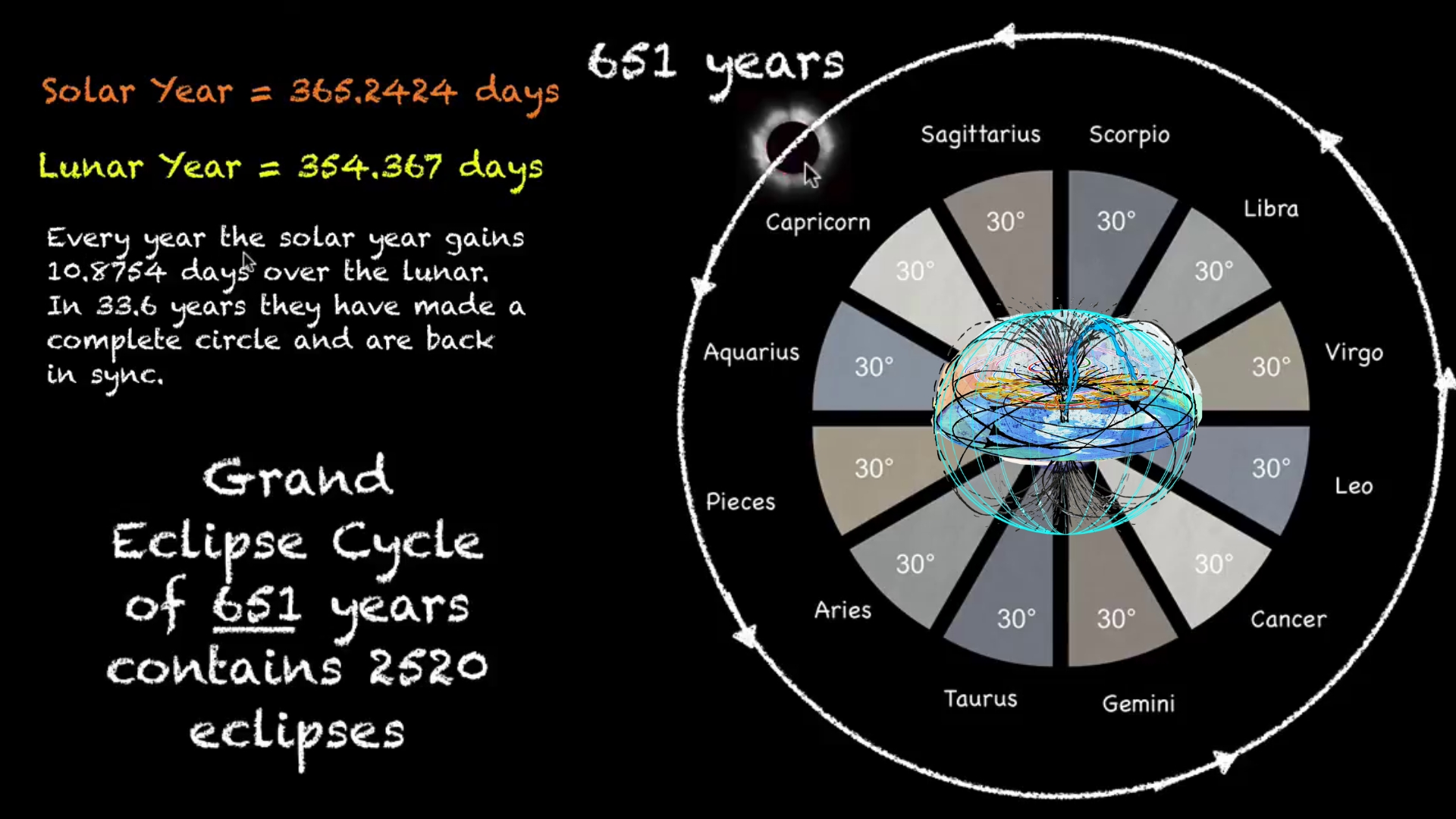
After 651 solar years, these eclipses return to their original dates, demonstrating the moon's completion of its maximum cycle and reaffirming the beginning and date of creation.
##### Astrological interpretation:
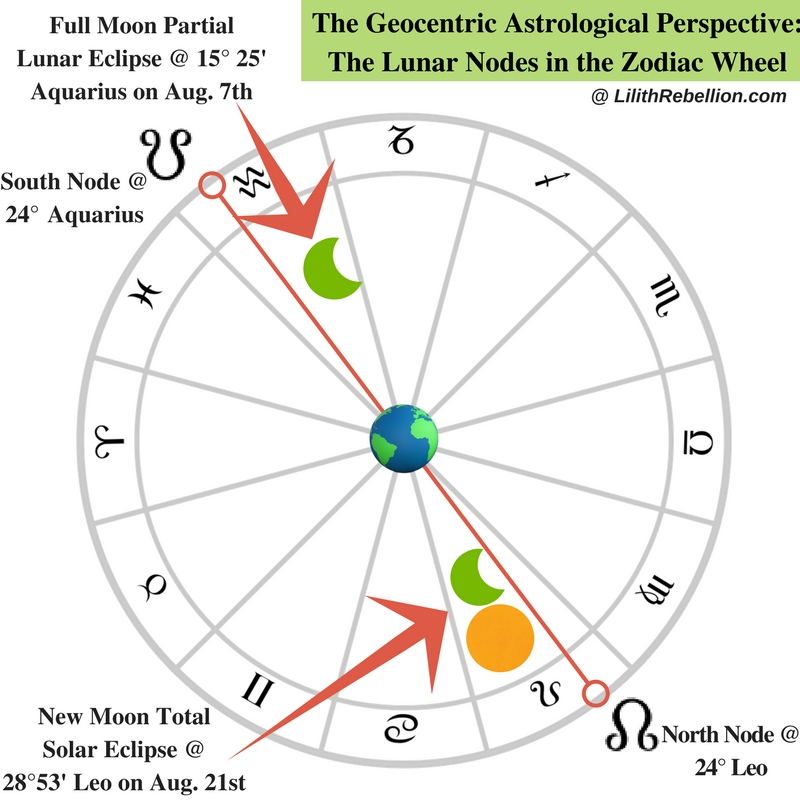
If during its New Moon or Full Moon phase, the Moon happens to be travelling in the vicinity of one of its orbital nodes it is also aligned with the Earth-Sun plane and thus either:
- **obscures the Sun** producing solar eclipse
- **is obscured by the Earth** producing lunar eclipse
Solar eclipses only happen during the New Moon and lunar eclipses only happen during the Full Moon. This may give some associations:
- New Moon’s solar eclipse is a **“super emptiness of spirit”**
- Full Moon’s lunar eclipse is an **“obstruction of life’s flow”**
##### Heliocentric Depiction:
##### Geocentric Equivalent


[https://thu8an.blogspot.com/2013/01/moon-nodes.html](https://thu8an.blogspot.com/2013/01/moon-nodes.html)
The Sun makes one complete circuit of the ecliptic in 365.24 days, so its average angular velocity is 0.99° per day. At this rate, it takes 34.5 days for the Sun to cross the 34° wide eclipse zone centered on each node. Because the Moon's orbit with respect to the Sun has a mean duration of 29.53 days, there will always be one and possibly two solar eclipses during each 34.5-day interval when the Sun passes through the nodal eclipse zones. These time periods are called eclipse seasons. The mid-point of each eclipse season is separated by 173.3 days which is the mean time for the Sun to travel from one node to the next. The period is a little less that half a calendar year because the lunar nodes slowly regress westward by 19.3° per year.
[https://en.wikipedia.org/wiki/Lunar_node](https://en.wikipedia.org/wiki/Lunar_node)
# How do Eclipses Work? An Eclipse Primer 1, 2, 3
[https://www.lilithrebellion.com/2017/08/07/eclipse-primer/](https://www.lilithrebellion.com/2017/08/07/eclipse-primer/)
What determines what type of eclipse? Well mostly, it depends on how close the sun is to the moon when the moon crosses the suns ecliptic. TIMING of the intersection more than any other factor determines the type of eclipse:
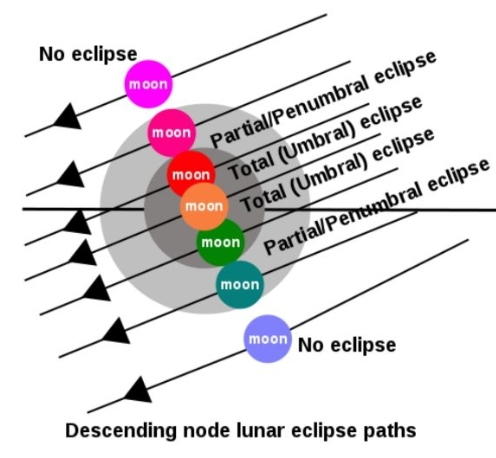
This is just a model pictorially representing how close the two objects are when the moon crosses the suns ecliptic. Dead center will yield a total solar or lunar eclipse, and the further from away from the center you get the less noticeable an occultation will become.
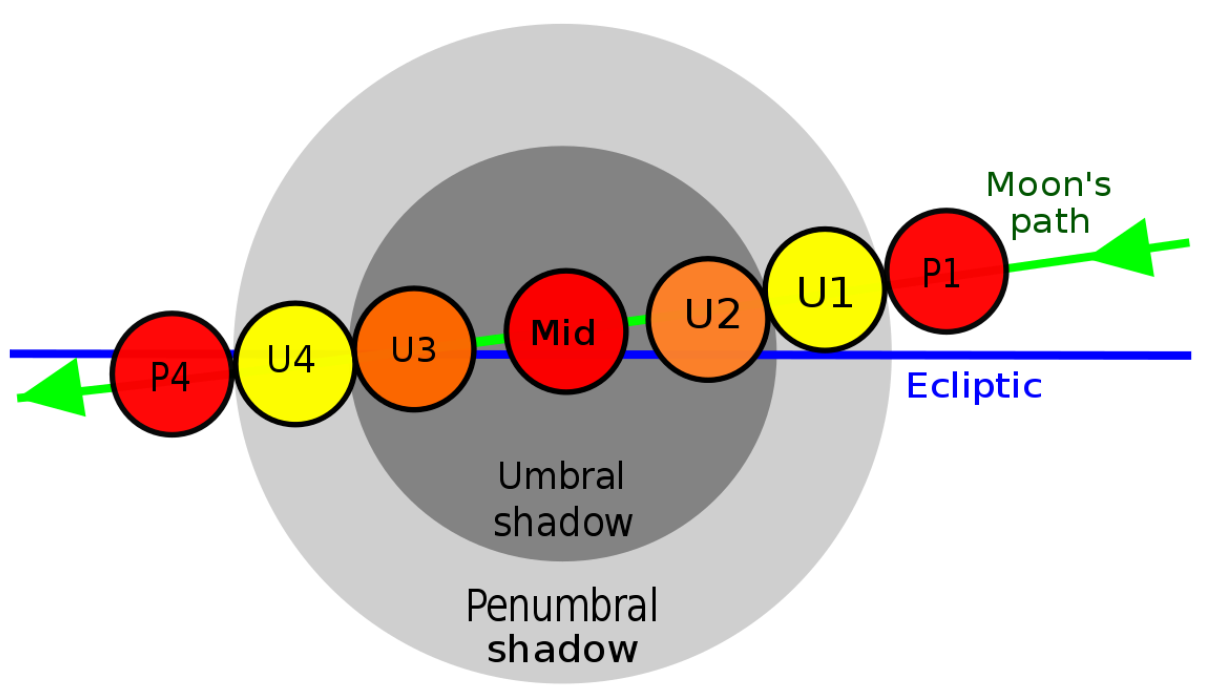
```embed
title: "File:Lunar eclipse contact diagram.svg - Wikimedia Commons"
image: "https://upload.wikimedia.org/wikipedia/commons/thumb/b/b9/Lunar_eclipse_contact_diagram.svg/1200px-Lunar_eclipse_contact_diagram.svg.png"
description: "You cannot overwrite this file."
url: "https://commons.wikimedia.org/wiki/File:Lunar_eclipse_contact_diagram.svg"
```
- _P1_ (_First contact_): Beginning of the penumbral eclipse. Earth's penumbra touches the Moon's outer limb.
- _U1_ (_Second contact_): Beginning of the partial eclipse. Earth's umbra touches the Moon's outer limb.
- _U2_ (_Third contact_): Beginning of the total eclipse. The Moon's surface is entirely within Earth's umbra.
- _Greatest eclipse_: The peak stage of the total eclipse. The Moon is at its closest to the center of Earth's umbra.
- U3 (_Fourth contact_): End of the total eclipse. The Moon's outer limb exits Earth's umbra.
- _U4_ (_Fifth contact_): End of the partial eclipse. Earth's umbra leaves the Moon's surface.
- _P4_ (_Sixth contact_): End of the penumbral eclipse. Earth's penumbra no longer makes contact with the Moon.
```embed
title: "Lunar eclipse - Wikipedia"
image: "https://upload.wikimedia.org/wikipedia/commons/a/a0/Eclipse_lunar_2019.gif"
description: "From Wikipedia, the free encyclopedia"
url: "https://en.wikipedia.org/wiki/Lunar_eclipse"
```


[https://commons.wikimedia.org/wiki/File:Lunar_eclipse_contact_diagram.svg](https://commons.wikimedia.org/wiki/File:Lunar_eclipse_contact_diagram.svg)
## How do they work
- Eclipses can only occur at New Moons (i.e. Solar Eclipses) and Full Moons (i.e. Lunar Eclipses).
- The Moon’s orbit is on _a 5 degree tilt_. If the Moon’s orbit were _not_ tilted, it would perfectly line up with the Sun and _every_ single New Moon and Full Moon would be an eclipse.
- Full Solar and Lunar Eclipses occur when the Moon is in its proper phase and crosses the suns ecliptic.
- During a **New Moon Solar Eclipse** The moon is in its lowest energy phase and invisible, and crosses the ecliptic plane. Only visible during the day.
- During a **Full Moon Lunar Eclipse** The moon is in its highest energy phase, full moon, and crosses the suns ecliptic. Only Visible at night.
- Imagine inserting one hula hoop into another so they are almost lined up, but on a slight angle to each other. There will be two opposing places where the hula hoops will cross. In astro terms one hula hoop is the Sun’s path around the Earth (called the ecliptic), and the other hoola hoop is the Moon’s path around the Earth.
- The two opposing points where the Moon’s path intersects with the Sun’s path are called _**the Lunar Nodes**_. The Nodes are not physical objects, but rather, they are two gradually shifting mathematical points in space that are energetically activated when the Moon, Sun, or other planet intersects with them.
- **THE SUN’S PATH _(the ecliptic)_:**
- The predictable, gradual shift of the Sun’s position through our skies has an exact logic to it due to the fact that the Sun is positioned on a tilted axis while completing a 365-day orbit around the Earth. This creates varying seasons and weather conditions as the Sun moves closer to the Northern axis _(toward the June solstice)_ and then back down toward the Southern axis _(toward the December solstice)_. This “path” is divided into the 12 zodiac signs (Aries – Pisces), through each of which the Sun travels for about 30 days at a time, completing an entire rotation of the zodiac wheel every year.
.gif)
- **THE MOON’s PATH:** The Moon takes about 29 days to complete it’s orbit around the Earth and return to the New Moon position. Thinking back to the hula hoop example where the hula hoops intersect at two points, this means that during each monthly lunation cycle, the Moon’s path of orbit will intersect with the Sun’s path of orbit _twice,_ every single month. This does _**not**_ always produce an eclipse obviously, because an eclipse can only happen during the Full Moon or New Moon phase when the Sun is _also_ near one of the Nodes and a straight line alignment occurs between the Sun, Moon, and Earth. For example, if the Moon passed this point of intersection while at its first quarter phase, it would _not_ be in a straight line alignment with the Sun and the Earth, and so we would _not_ experience an eclipse.
```
- it takes the moon 29.5 days (29.5 360° rotations of the sun) to make ONE 360° rotation. When the sun has completed 354 360° rotations the moon has completed an Analemma of the Moon
```
%201.png)
- The _**Descending Node**_ eclipses go south after crossing, moon travels lower after crossing the suns ecliptic
- The _**Ascending Node**_ eclipses go north after crossing, moon travels higher after crossing the suns ecliptic
- **A New Moon or Full Moon eclipse can occur when the Sun is** _**within about** **18 degrees of either the South Node, or the North Node.**_ The closer the Sun (and therefore by extension, the Moon) is to one of the Lunar Nodes, the more powerful an eclipse will be.
- **There are three types of eclipses: [Total, Annular, and Partial](https://www.timeanddate.com/eclipse/solar-eclipse-types.html).** Total eclipses are the most dramatic and occur when the moon is in the peak apex of its New or Full phase. Partial Eclipses are the weakest type of eclipse and occur when a Full Moon or New Moon alignment is _farther away_ from the South or North node.
- The Nodal Axis, the two points where the Moon’s path intersects with the Sun’s path (think of the two hula hoops touching), is not static. The Nodal Axis predictably shifts into a new set of opposing zodiac signs **every 18 months**. (Astrology)
- Each zodiac sign is 30 degrees, and together the 12 zodiac signs form a 360° wheel (i.e. 12 x 30 degrees).
- Because the Sun takes a year to orbit the Earth, it will cross the Lunar Nodes _twice a year_ – once at the South Node, and again at the North Node about 6 months later.
- Therefore, you’ll hear astrologers refer to these **_two time periods in a year__, as_** _**“Eclipse Seasons”**;_ an astrological contrast to our regular transitions through the seasons of Spring to Winter, etc. The Sun moves through the zodiac ecliptic at about _one degree per day_, and thus an Eclipse Season is said to last **about _36 days_** – the period of time during which the Sun will either be within 18 degrees _approaching_ the Nodes, or within 18 degrees _moving away_ from the Nodes (i.e. 18° + 18°, at 1° per day = 36 days).
- Whatever Full Moons and New Moons occur during “Eclipse Season”, they will arrive as a Solar or Lunar Eclipse. Most of the time, but not always, there will be both a Full Moon eclipse _and_ a New Moon eclipse (occurring as a _pair_ of eclipses, one arriving right after the other), and sometimes there will be up to _three_ eclipses within one Eclipse Season of 36 days.
- The criterion distance range or “orb” allowed for a Full Moon eclipse is _narrower_ than it is for a New Moon Eclipse. For a Full Moon Lunar Eclipse to occur, the Sun and Moon need to be within at least **13°** of the Nodes, rather than within the ~**18°** of distance from the Nodes that is permitted for a New Moon Solar Eclipse to occur.
- _Full Moon Lunar_ Eclipses can be seen anywhere in the world where the Moon is above the horizon at the moment when the eclipse occurs (generally best seen at night), whereas _New Moon Solar Eclipses_ are only visible from particular places in the world.
- In general, eclipses themselves are not rare. **Some form of Solar or Lunar eclipse (total, annular, or partial) will occur [at least twice a year](https://www.astrologyzone.com/eclipse-dates/)**, at least six months apart, during the ~36 days of Eclipse Season when the Sun is within 18 degrees of the Lunar Nodes.
- **Some questions that are asked to determine whether an eclipse is globally significant or not, include:** Is it a Total Eclipse? Total Eclipses are understood to be more powerful than a Partial Eclipse since they are closer to the Nodal Axis. From what countries or states will the eclipse be visible from? In other words, on what countries or parts of the world, is the _**shadow** of the eclipse falling on_? What [Saros Series](https://en.wikipedia.org/wiki/Saros_(astronomy)) does this eclipse belong to and what has happened previously during an eclipse in this same series?
- **The Saros Series is complicated to fully describe here, but briefly:** Each eclipse belongs to a Saros Series _(Saros is a Greek word that means repetition or to be repeated)_. Each Saros Series of eclipses has a **lifespan of about 1300 years**. Each Saros Series is birthed at either the North Pole or the South Pole, and finishes at the opposite Pole after about 1300 years. There are always about 42 Saros Series active at one time. A Saros Series produces a New Moon Solar Eclipse **about every 18 years.** Each Eclipse in a Saros Series will jump forward about 10 degrees along the zodiac wheel when it occurs 18 years after the last Eclipse was produced in that particular Saros Series. Eclipses in any one Saros Series _begin_ as **Partial Eclipses and gradually become more _Total_ as they reach their midlife point at the equator and conjoin the Nodal Axis (when a Saros Series is about 650 years old**). They will then will begin petering out as successive _Partial_ Eclipses near the end of their 1300 year life span toward the North or South Pole. **In total, each Saros Series will produce about 72 Solar Eclipses over the course of its lifespan.**
([https://i0.wp.com/www.lilithrebellion.com/wp-content/uploads/2017/08/The-Geocentric-Astrological-Perspective-The-Lunar-Nodes-in-the-Zodiac-Wheel.jpg?ssl=1](https://i0.wp.com/www.lilithrebellion.com/wp-content/uploads/2017/08/The-Geocentric-Astrological-Perspective-The-Lunar-Nodes-in-the-Zodiac-Wheel.jpg?ssl=1))
# Time & Types of Cycles
In an attempt to break up the NASA jargon, lets take a look at what these different cycles could mean.
Let's go over some terms NASA uses, in my opinion, in an attempt to intentionally confuse the true nature of the eclipse cycle. First, the types of cycles they broke up the lunar and solar cycle into, then the terms of the lunar cycle and lunar phase.
##### Metonic
The Metonic cycle
The Metonic cycle is specifically a period of almost exactly 19 years after which the lunar phases recur at the same time of the year. The recurrence is not perfect, and by pre
The Metonic cycle is a lunisolar cycle. A lunisolar calendar is a calendar in many cultures, incorporating lunar calendars and solar calendars. The date of lunisolar calendars therefore indicates both the Moon phase and the time of the solar year, that is the position of the Sun in the Earth's sky. If the sidereal year (such as in a sidereal solar calendar) is used instead of the solar year, then the calendar will predict the constellation near which the full moon may occur. Cuneiform sources indicate that this cycle was used by Babylonian astronomers (perhaps as early as the 6th century B.C.) for predicting lunar eclipses (Koch, 2001). In the Babylonian and Hebrew lunisolar calendars, the years 3, 6, 8, 11, 14, 17, and 19 are the long (13-month) years of the Metonic cycle. The Babylonians applied the 19-year cycle from the late sixth century BC.
The period of lunations, almost exactly years days (or years days if there are five leap years in the time interval considered), is called the **saros** . as lunations exceed the years days by approximately a third of a day, the regions of the earth affected by the eclipse are shifted at the end of a **saros** , an eclipse does not reproduce in a completely identical way because, the commensurability between the synodic and draconitic revolutions of the moon being only approximated, the relative positions of the sun, the moon and the node have changed. taking into account the minimum and maximum apparent diameters of the astronomers realized that the eclipses repeated at regular intervals. the same eclipse repeats itself every year and day (or if it is a leap year). this period is called the **saros** . thanks to the **saros** , we can find the exact date of an eclipse which took place several centuries before ours.
# Eclipses and the Moon's Orbit
---
## 4.1 Introduction
The Moon revolves around Earth in an elliptical orbit with a mean eccentricity of 0.0549. Thus, the Moon's center-to-center distance from Earth varies with mean values of 363,396 km at perigee to 405,504 km at apogee. The lunar orbital period with respect to the stars (sidereal month) is 27.32166 days (27d 07h 43m 12s). However, there are three other orbital periods or months that are crucial to the understanding and prediction of eclipses. These three cycles and the harmonics between them determine when, where, and how solar and lunar eclipses occur.
The mutual gravitational force between the Sun and Moon is over twice as large as between the Moon and Earth. For this reason, the Sun plays a dominant role in perturbing the Moon's motion. The ever changing distances and relative positions between the Sun, Moon, and Earth, the inclination of the Moon's orbit, the oblateness of Earth, and (to a lesser extent) the gravitational attraction of the other planets all act to throw the Moon's orbital parameters into a constant state of change. Although the Moon's position and velocity can be described by the classic Keplerian orbital elements, such osculating elements are only valid for a single instant in time (Chapront-Touze' and Chapront, 1991). Nevertheless, these instantaneous parameters are of value in understanding the Moon's complex motions particularly with respect to the three major orbital cycles that govern eclipses.
---
## 4.2 Synodic Month
The most familiar lunar cycle is the synodic month because it governs the well-known cycle of the Moon's phases. The Moon has no light of its own but shines by reflected sunlight. As a consequence, the geometry of its orbital position relative to the Sun and Earth determines the Moon's apparent phase.
The mean length of the synodic month is 29.53059 days (29d 12h 44m 03s). This is nearly 2.21 days longer than the sidereal month. As the Moon revolves around Earth, both objects also progress in orbit around the Sun. After completing one revolution with respect to the stars, the Moon must continue a little farther along its orbit to catch up to the same position it started from relative to the Sun and Earth. This explains why the mean synodic month is longer than the sidereal month.
According to astronomical convention, New Moon is defined as the instant when the geocentric ecliptic longitudes of the Sun and Moon are equal. When the synodic month is measured from New Moon to New Moon, it is sometimes referred to as a lunation, and we will follow that usage here. Historically, the phases of the Moon have been used as the basis of lunar calendars by many cultures around the world. The major problem with such calendars is that the year, based on the solar calendar, is not evenly divisible by a whole number of lunations. Consequently, most lunar calendars are actually lunisolar calendars (e.g., Chinese, Hebrew, and Hindu) that include intercalary months to keep the seasons in step with the year.
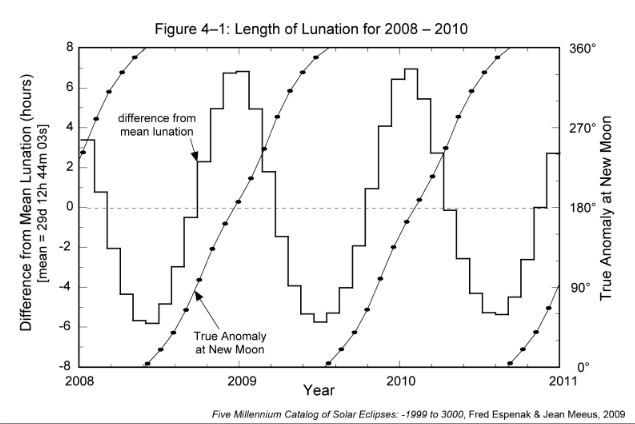
The duration of the lunation actually varies from its mean value by up to seven hours. For instance, Table 4-1 contains details for all lunations in 2008. The first column lists the decimal date of every New Moon throughout the year (Terrestrial Dynamical Time), while the second column gives the duration of each lunation. The third column is the difference between the actual and mean lunation. The first lunation of the year (Jan 08) was 03h 23m longer than the mean. Continuing through 2008, the length of each lunation drops and reaches a minimum of 05h 48m shorter than the mean value (Jun 03). The duration now increases with each succeeding lunation until the maximum value of the year is reached of 06h 49m longer than the mean (Dec 27).
What is the cause of this odd behavior? The last column in Table 4-1 gives a clue; it contains the Moon's true anomaly at the instant of New Moon. The true anomaly is the angle between the Moon's position and the point of perigee along its orbit. In other words, it is the orbital longitude of the Moon with respect to perigee. Table 4-1 shows that when New Moon occurs near perigee (true anomaly = 0°), the length of the lunation is at a minimum (e.g., Jun 03). Similarly, when New Moon occurs near apogee (true anomaly = 180°), the length of the lunation reaches a maximum (e.g., Dec 27).
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-1a.png)
This relationship is quite apparent when viewed graphically. Figure 4-1 plots the difference from mean lunation (histogram) and the Moon's true anomaly (diagonal curves) for every New Moon from 2008 through 2010. The left-hand scale is for the difference from mean lunation, while the right-hand scale is for the true anomaly. The shortest lunations are clearly correlated with New Moon at perigee, while the longest lunations occur at apogee. From the figure, the length of this cycle appears to be about 412 days. The reason why must wait until the next section.
The Moon's orbital period with respect to perigee is the anomalistic month and has a duration of approximately 27.55 days. The lock-step rhythm between the lunation length and true anomaly can be explained with the help of the anomalistic month and Figure 4-2. It illustrates the Moon's orbit around Earth and Earth's orbit around the Sun. The relative sizes and distances of the Sun, Moon, and Earth as well as the eccentricity of the Moon's orbit are all exaggerated for clarity. The major axis of the Moon's orbit marks the positions of perigee and apogee.
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-2a.png)
Two distinct cases-each consisting of two revolutions of the Moon around Earth-are depicted in Figure 4-2. The first case covers the New Moon geometry around perigee. The orbit marked A shows New Moon taking place near perigee at position a1. One anomalistic month later (orbit B), the Moon has returned to the same position relative to perigee (marked b1). However, Earth has traveled about 30° around its orbit so the Sun's direction relative to the Moon's major axis has shifted. The Moon must travel an additional distance of Δb in its orbit before reaching the New Moon phase at b2. This graphically demonstrates why the synodic month is longer (~1.98 days) than the anomalistic month.
The second case takes place about half a year later. New Moon then occurs near apogee (orbit C, position c1). After one anomalistic month, the Moon has returned to the same location with respect to apogee (orbit D, position d1). Once again, Earth has traveled about 30° around its orbit so the Moon must revolve an additional distance of Δd before reaching the New Moon phase at position d2.
An inspection of orbits B and D reveals that the orbital arc Δd is longer that Δb. This means that the Moon must cover a greater orbital distance to reach New Moon near apogee as compared to perigee. Furthermore, the Moon's orbital velocity is slower at apogee so it takes longer to travel a given distance. Thus, the length of the lunation is shorter than average when New Moon occurs near perigee and longer than average when New Moon occurs near apogee. Earth's elliptical orbit around the Sun also factors into the length of the lunation. With an eccentricity of 0.0167, Earth's orbit is about one third as elliptical as the Moon's orbit. Nevertheless, it affects the length of the lunation by producing shorter lunations near aphelion and longer lunations near perihelion.
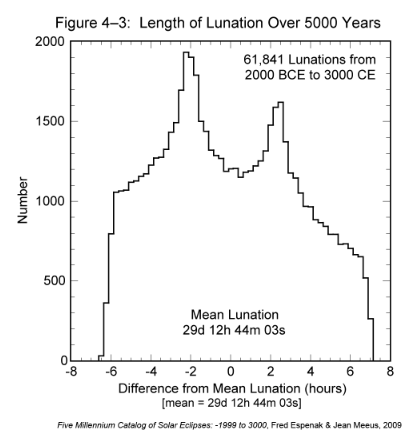
During the 5000-year period covered in this catalog, there are 61841 complete lunations. The shortest lunation began on -1602 Jun 03 and lasted 29.26574 days (29d 06h 22m 40s; 6h 21m 23s shorter than the mean). The longest lunation began on -1868 Nov 27 and lasted 29.84089 days (29d 20h 10m 53s; 7h 26m 50s longer than the mean). Thus, the duration of the lunation varies over a range of 13h 48m 13s during this time interval.
The histogram presented in Figure 4-3 shows the distribution in the length of the lunation over 5000 years. To create the histogram, the durations of individual lunations were binned into 30-minute groups. It might seem reasonable to expect a simple bell-shaped Gaussian curve. However, the results are surprising because the distribution in lunation length has two distinct peaks. This bifurcation can be understood if the lunation length, which depends primarily on the Moon's distance, is considered as a series of sine functions. The extremes of a sine function always occur more frequently than the mean, which is just what is seen in Figure 4-3. For a more detailed discussion, see Meeus (1997).
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-3a.png)
---
## 4.3 Anomalistic Month
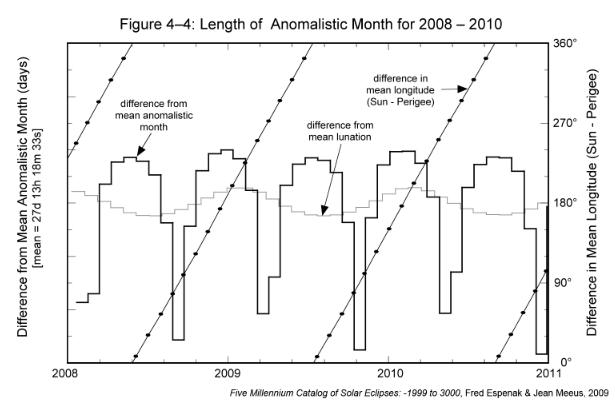
The anomalistic month is defined as the revolution of the Moon around its elliptical orbit as measured from perigee to perigee. The length of this period can vary by several days from its mean value of 27.55455 days (27d 13h 18m 33s). Figure 4-4 plots the difference of the anomalistic month from the mean value for the 3-year interval 2008 through 2010. Also plotted is the difference between the mean longitudes of the Sun and perigee. This is just the angle between the Sun and the Moon's major axis in the direction of perigee. The left-hand scale is the length of the anomalistic month minus the mean value, while the right-hand scale is for the difference in longitude (Sun-perigee). For comparison, the lunation length minus its mean value is also plotted (light gray).
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-4a.png)
The variation in the length of the anomalistic month is much larger than that of the lunation. Figure 4-4 shows the anomalistic month is typically within 1 day of its mean value. But once or twice every 7 to 8 months, the anomalistic month is significantly shorter than the mean by 2 to nearly 3 days. The difference in longitude of the Sun and perigee show that the shortest anomalistic months are correlated with values of 90° and 270°, when the line of apsides is perpendicular to the Sun's direction.
In comparison, the longest anomalistic months take place when the difference in longitude passes through 0° or 180°. The line of apsides is then directed towards or away from the Sun. The maximum duration of the anomalistic month is then about 28.5 days (1.0 day longer than the mean). The Earth-Sun distance also influences the anomalistic month by causing greater extremes near perihelion. This currently occurs in early January each year.
In an earlier discussion on the synodic month, it was assumed that the lunar orbit's line of apsides has a fixed and permanent direction in space. In fact, the length of the mean anomalistic month (27.55 days) exceeds the mean sidereal month (27.32 days) by 0.23 days. Thus, the Moon's major axis slowly shifts with a mean rate of 0.11140° per day in the direct sense, that is, in the same direction as the Moon's orbital motion. This corresponds to an average of 40.7° per year, so it takes 8.85 years (3231.6 days) for the line of apsides to make one complete revolution with respect to the stars.
What impact do the varying length of the anomalistic month and the direct (eastward) rotation of the Moon's elliptical orbit have on the length of the lunation? To answer this, one must first consider Earth's elliptical orbit around the Sun, which has a mean eccentricity of 0.0167. The center-to-center distance between Earth and the Sun varies with mean values of 147,098,074 km at perihelion to 152,097,701 km at aphelion. The direction of Earth's orbital line of apsides also changes but at a rate far slower than the Moon's. Having a direct (eastward) shift with a mean value of 0.0172° per year, it takes about 20,500 years for Earth's major axis to make one complete revolution. This is only 0.0004 of the lunar rate, so it can be treated as fixed for the purpose of the following discussion.
At certain times, the perigee of the lunar orbit and the perihelion of Earth's orbit can have the same ecliptic longitude. Ignoring the 5.1° tilt of the Moon's obit, the major axes are then essentially parallel to each other and point in the same direction. As time passes, the major axis of the lunar orbit slowly rotates east with respect to Earth's major axis until it becomes perpendicular to it 2.21 years later. In another 2.21 years (4.42 years from the start), the major axes of the orbits are again parallel to each other, but the perigee and the perihelion are 180° apart as they point in opposite directions. After an additional period of 2.21 years, the axes are once more perpendicular. Finally, the Moon's perigee and Earth's perihelion again share the same ecliptic longitude after a total interval of 8.85 years.
The length of each lunation minus the mean lunation is plotted in Figure 4-5 for the 20-year period from 2008 through 2027. The periodic rhythm between the lunation length and the true anomaly, as described earlier (via Figure 4-1), can now be seen over the course of two decades. The 412-day mean period of this cycle corresponds to the time between two consecutive alignments of the major axis in the direction of the Sun. It is slightly longer than a year because of the slow eastward shift of the Moon's major axis.
[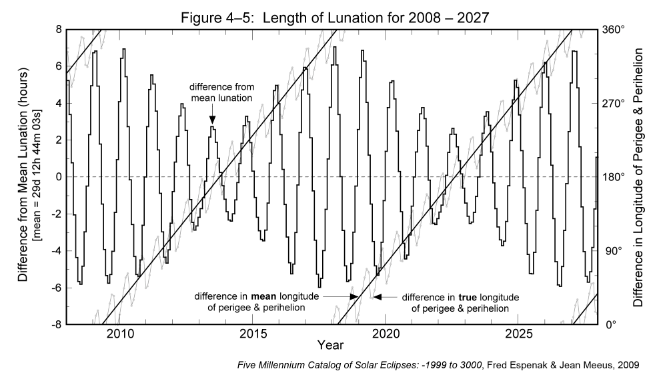
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-5a.png)
An interesting feature revealed in Figure 4-5 is how the extremes in the lunation length slowly vary over a period of nearly 9 years. The envelope defined by the minima and maxima appears to oscillate over a range of values from ±2 h to ±6 h. This behavior is evidence revealing the influence of the 8.85-year cycle in the alignment of the major axes of the orbits of the Moon and Earth.
The amplitude of the envelope is due to the eccentricity of Earth's orbit. When Earth is at perihelion, its orbital velocity is at its maximum value so Earth travels a larger distance around its orbit in a given time as compared to aphelion. Thus, the Moon must travel a greater distance to align with the Sun, which results in a longer lunation. Near aphelion, the opposite conditions produce a shorter lunation.
Using the axis scale on the right, the diagonal lines in Figure 4-5 plot the angle between the Moon's perigee and Earth's perihelion. This is the difference between the Moon's mean longitude of perigee and Earth's true longitude of perihelion. When the angle between the perigee and perihelion is 0°, the length of the lunation varies from a minimum of 29.273 days (-6.17 hours from mean) to a maximum of 29.820 days (+6.93 hours from mean). Similarly, when the angle between the perigee and perihelion is 180°, the length of the lunation varies from a minimum of 29.452 days (-1.88 hours from mean) to a maximum of 29.628 days (+2.33 hours from mean). To summarize, the greatest extremes in the length of the lunation occur when the longitudes of the Moon's perigee and Earth's perihelion are equal. The smallest extremes in the lunation length occur when their longitudes differ by 180°.
Although the Moon's major axis rotates eastward at a mean rate of 0.1114° per day, the true rate varies considerably. Figure 4-5 illustrates the variation by plotting the difference between the true longitudes of the Moon's perigee and Earth's perihelion. This quasi-sinusoidal oscillation about the difference in the mean longitudes shows peak departures of ±30° from average. Indeed, the Moon's major axis can swing both east and west of its mean value, taking on an actual retrograde shift west during some anomalistic months.
This dynamic behavior is due to the gravitational pull of the Sun on the Moon as it orbits Earth. Consequently, a continuous torque is applied to the lunar orbit in an unsuccessful effort to permanently align the major axis towards the Sun. The annual orbit of the Earth-Moon system around the Sun coupled with the Moon's synodic orbit around Earth mean that the conditions for such a permanent alignment are always changing. The overall effect is to twist and distort the shape and orientation of the Moon's elliptical orbit.
It was stated earlier that the Moon's mean orbital eccentricity is 0.0549, but this too is subject to large changes because of solar perturbations. Figure 4-6 plots the variation in the Moon's orbital eccentricity from 2008 through 2010. The instantaneous eccentricity (light gray curve) oscillates with a period tied to the synodic month and ranges from 0.0266 to 0.0762 over this 3-year interval. Superimposed on the instantaneous eccentricity is the eccentricity at the instant of perigee, which occurs at the beginning of each anomalistic month (heavy black curve). The straight diagonal lines represent the difference between the mean longitudes of the Sun and perigee. In other words, it is the angle between the Moon's perigee-directed major axis and the Sun. Oscillating about this line is the difference between the true longitudes of Sun and perigee. The scale for these angles appears along the right side of Figure 4-6. The extreme range of the Moon's orbital eccentricity at perigee during the 5000 years covered by the catalog is 0.0255 to 0.0775.
[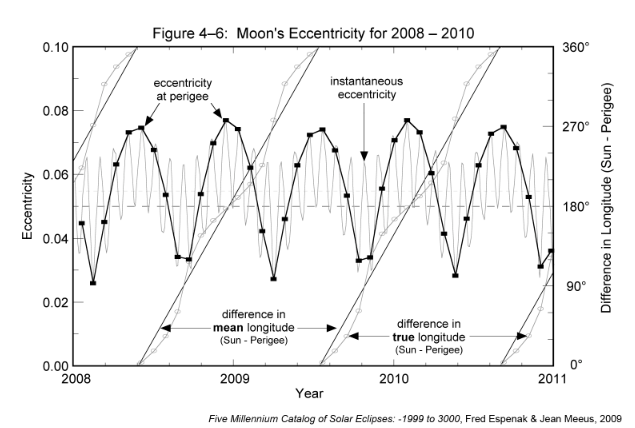
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-6a.png)
Figure 4-6 shows that the eccentricity reaches a maximum when the major axis of the lunar orbit is pointed directly towards or directly away from the Sun (angles of 0° and 180°, respectively). This occurs at a mean interval of 205.9 days, which is somewhat longer than half a year because of the eastward shift of the major axis. The eccentricity reaches a minimum when the major axis of the lunar orbit is perpendicular to the Sun (angles of 90° and 270°).
Such changes in orbital eccentricity produce significant variations in the Moon's distance at perigee and apogee. Figure 4-7 plots the Moon's distance for all perigees and apogees from 2008 through 2010. Also shown is the orbital eccentricity at perigee as well as the angle between the perigee directed major axis and the Sun. The closest perigee (minimum perigee distance) and farthest apogee (maximum apogee distance) occur when the eccentricity is at maximum. This corresponds to times when the Moon's major axis points directly towards or directly away from the Sun (angles of 0° and 180°, respectively). The farthest perigee (maximum perigee distance) and closest apogee (minimum apogee distance) occur when the eccentricity is at minimum. At such times, the major axis is oriented perpendicular to the Sun. During the 3-year interval covered in Figure 4-7, the Moon's perigee distance ranges from 356,568 to 370,216 km while the apogee distance ranges from 404,168 to 406,602 km.
[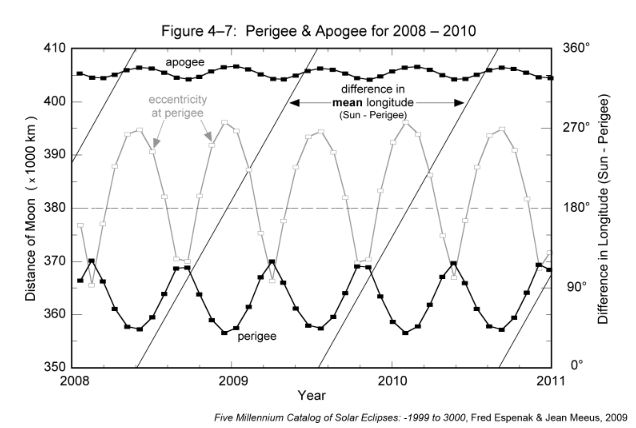
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-7a.png)
Over the 5000-year period of the catalog, there are 66,276 perigees and apogees. During this epoch, the distance of the Moon's perigee varies from 356,355 to 370,399 km while the apogee varies from 404,042 to 406,725 km. The minimum and maximum extremes in orbital eccentricity are 0.0255 to 0.0775 and the extremes in the length of the anomalistic month are 24.629 days (2.925 days shorter than the mean) to 28.565 days (1.011 days longer than the mean). A histogram showing the distribution in the length of the anomalistic month is presented in Figure 4-8 where the durations of individual anomalistic months have been binned into 2-hour groups. The sharply asymmetric distribution shows that anomalistic months longer than the mean cluster over a much shorter range of values compared to anomalistic months shorter than the mean.
[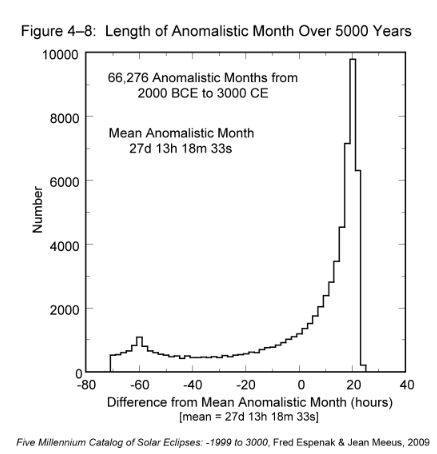
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-8a.png)
---
## 4.4 Draconic Month
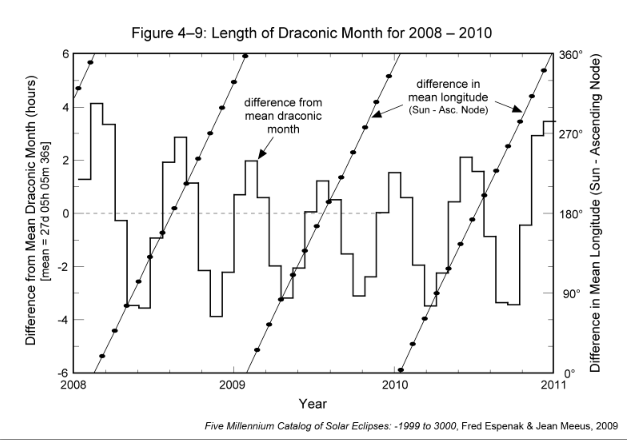
The plane of the Moon's orbit is inclined at a mean angle of 5.145° to the plane of Earth's orbit around the Sun. The intersection of these planes defines two points or nodes on the celestial sphere. The node where the Moon's path crosses the ecliptic from south to north is the ascending node, while the node where the Moon's path crosses the ecliptic from north to south is the descending node. The draconic month is defined as one revolution of the Moon about its orbit with respect to the ascending node. The mean length of this nodical period is 27.21222 days (27d 05h 05m 36s). However, the actual duration can vary by over 6 h from the mean. Figure 4-9 plots the duration of the draconic month minus its mean value for 2008 through 2010. The shortest month over this 3-year period is 27.05115 days (27d 01h 14m), while the longest month is 27.38409 days (27d 09h 13m).
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-9a.png)
The most significant characteristic of this variation is that it is synchronized with the ascending node relative to the Sun's position along the ecliptic. The mean angle between the Sun and the ascending node (i.e., difference in mean longitude) is also plotted in Figure 4-9 (diagonal lines) to illustrate this relationship. The longitude difference at the start of each draconic month is plotted as a black dot. Longitude values can be read using the scale along the right side of the figure. The longest draconic months occur when the difference in the mean longitudes of the Sun and the ascending node is either 0° or 180°. In contrast, the shortest months occur when the angle between the Sun and the ascending node is either 90° or 270°.
The mean draconic month is 0.10944 day (2h 36m 36s) shorter than the sidereal month. Consequently, the lunar nodes slowly rotate west or retrograde (opposite the Moon's orbital motion) along the ecliptic at a rate of 0.05295° per day. One complete rotation of the ascending node about the ecliptic requires 18.6 years (6793.48 days) with respect to the fixed stars.
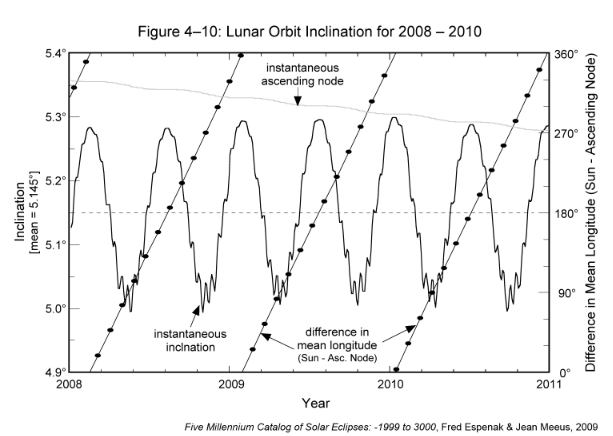
Figure 4-10 plots the instantaneous inclination of the lunar orbit over the 3-year period 2008-2010. The mean angle between the Sun and the ascending node (i.e., difference in mean longitude) is also plotted. The largest inclination of 5.30° occurs when the difference in longitude is either 0° or 180°. In other words, the inclination is always near its maximum value for both solar and lunar eclipses. The smallest inclination of 5.00° occurs when the difference in longitude is either 90° or 270°. Note the small monthly oscillations in the inclination when near its minimum. The figure also plots the longitude of the instantaneous ascending node. Its westward motion draws to a near standstill whenever the Sun aligns with either of the nodes. This corresponds to a difference in longitude of either 0° or 180°.
The mean interval in the periodic variation of both the draconic month and the orbital inclination is 173.3 days. This is the average time it takes for the Sun to travel from one node to the other. It is also equivalent to the interval between the midpoints of two eclipse seasons. The period is slightly less than half a year because of the retrograde motion of the nodes.
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-10a.png)
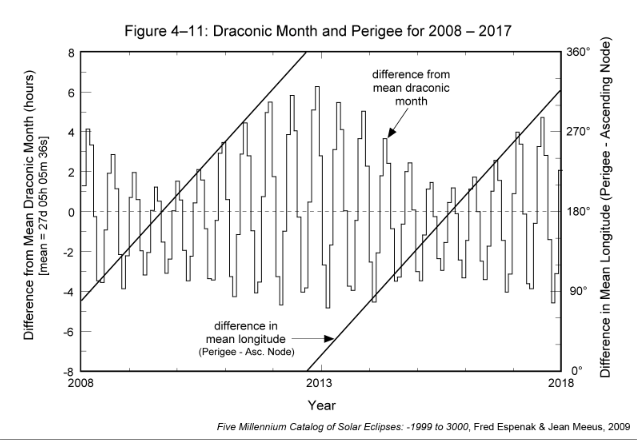
The length of the draconic month is strongly modulated by the position of the nodes with respect to the major axis of the Moon's orbit. The histogram in Figure 4-11 shows how the draconic month changes from 2008 through 2017. The 173-day alignment of the Sun with a node appears as the rapid oscillation in the month length. The quasi-sinusoidal envelopes surrounding the minima and maxima form two longer period oscillations. Over the 10-year period covered in this figure, the minimum month duration varies from 27.089 to 27.011 days (3.0 to 4.8 hours shorter than the mean). The maximum month duration ranges from 27.261 to 27.472 days (1.2 to 6.2 hours longer than the mean).
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-11a.png)
The difference in the mean longitudes of perigee and the ascending node appear as diagonal lines in Figure 4-11. This is the angle between these orbital parameters measured along the ecliptic. The greatest extremes in the draconic month occur when the angle between perigee and the ascending node is 0°. Likewise, the smallest extremes of the month take place when the difference in longitude is 180°. The mean rates of the major axis and the ascending node are 0.11140° east and 0.05295° west per day, respectively. Therefore, the mean period between alignments of the axis and node is 2190.4 days or 6.0 years. This period is clearly seen in Figure 4-11.
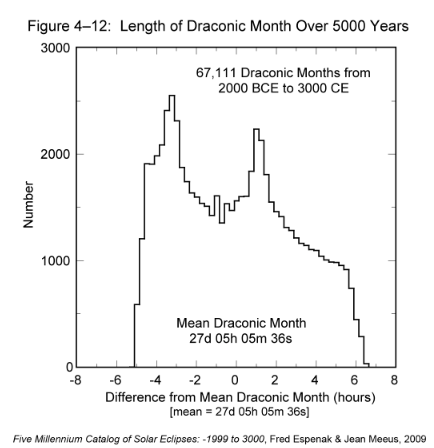
There are 67,111 draconic months during the 5000 years covered in this catalog. The shortest and longest months are 27.004 days (0.208 days or 5.0 hours shorter than the mean) and 27.487 days (0.275 days or 6.6 hours longer than the mean), respectively. A histogram of the distribution in the length of the draconic month over the five millennia appears in Figure 4-12 where the duration of individual draconic months have been binned into 30-min groups. The width and bifurcated symmetry of the distribution resemble the distribution for the lunation (synodic month) in Figure 4-4.
[
Click to enlarge.](https://eclipsewise.com/help/images/Fig4-12a.png)
---

### Lunar Periodicities
[https://eclipsewise.com/solar/SEhelp/SEperiodicity.html](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html)
Name Value (days) Definition
Sidereal month
27.321662
with respect to the distant stars (13.36874634 passes per solar orbit)
Synodic month 29.530589
with respect to the Sun (phases of the Moon,
12.36874634 passes per solar orbit)
Tropical month 27.321582
with respect to the vernal point (precesses in ~26,000 years)
Anomalistic month
27.554550
with respect to the perigee (precesses in 3232.6054 days = 8.850578 years)
Draconic month
27.212221
with respect to the ascending node (precesses in 6793.4765 days = 18.5996 years)
Three periodicities related to lunar orbit, the synodic month, the draconic month, and the anomalistic month coincide almost perfectly each saros cycle. For an eclipse to occur, either the Moon must be on a part of its path in the sky that lines up in front of the Sun (for a solar eclipse) relative to the observer on earth, or must be on a part of its path in the sky that lines up in behind the Sun (for a lunar eclipse). This can happen only when the Moon is new or full, respectively, and repeat occurrences of these lunar phases result from solar and lunar orbits producing the Moon's synodic period of 29.53059 days. During most full and new moons, however, the optical effect we call the shadow of the Earth or Moon falls to the north or south of the other body. Eclipses occur when the sun and moons orbital path crosses at an ascension or descension node. The period of time for two successive lunar passes through the ecliptic plane (returning to the same node) is termed the draconic month, a 27.21222 day period. The geometry of an eclipse, when the new or full moon is near one of the nodes, occurs every five or six months when the Sun is in conjunction or opposition to the Moon and coincidentally also near a node of the Moon's orbit at that time. Two eclipses separated by one saros have very similar appearance and duration due to the orbital paths lining up perfectly and also occurring at a node: this is because the saros is also an integer multiple of the anomalistic month of 27.5545 days, the period of the moon with respect to the lines of apsides in its orbit.
After one saros, the Moon will have completed roughly a whole number amount of synodic, draconic, and anomalistic periods (223, 242, and 239) and the Sun-Moon geometry will be nearly identical: the Moon will have the same phase and be at the same node and the same distance from the Earth. In addition, because the saros is close to 18 years in length (about 11 days longer), the Earth will be nearly the same distance from the Sun, and tilted to it in nearly the same orientation (same season).
However, there are three other orbital periods or months that are crucial to the understanding and prediction of eclipses. These three cycles and the harmonics between them determine when, where, and how solar and lunar eclipses occur. The Saros arises from a natural harmony between three of the Moon's orbital periods: Synodic Month, Anomalistic Month, and Draconic Month.
(period lengths for 2000 CE)
Synodic Month (New Moon to New Moon) = [synodic](https://publish.obsidian.md/shanesql/synodic)
29.530589 days - 29d 12h 44m 03s
Anomalistic Month (perigee to perigee) = The point at which moon is furthest away from earth
27.554550 days - 27d 13h 18m 33s
Draconic Month (node to node) =
27.212221 days - 27d 05h 05m 36s
223 Synodic Months =
6585.3223 days
6585d 07h 43
239 Anomalistic Months =
6585.5375 days
6585d 12h 54m
Draconic Months =
6585.3575 days
6585d 08h 35m
Lunation: When the synodic month is measured from New Moon to New Moon, it is sometimes referred to as a lunation
Types of Cycles within the GAY (Great Astrological Year)
[eclipse cycles](https://en.wikipedia.org/wiki/Eclipse_cycle) of varying lengths can be constructed by combining basic cycles
The [Saros](https://en.wikipedia.org/wiki/Saros_(astronomy)) cycle (18 years 11 days 8 hours) _Just another name for the Lunar Cycle_
the [Inex](https://en.wikipedia.org/wiki/Inex) cycle (29 years minus 20 days) [innex](https://publish.obsidian.md/shanesql/innex)
the [Metonic Cycle](https://en.wikipedia.org/wiki/Metonic_cycle) cycle (19.0 years),
the [Exeligmos](https://en.wikipedia.org/wiki/Exeligmos) cycle (54.1 years),
the [Hipparchus Cycle](https://en.wikipedia.org/wiki/Hipparchic_cycle)cycle (345.0 years)
the Babylonian Period (441.3 years)
An exeligmos is a period of 54 years, 33 days
##### Metonic Cycle
- **Duration**: 19 years.
- **Process of Identification**:
- **Lunar-Solar Reconciliation**: Noticing that 19 solar years (about 6939.6 days) are almost exactly equal to 235 lunar months (about 6939.69 days).
- **Calendar Integration**: Utilizing this cycle to harmonize lunar phases with the solar year in calendars, such as the Hebrew calendar which incorporates 7 leap months in a 19-year cycle.
#### Draconic Month
Moon's path above the earth is inclined at a mean angle of 5.145° to the plane of the suns ecliptic.
#### Synodic Month
The most familiar lunar cycle is the synodic month because it governs the well-known cycle of the Moon's phases. The general geometry of its path above the earth relative to the Sun determines the Moon's apparent phase. The mean length of the synodic month is 29.53059 days (29d 12h 44m 03s). This is nearly 2.21 days longer than the sidereal month. As the Moon revolves around Earth, both objects also progress in orbit around the Sun. After completing one revolution with respect to the stars, the Moon must continue a little farther along its orbit to catch up to the same position it started from relative to the Sun and Earth. This explains why the mean synodic month is longer than the sidereal month. According to astronomical convention, New Moon is defined as the instant when the geocentric ecliptic longitudes of the Sun and Moon are equal. When the synodic month is measured from New Moon to New Moon, it is sometimes referred to as a lunation, and we will follow that usage here. Historically, the phases of the Moon have been used as the basis of lunar calendars by many cultures around the world.
This relationship is quite apparent when viewed graphically. Figure 4-1 plots the difference from mean lunation (histogram) and the Moon's true anomaly (diagonal curves) for every New Moon from 2008 through 2010. The left-hand scale is for the difference from mean lunation, while the right-hand scale is for the true anomaly. The shortest lunations are clearly correlated with New Moon at perigee, while the longest lunations occur at apogee. From the figure, the length of this cycle appears to be about 412 days. - [https://eclipsewise.com/help/moonorbit.html](https://eclipsewise.com/help/moonorbit.html)
#### Anomalistic Month
[https://eclipsewise.com/help/moonorbit.html](https://eclipsewise.com/help/moonorbit.html)
The anomalistic month is defined as the revolution of the Moon around its elliptical orbit as measured from perigee to perigee. The length of this period can vary by several days from its mean value of 27.55455 days (27d 13h 18m 33s). Figure 4-4 plots the difference of the anomalistic month from the mean value for the 3-year interval 2008 through 2010. Also plotted is the difference between the mean longitudes of the Sun and perigee. This is just the angle between the Sun and the Moon's major axis in the direction of perigee. The left-hand scale is the length of the anomalistic month minus the mean value, while the right-hand scale is for the difference in longitude (Sun-perigee). For comparison, the lunation length minus its mean value is also plotted (light gray). - [https://eclipsewise.com/help/moonorbit.html](https://eclipsewise.com/help/moonorbit.html)
The length of each lunation minus the mean lunation is plotted in Figure 4-5 for the 20-year period from 2008 through 2027. The periodic rhythm between the lunation length and the true anomaly, as described earlier (via Figure 4-1), can now be seen over the course of two decades. The 412-day mean period of this cycle corresponds to the time between two consecutive alignments of the major axis in the direction of the Sun. It is slightly longer than a year because of the slow eastward shift of the Moon's major axis.
An interesting feature revealed in Figure 4-5 is how the extremes in the lunation length slowly vary over a period of nearly 9 years. The envelope defined by the minima and maxima appears to oscillate over a range of values from ±2 h to ±6 h. This behavior is evidence revealing the influence of the 8.85-year cycle in the alignment of the Moon along its axis.
Using the axis scale on the right, the diagonal lines in Figure 4-5 plot the angle between the Moon's perigee and Earth's perihelion. This is the difference between the Moon's mean longitude of perigee and Earth's true longitude of perihelion. When the angle between the perigee and perihelion is 0°, the length of the lunation varies from a minimum of 29.273 days (-6.17 hours from mean) to a maximum of 29.820 days (+6.93 hours from mean). Similarly, when the angle between the perigee and perihelion is 180°, the length of the lunation varies from a minimum of 29.452 days (-1.88 hours from mean) to a maximum of 29.628 days (+2.33 hours from mean). To summarize, the greatest extremes in the length of the lunation occur when the longitudes of the Moon's perigee and Earth's perihelion are equal. The smallest extremes in the lunation length occur when their longitudes differ by 180°.
Figure 4-6 shows that the eccentricity reaches a maximum when the major axis of the lunar orbit is pointed directly towards or directly away from the Sun (angles of 0° and 180°, respectively). This occurs at a mean interval of 205.9 days, which is somewhat longer than half a year because of the eastward shift of the major axis. The eccentricity reaches a minimum when the major axis of the lunar orbit is perpendicular to the Sun (angles of 90° and 270°).

[https://eclipsewise.com/help/images/Fig4-8a.png](https://eclipsewise.com/help/images/Fig4-8a.png)
#### Solar Cycle
- **Duration**: About 365.24 days, which is the Earth's orbital period around the Sun.
- **Process of Identification**:
- **Season Observation**: Watching the seasonal shifts and equinoxes/precession to measure a year.
- **Calendar Adjustment**: Incorporating leap years to adjust for the approximately quarter-day discrepancy in the calendar year.
#### Saros Cycle
- **Duration**: Approximately 18 years, 11 days, and 8 hours.
- **Process of Identification**:
- **Eclipse Prediction**: Observing that after one Saros cycle, similar eclipses (solar or lunar) occur with very similar geometries, at roughly the same geographic location.
- **Series Formation**: Identifying a series of these cycles over centuries, as each cycle slightly shifts eastward due to the additional 8 hours.
#### Inex Cycle
- **Duration**: 10,585.32 days, approximately 29 years.
- **Process of Identification**:
- **Eclipse Pattern Tracking**: Observing that eclipses separated by one Inex cycle (10,585.32 days) share similar geometries and occur at the same node with the moon at nearly the same distance from Earth.
- **Series Cataloging**: Cataloging eclipse series based on this cycle to predict future eclipses.
The process of identifying and understanding these cycles involves meticulous observation and recording of astronomical events over time, mathematical calculation to discern patterns and intervals, and the development of calendar systems to synchronize lunar, solar, and eclipse cycles with human timekeeping. These cycles not only help predict celestial events but also have historically been crucial for agricultural planning, religious ceremonies, and calendar reforms
# Great Astrological Year
The Sun makes one complete circuit of the ecliptic in 365.24 days, so its average angular velocity is 0.99° per day. At this rate, it takes 34.5 days for the Sun to cross the 34° wide eclipse zone centered on each node. Because the Moon's orbit with respect to the Sun has a mean duration of 29.53 days, there will always be one and possibly two solar eclipses during each 34.5-day interval when the Sun passes through the nodal eclipse zones. These time periods are called eclipse seasons. The mid-point of each eclipse season is separated by 173.3 days which is the mean time for the Sun to travel from one node to the next. The period is a little less that half a calendar year because the lunar nodes slowly regress westward by 19.3° per year.
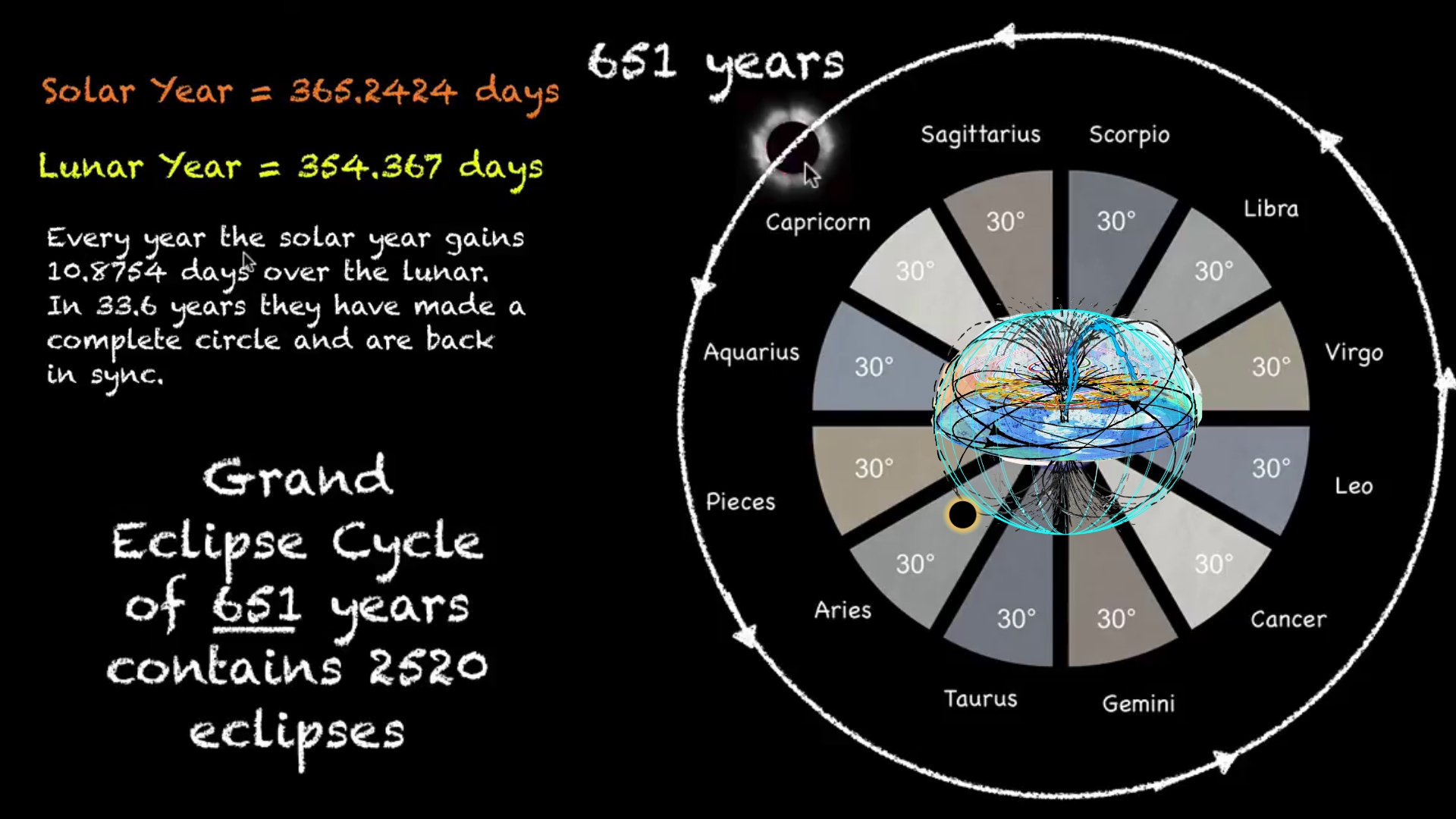
The diagram illustrates the sequence of lunar months before and after the first and second eclipses, totaling six lunar months, which is a consistent pattern between eclipses.
The position of the eclipses marks a significant moment in scientific time, confirming the motion of the moon and the establishment of the seasons beginning in year 0 A.M.
The nodes of the moon, which revolve around its orbit in a direction opposite to its progress around the Earth, cause these two eclipses of the same character to repeat after an 18-year period, albeit not at the same time of the year due to their progressive motion.
After 651 solar years, these eclipses return to their original dates, demonstrating the moon's completion of its maximum cycle and reaffirming the beginning and date of creation.
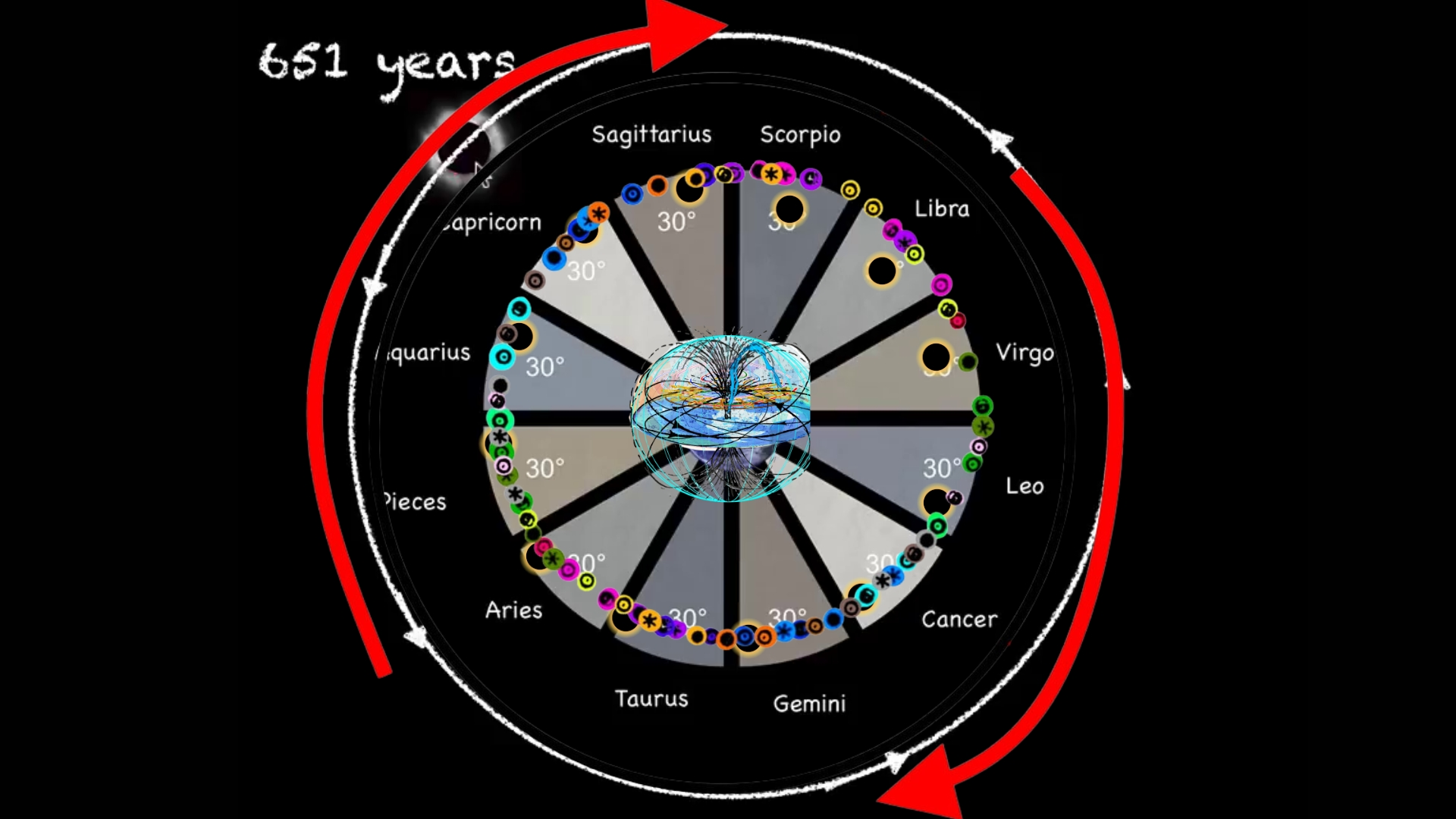
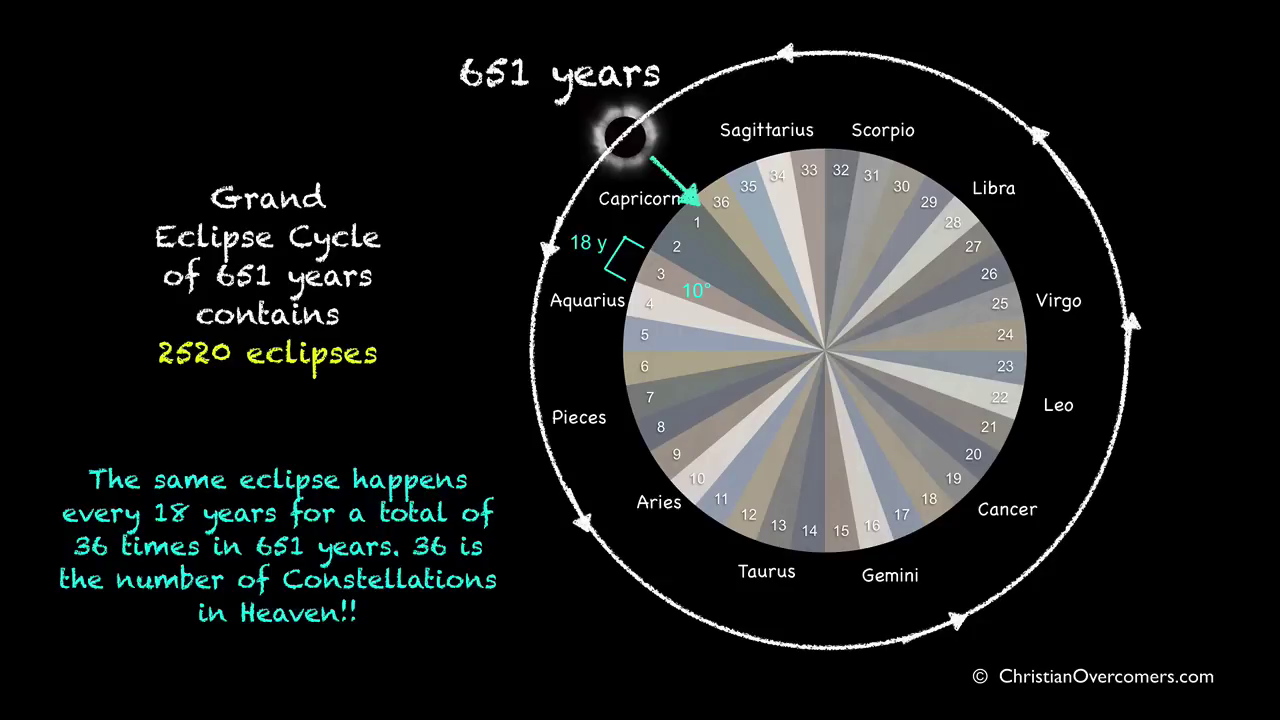
The understanding of these celestial events, the cyclical nature of eclipses, and the positioning of the stars in the firmament contribute to the scientific and astronomical knowledge of our world.
The great cycle in the sky
This is getting complicated quickly, but lets take a moment and think. The lunar cycle is 18 years 11 days 8 hours long. Because it is not a nice even number, the periodicity overlaps a bit from completing a total period, then begins anew slightly ahead of where it began last time. This cycle then repeats, each time a little ahead of where it began, and it does this until this cycle completes the greater cycle around a circle.
Here is a walkthrough video with commentary
[https://share.descript.com/view/YpdlAXF4HLN](https://share.descript.com/view/YpdlAXF4HLN)
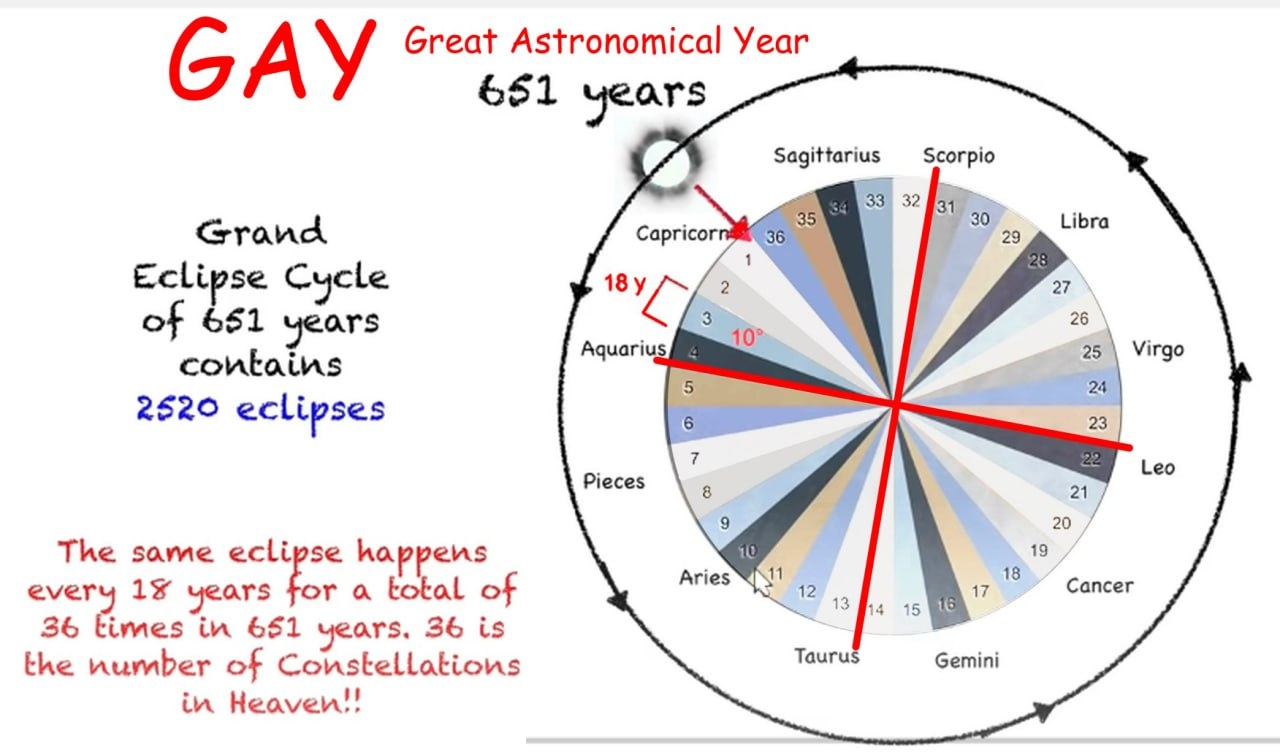

651 great astronomical year (cycles of 325/326),
18 year lunar cycle,
18yr10/11day eclipse cycle,
It takes 18 years for the teams of eclipses to make a 360 around our realm. That is it's cycle.
18 eclipses times 18 year, 10 day
-The 651 GAY is divided into two revolutions,
(A) 325years
(B) 326years alternating,
-2023 is year 162... completes 9 eclipse cycles, half of (A) 325.
-2023 is year 18 in the repeating series or teams of eclipses 18, team 18, in the 18yr eclipse cycle.
-Each year of the 18yr eclipse cycle is made up of a team of eclipses.
The teams never leave their year marker, line of time.
-It takes 18 eclipse cycles to complete (A) and another
18 eclipse cycles to complete (B).
-18 eclipse cycles +18 eclipse cycles =36 eclipse cycles
i**s 325 +326 = 651year astronomical great year (GAY)**
-36 eclipse cycles completes 360° in 18 years to bring the eclipses to their self-same dates and start a new Great astronomical year
-There are 70 eclipses in the 2023 18yr eclipse cycle.
18 * 70 = 1261 Eclipses in a cycle, paring, or soros cycle
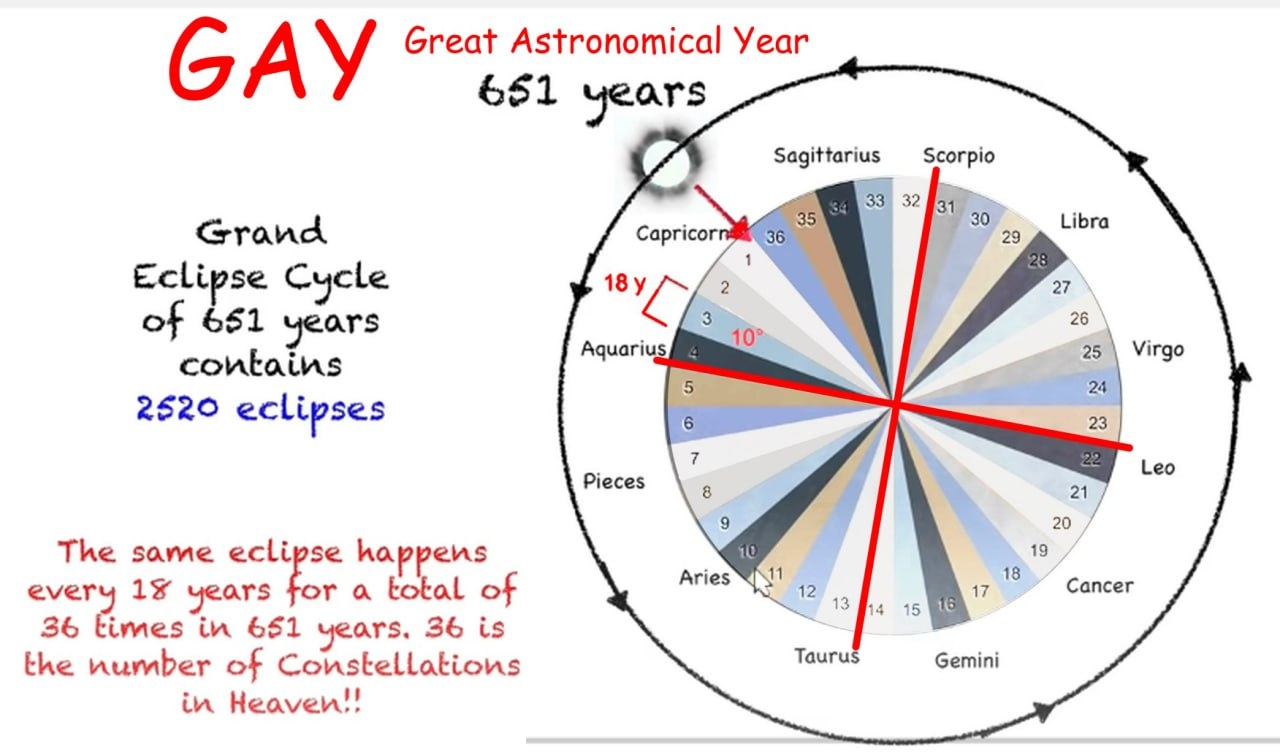
After one saros, the Moon will have completed roughly a whole number amount of synodic, draconic, and anomalistic periods (223, 242, and 239) and the Sun-Moon geometry will be nearly identical: the Moon will have the same phase and be at the same node and the same distance from the Earth. In addition, because the saros is close to 18 years in length (about 11 days longer), the Earth will be nearly the same distance from the Sun, and tilted to it in nearly the same orientation (same season).
However, there are three other orbital periods or months that are crucial to the understanding and prediction of eclipses. These three cycles and the harmonics between them determine when, where, and how solar and lunar eclipses occur. The Saros arises from a natural harmony between three of the Moon's orbital periods: Synodic Month, Anomalistic Month, and Draconic Month.
(period lengths for 2000 CE)
Synodic Month (New Moon to New Moon) = [synodic](https://publish.obsidian.md/shanesql/synodic)
29.530589 days - 29d 12h 44m 03s
Anomalistic Month (perigee to perigee) = The point at which moon is furthest away from earth
27.554550 days - 27d 13h 18m 33s
Draconic Month (node to node) =
27.212221 days - 27d 05h 05m 36s
223 Synodic Months =
6585.3223 days
6585d 07h 43
239 Anomalistic Months =
6585.5375 days
6585d 12h 54m
Draconic Months =
6585.3575 days
6585d 08h 35m
Let's go over some terms NASA uses, in my opinion, in an attempt to intentionally confuse the true nature of the eclipse cycle. First, the types of cycles they broke up the lunar and solar cycle into, then the terms of the lunar cycle and lunar phase.
Types of Cycles within the GAY (Great Astrological Year)
[eclipse cycles](https://en.wikipedia.org/wiki/Eclipse_cycle) of varying lengths can be constructed by combining basic cycles
The [Saros](https://en.wikipedia.org/wiki/Saros_(astronomy)) cycle (18 years 11 days 8 hours) _Just another name for the Lunar Cycle_
the [Inex](https://en.wikipedia.org/wiki/Inex) cycle (29 years minus 20 days) [innex](https://publish.obsidian.md/shanesql/innex)
the [Metonic Cycle](https://en.wikipedia.org/wiki/Metonic_cycle) cycle (19.0 years),
the [Exeligmos](https://en.wikipedia.org/wiki/Exeligmos) cycle (54.1 years),
the [Hipparchus Cycle](https://en.wikipedia.org/wiki/Hipparchic_cycle)cycle (345.0 years)
the Babylonian Period (441.3 years)
An exeligmos is a period of 54 years, 33 days

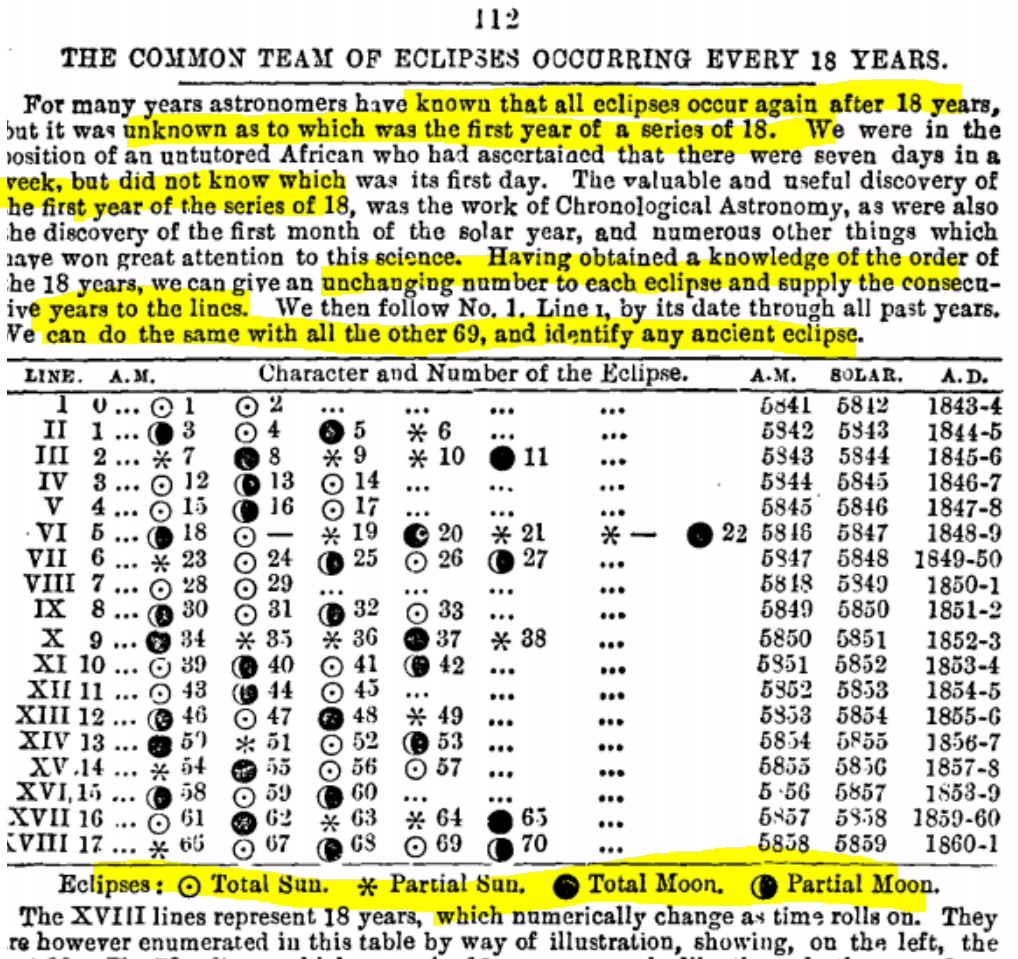

Example of one eclipse pair and the path they take.


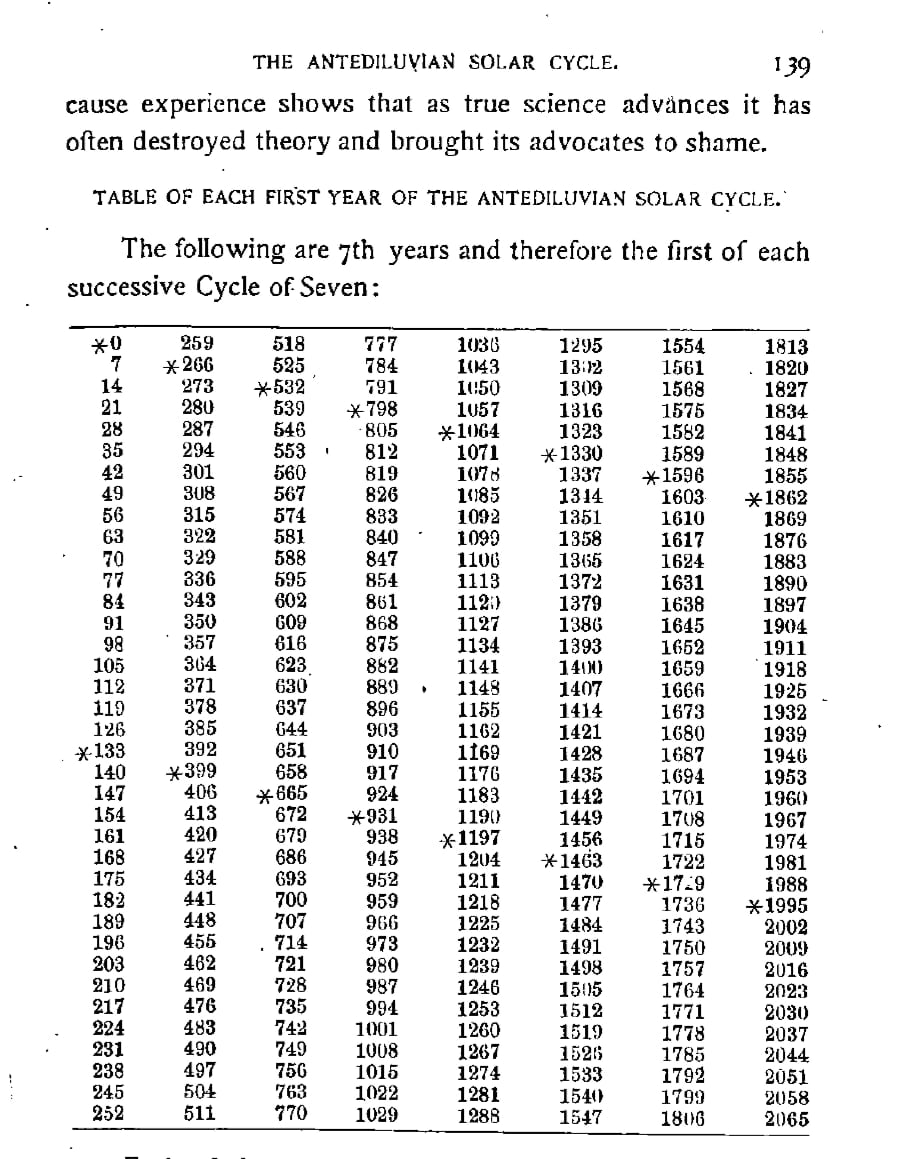


### Teams of Eclipses
[All Past Time - J. B. Dimbleby](https://publish.obsidian.md/shanesql/All+Past+Time+-+J.+B.+Dimbleby)

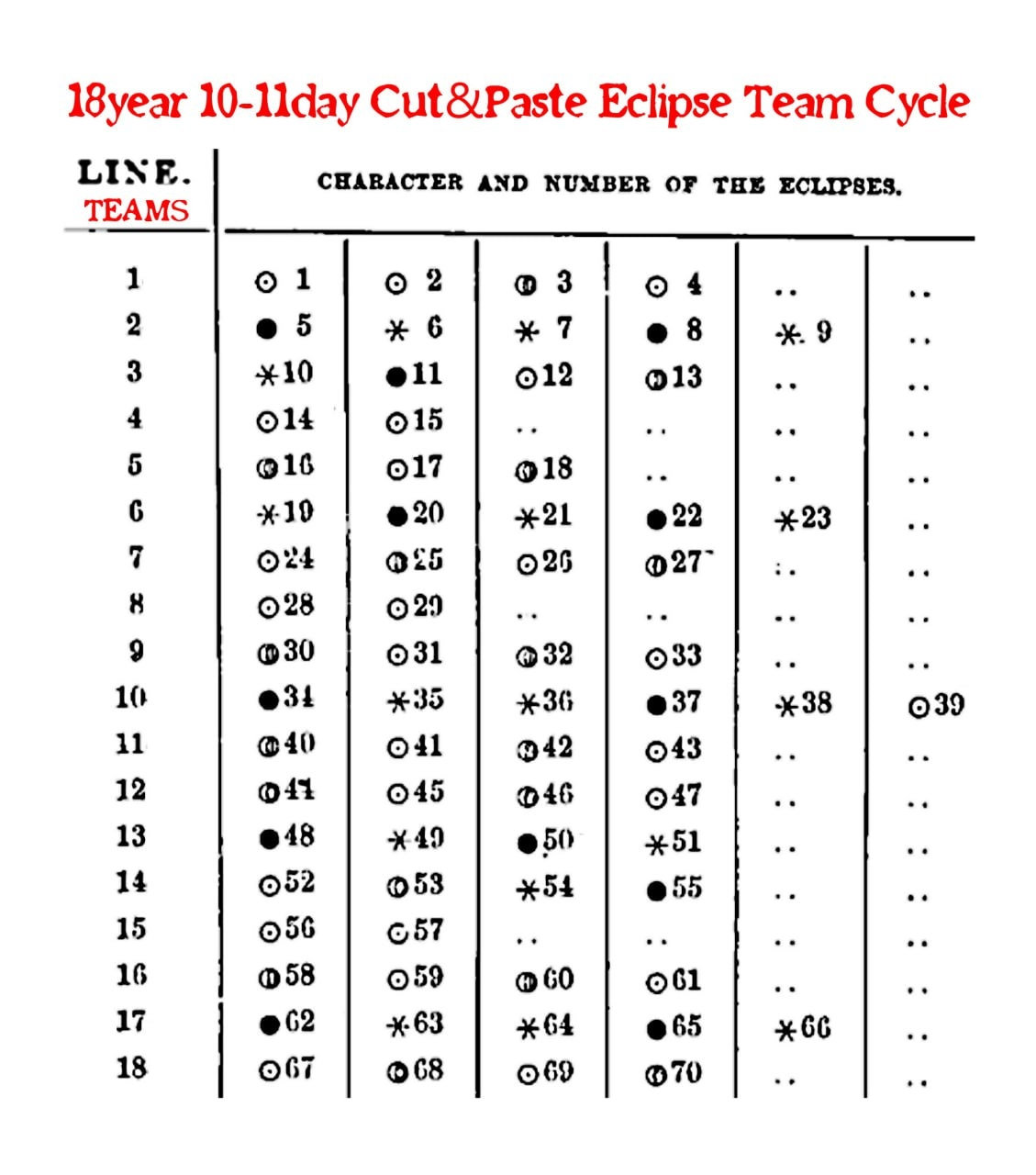


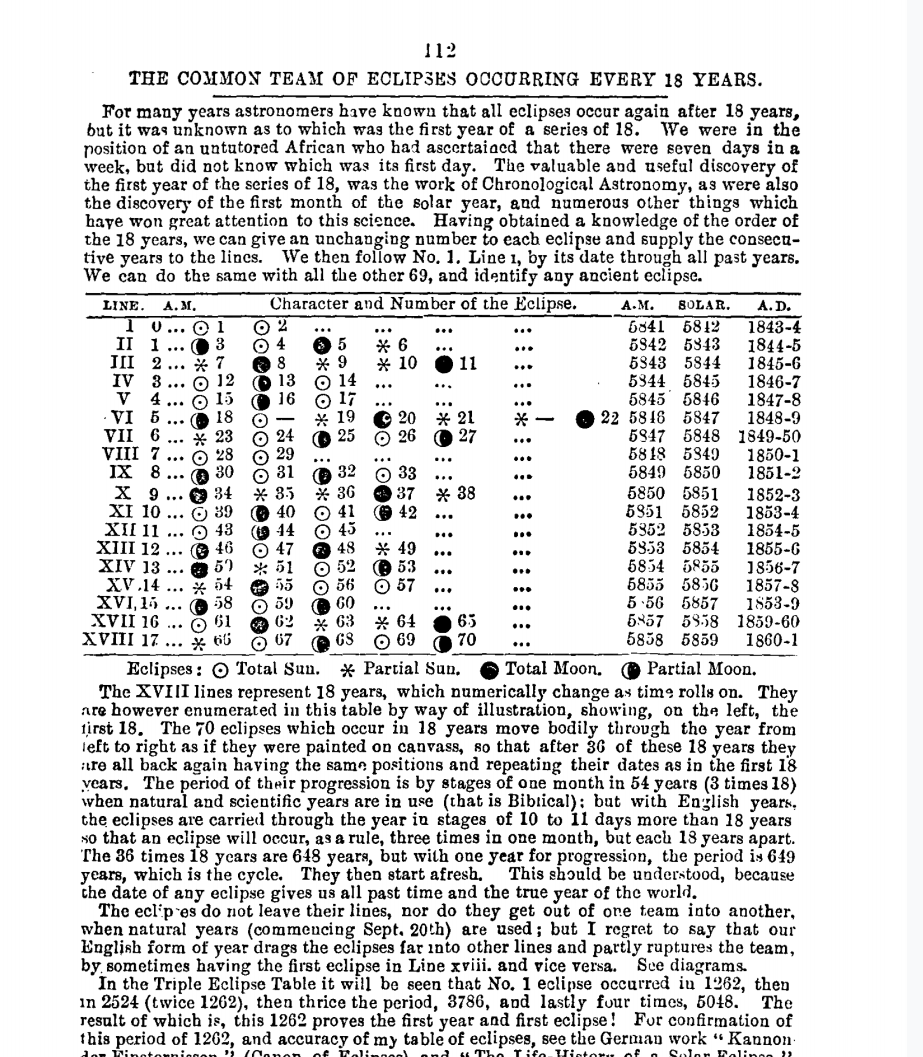
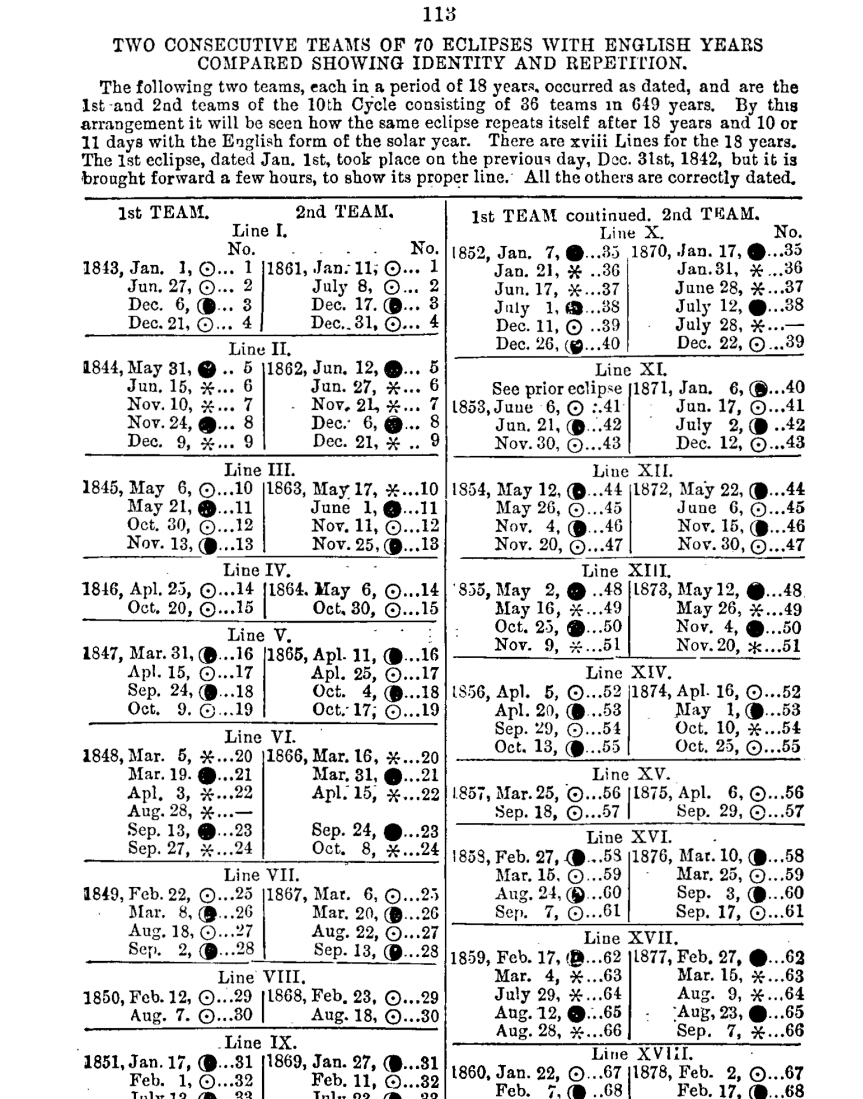

[https://web.descript.com/drives/eb370150-362a-4f1d-b9e2-c866ecd10a6e/join?invite_link_token=sOEeTpHw9o32muRIK7n1k8](https://web.descript.com/drives/eb370150-362a-4f1d-b9e2-c866ecd10a6e/join?invite_link_token=sOEeTpHw9o32muRIK7n1k8)
.gif)


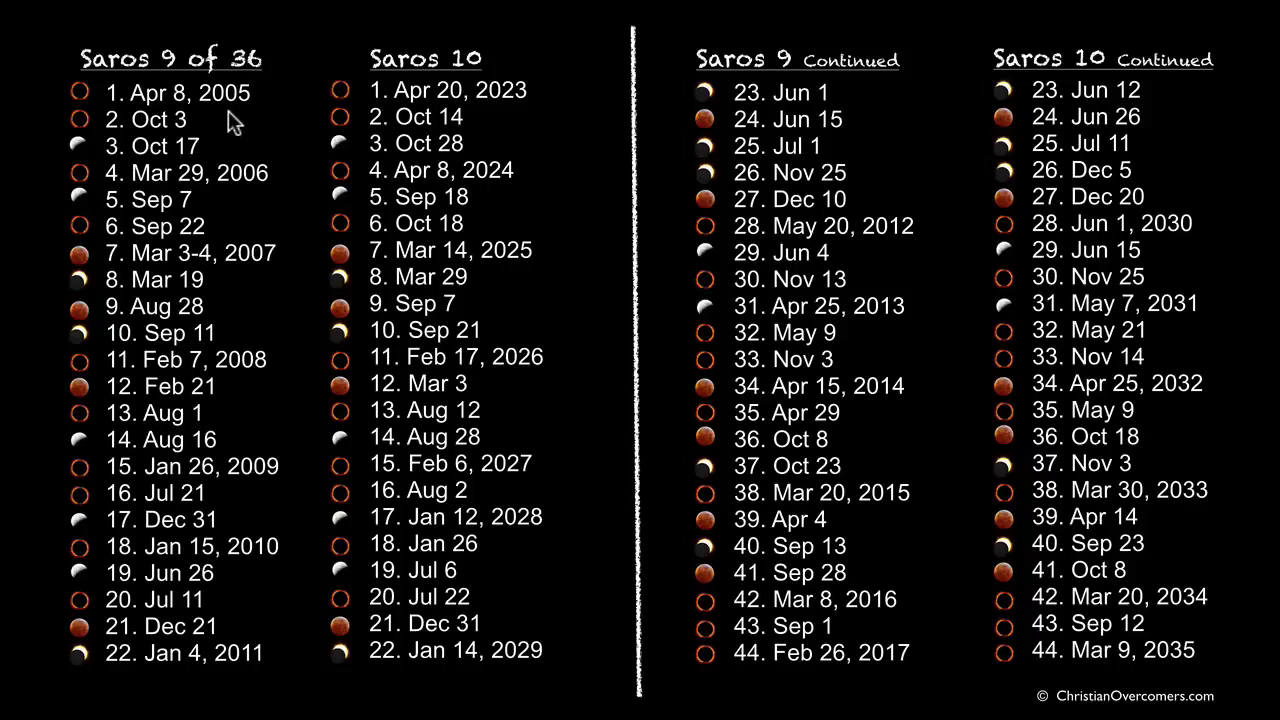

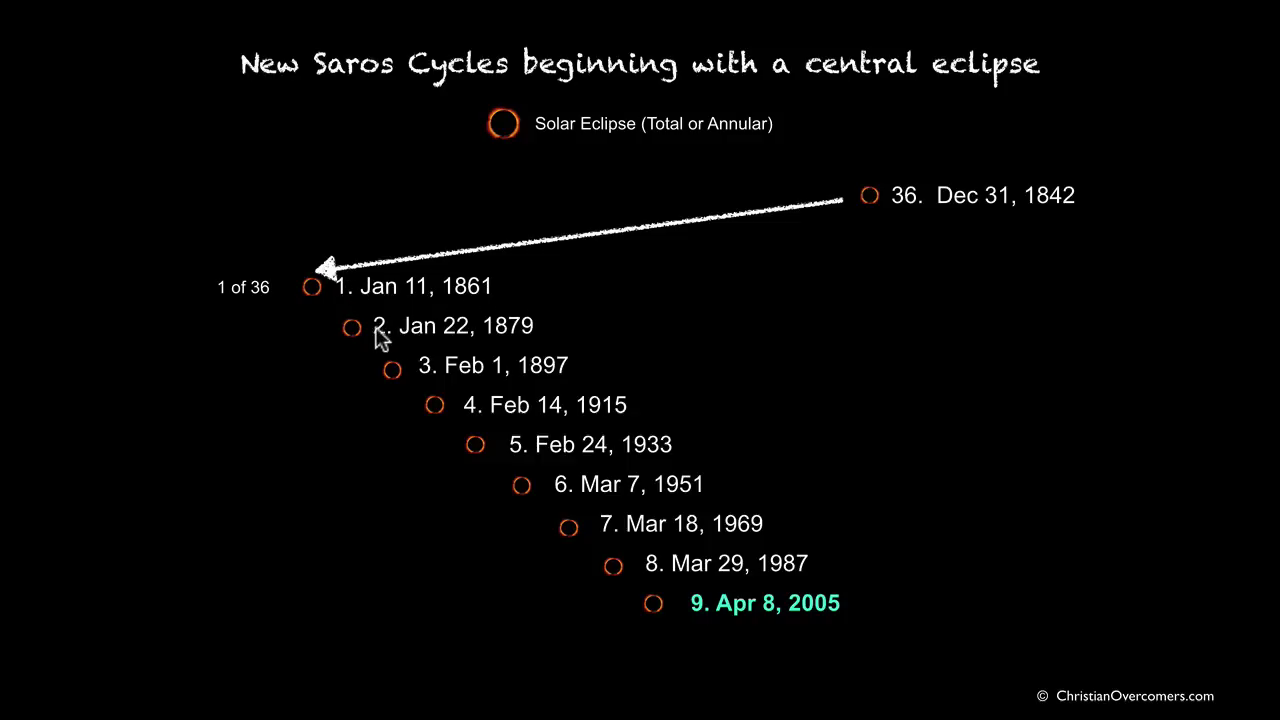

# Saros Inex Panorma
For those who know where it comes from, depictions like this are magnificent, but for everyone else, it means almost nothing.
[https://eclipsewise.com/solar/SEhelp/images/SEpanoramaGvdB-big.jpg](https://eclipsewise.com/solar/SEhelp/images/SEpanoramaGvdB-big.jpg)

[https://eclipse.gsfc.nasa.gov/SEsaros/image/SEpanoramaGvdB-big.JPG](https://eclipse.gsfc.nasa.gov/SEsaros/image/SEpanoramaGvdB-big.JPG)
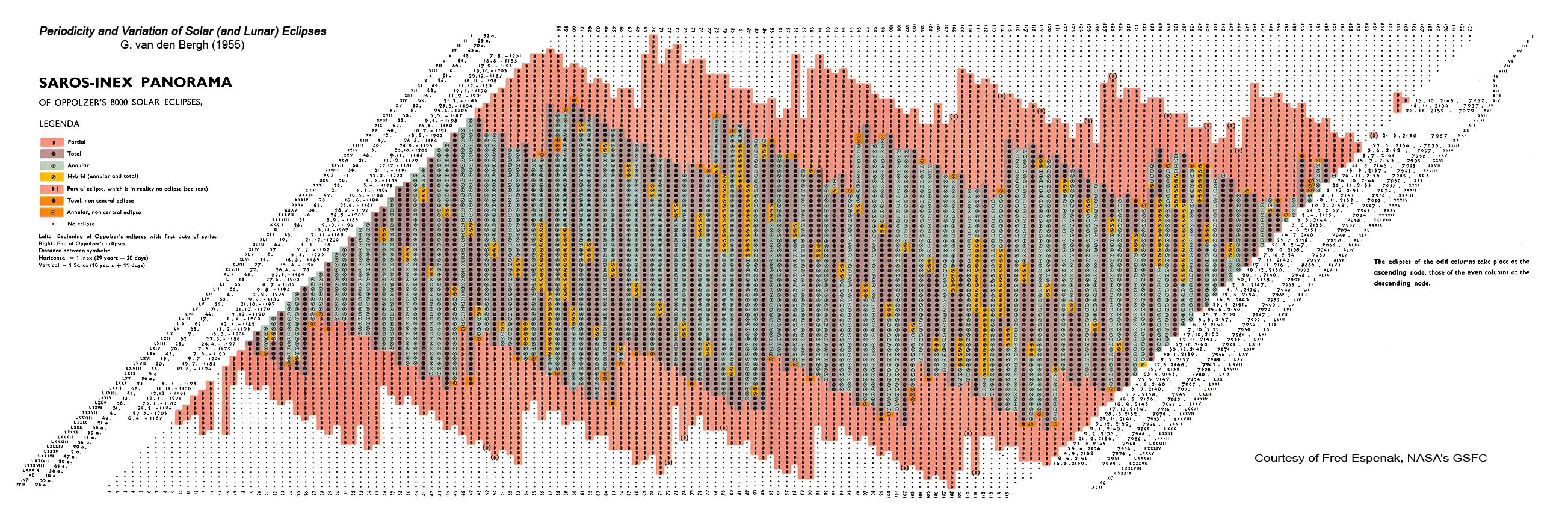
"Van den Bergh placed all 8,000 solar eclipses in von Oppolzer's _Canon der Finsternisse_ (1887) into a large two-dimensional matrix. Each Saros series was arranged as a separate column containing every eclipse in chronological order. The individual Saros columns were then staggered so that the horizontal rows each corresponded to different Inex series. This "Saros-Inex Panorama" proved useful in organizing eclipses. For instance, one step down in the panorama is a change of one Saros period (6585.32 days) later, while one step to the right is a change of one Inex period (10571.95 days) later. The rows and columns were then numbered with the Saros and Inex numbers." - From NASA [https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section109](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section109)
This is a depiction of the complete cycle in action. It is composed of smaller cycles within cycles, all leading back to the solar and lunar cycles. The cycle offsets a bit each time it repeats, creating an oscillation in the data that you can see best when the data is graphed like it is above. This is all a result of the exact time of the Lunar cycle. because of this offset, the actual period of repetition is expanded considerably. This illustrates the offset between cycles very well, and you can clearly see the cyclical nature of the cycle. From this, they were able to extrapolate the exact timing between eclipse parings within the cycle. They were then able to 'predict' the next cycle with even greater accuracy.
# Soros Cycle
The fascinating math behind solar eclipses and the Saros cycle
---
The periodicity and recurrence of eclipses is governed by the [Soros](https://publish.obsidian.md/shanesql/Eclipses#Soros) cycle, but it also includes a host of other factors which have their own cycles, and this leads to the much greater cycle of 651 astronomical years. The Soros is the building block cycle that upper level cycles are built with.
As the moon revolves around the earth in twenty nine and one-half days, the twelve months of the year must alternately possess thirty and twenty-nine days in order to keep up her movements—twelve lunations or months (Moon-eths,) in a year. This is a system now followed by the Jews, Turks and Chinese, w ho use the original Lunar Year, the months of which are more astronomical than our Pagan, Solar year, which, although a perfect measure of time, can begin and end anywhere, and have months of irregular length. The Lunar year, and each month of the year, must begin with a new moon.
It completes an analemma with 29.5 solar days, one lunation, one moonth (month). 12 lunations = 354 solar days.

.gif)

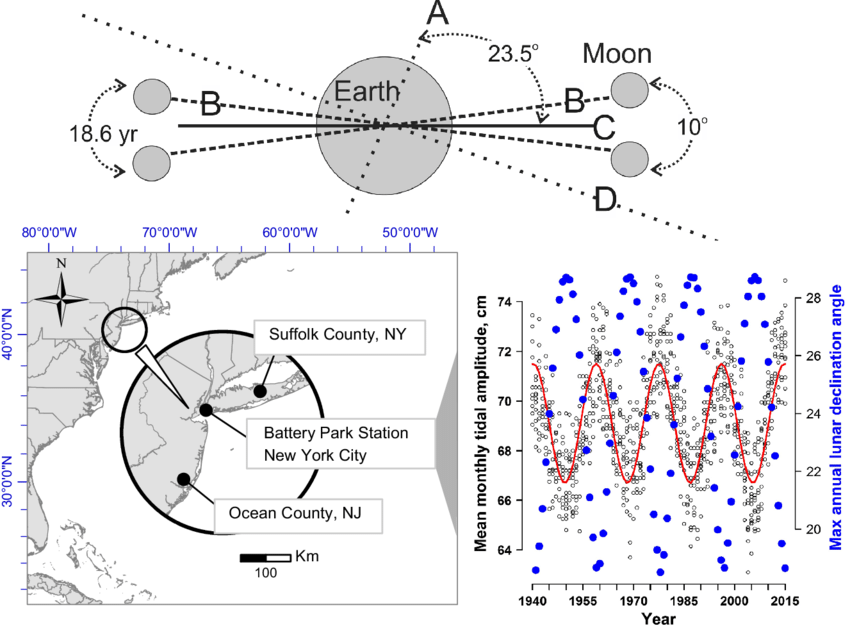
### Thanks Fred!
Who the hell is Fred Espenak??
Fred 'works for nasa' just like a professor with tenure who is on sabbatical 'works' for the Dean of the college. I mean to say, he was scooped up directly because of this knowledge and expertise, something NASA was sorely lacking.

[https://www.mreclipse.com/main/lectures.html#topics](https://www.mreclipse.com/main/lectures.html#topics)
Several new solar eclipse canons were published in the second half of the 20th century. Meeus, Grosjean, and Vanderleen published Canon of Solar Eclipses (1966) containing the Besselian elements of all solar eclipses from +1898 to +2510, together with central line tables and maps. The aim of this work was principally to provide data on future eclipses. The Mucke and Meeus work Canon of Solar Eclipses: –2003 to +2526 (1983) was intended mainly for historical research and served as a modern day successor of Oppolzer’s great canon. Espenak’s Fifty Year Canon of Solar Eclipses (1987) includes individual detailed maps and central path data for all solar eclipses from +1986 to +2035. Finally, the Espenak and Meeus Five Millennium Canon of Solar Eclipses: –1999 to +3000 (2006) contains individual maps of every solar eclipse and uses modern ephemerides of the Sun and Moon.~~*****
- —Fred Espenak and Jean Meeus 2009 January [https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf](https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf)
> > From von Oppolzer to Modern Canons
> > Theodor von Oppolzer's _Canon der Finsternisse_, published in 1887, represents a monumental achievement in the history of computational astronomy, cataloging the elements of solar and lunar eclipses over a span of more than three millennia. Despite its reliance on the 19th-century ephemerides and approximations, von Oppolzer's canon served as the primary reference for eclipse predictions until the advent of electronic computing. This paper reviews the evolution of eclipse canons from von Oppolzer's seminal work through to the present day, highlighting the advancements in accuracy and comprehensiveness enabled by modern computational methods and astronomical theories.
> >
> > The 19th century witnessed remarkable advancements in computational astronomy, among which Theodor von Oppolzer's _Canon der Finsternisse_ stands out. Documenting over 8,000 solar and 5,200 lunar eclipses, this work provided an exhaustive reference for astronomers and historians alike. However, the accuracy of von Oppolzer's canon was limited by the computational resources and astronomical knowledge of his time.
> >
> > Von Oppolzer's canon, encompassing eclipses from 1207 BCE to 2161 CE, was an unparalleled compilation of eclipse data, including maps of central eclipse paths. Despite its reliance on approximations and the less precise ephemerides of the 19th century, it remained the definitive guide for eclipse prediction into the early 20th century.
> >
> > The introduction of electronic computing facilitated significant advances in eclipse prediction. In 1979, Meeus and Mucke's _Canon of Lunar Eclipses: –2002 to +2526_ extended von Oppolzer's work with updated data for 10,936 lunar eclipses, incorporating modern ephemerides and accounting for phenomena such as penumbral eclipses, which were absent in von Oppolzer's work.
> >
> > Recent publications, such as Espenak's _Fifty Year Canon of Lunar Eclipses_ and the _Five Millennium Canon of Lunar Eclipses_ by Espenak and Meeus, have further refined eclipse predictions. These works utilize the latest astronomical theories and offer detailed diagrams and visibility maps for eclipses, covering extensive periods and including a wealth of supplementary data.
> >
> > The evolution of eclipse canons from von Oppolzer's foundational compilation to the comprehensive databases of today illustrates the significant strides made in the field of computational astronomy. These modern canons, underpinned by advanced computational techniques and astronomical theories, offer unprecedented accuracy and detail in eclipse prediction, serving both scientific and historical research.***~~
>
> > > _- Fred Espenak_

The Saros Cycle is a team of repeating sister eclipses (saros series) that repeat the phases of the moon exactly after 235 lunations (synodic months). A series of eclipses that are separated by one saros is called a saros series. A series will span hundreds of years because of the dual nature and spread of time between eclipses. It is not a sequential list of eclipses that occur in order, it is a wide spread list of pairings. Because of the supposed ellipticity of the orbits of the Moon, the exact duration and number of eclipses in a complete Saros series is not constant. The exact period of a Soros cycle is 6585.3211 days (15 common years + 3 leap years + 12.321 days, 14 common years + 4 leap years + 11.321 days, or 13 common years + 5 leap years + 10.321 days). They typically last 1,226 to 1,551 years and is composed of 69 to 87 eclipses, however, the majority (84.6%) are composed of 70 to 73 eclipses.
An eclipse is a relatively rare coincidence of several factors at once in order to make the shadows line up properly.
First of all, it must be a new moon for a solar eclipse or a full moon for a lunar one, so the Sun, Moon and Earth lie in a straight line.
Secondly, these 3 bodies must line up "vertically" so the eclipse shadows aren't too far north or south to be seen from Earth. This means the Sun and Moon must be "near" one of the Moon's Nodes, where the paths of the Sun and Moon cross. ("Near" is roughly an 18 degree orb.)
Finally, the difference between a total eclipse of the Sun and an annular eclipse is in how far apart the Moon and Earth are. At the "perigee" point, when they are closest together, the Moon's umbral shadow (the dark part of the shadow, where totality occurs) can reach all the way to the Earth's surface and form a total eclipse. At the "apogee" point, on the other hand, when the Moon is farthest from the Earth, the tip of the Moon's umbral shadow dangles thousands of kilometers above the Earth's surface, never touching, and we see only an annular eclipse. (See the [Eclipse Geometry page](http://members.bitstream.net/bunlion/bpi/EclGeom.html) for an illustrated explanation of how eclipses are formed.)
So to predict future eclipses, we need to understand 3 interlocking cycles, namely:
- The period of time from one new or full moon to the next. Astronomers call this the "Synodic Month" and have measured its average length as 29.53059 days.
- The period of time it takes the Sun to travel from the Moon's North (or South) Node around the zodiac and back, called the "Draconic Year". Because the Moon's Nodes move backwards 19-20 degrees a year, the Draconic Year is shorter than the usual calendar year by several weeks. It's average length is 346.62005 days.
- The period of time from perigee to perigee in the Moon's orbit, called the "Anomalistic Month". This period averages 27.55455 days.
By "coincidence", these cycles all repeat nearly exactly every 18+ years. This is because:
- 223 Synodic Months = 6585 days, 7 hours, 43+ minutes,
- 19 Draconic Years = 6585 days, 18 hours, 44+ minutes,
- 239 Anomalistic Months = 6585 days, 12 hours, 53+ minutes.
This period of 6585.322 days is called a "Saros Cycle" (named from a Greek word meaning "to repeat"). This is roughly 18 years, 11 days and 8 hours (depending upon how many leap days there are on the calendar). So if there's an eclipse now, in 6585.322 days from now,
- There will be another new or full moon occuring,
- The Sun and Moon will be roughly the same distance along the zodiac from the same Lunar Node again,
- The Earth and Moon will be roughly the same distance apart as they are now.
In short, all the factors that make an eclipse happen now will repeat (with surprising accuracy!) exactly one Saros cycle from now and another eclipse (of very similar geometry) will happen then. This Saros cycle is the very powerful predictive tool that the Babylonians were so clever to discover.
Please note that the eclipses in a Saros Family do **not** happen at the same zodiac position each time. It takes 6798 days (about 18.61 years) for the Moon's Nodes to retrograde around the zodiac and return to the same position. In one Saros period, the Nodes are roughly 11-12 degrees short of returning. So from one Saros period to the next, the Node's move eastward (in the same direction as the Sun moves through the zodiac signs) 11-12 degrees. Therefore, all the eclipse points also drift 11-12 degrees each Saros, gradually sweeping around the zodiac over the centuries. This is the "11+ days" part of the Saros period in action (the Sun moves roughly 1 degree per day).
#### What is the Saros Cycle?
The term **"saros"** refers to an astronomical cycle used to predict eclipses. A **saros** lasts approximately **18 years, 11 days, and 8 hours** (6,585.3 days) and consists of 223 synodic months (lunar phases)
## Key Details:
- **Exact Duration**:
- 18.03 years
- 6,585.32 days
- 223 synodic months (29.5-day lunar cycles)
- 241 draconic months (Moon's orbital nodes alignment)
- 239 anomalistic months (Moon's elliptical orbit phases)
Eclipses separated by one saros **share nearly identical geometry**, occurring at the same lunar node and distance from Earth. However, sidereal rotation shifts visibility westward by ~120° each cycle
[http://members.bitstream.net/bunlion/bpi/EclSaros.html#numbering](http://members.bitstream.net/bunlion/bpi/EclSaros.html#numbering)
#### Innex
It corresponds to:
- 358 lunations ([synodic months](https://en.wikipedia.org/wiki/Synodic_month "Synodic month"))
- 388.50011 [draconic months](https://en.wikipedia.org/wiki/Lunar_month#Draconic_month "Lunar month")
- 30.50011 eclipse years (61 [eclipse seasons](https://en.wikipedia.org/wiki/Eclipse_season "Eclipse season"))
- 383.67351 [anomalistic months](https://en.wikipedia.org/wiki/Anomalistic_month "Anomalistic month").
- 8 eclipse sets
#### How are Saros Cycles numbered?
Obviously with this much confusion going on, it's important to come up with some way to talk about individual Saros Cycles and tell one from another. This requires that we give these cycles some kind of name or number that identifies it. Astronomers have one scheme for numbering Saros cycles, while astrologers have their own scheme.
The astronomical scheme for numbering Saros Cycles is based on a 1955 paper published by a fellow named van den Bergh. Ignoring some crucial details, he more or less numbers the Saros' consecutively as new ones start up, beginning at some remote time in ancient history. If the Sun is near the North Node, the Saros is given an odd number; if near the South Node instead, the Saros number is even. The Saros Cycles for solar and lunar eclipses are numbered separately (be careful you keep straight whether it's solar or lunar when talking about a Saros number!). Once a Saros Cycle is complete after 12-14 centuries, its number is permanently retired. The Saros numbers for solar eclipses are currently (2002) in the range of 117 through 155, while lunar eclipses have Saros numbers between 109 and 149.
The Saros numbers that astrologers use is more complicated and confusing (in my mind). First of all, I've only seen two astrology books that discuss the Saros and both of them only work with solar eclipses. Lunar eclipses are ignored, a big mistake in my estimation. They arbitrarily number consecutive eclipse seasons "1 North" (with the Sun at the North Node), "1 South" (South Node), "2 North", etc. all the way up to "19 South". In addition, if there are 2 eclipses in the same eclipse season (29 days apart), the older Saros Family is called "old" and the younger one "new". This leads to long names like "9 South Old" for a Saros that started in 727 AD, and the newer "9 South New" that began in 1917 AD. When the old cycle dies off, the younger Saros drops "new" from its name. Since names are reused and occasionally change as Saros Cycles come and go, this second scheme seems like confusion waiting to happen.
In the tables of [active Saros Cycles](http://members.bitstream.net/bunlion/bpi/ActSaros.html), I'll use the astronomer's method of numbering for clarity. The current equivalent in the astrologer's scheme will also be given for solar eclipses.
#### Visualization of a complete Soros cycle.
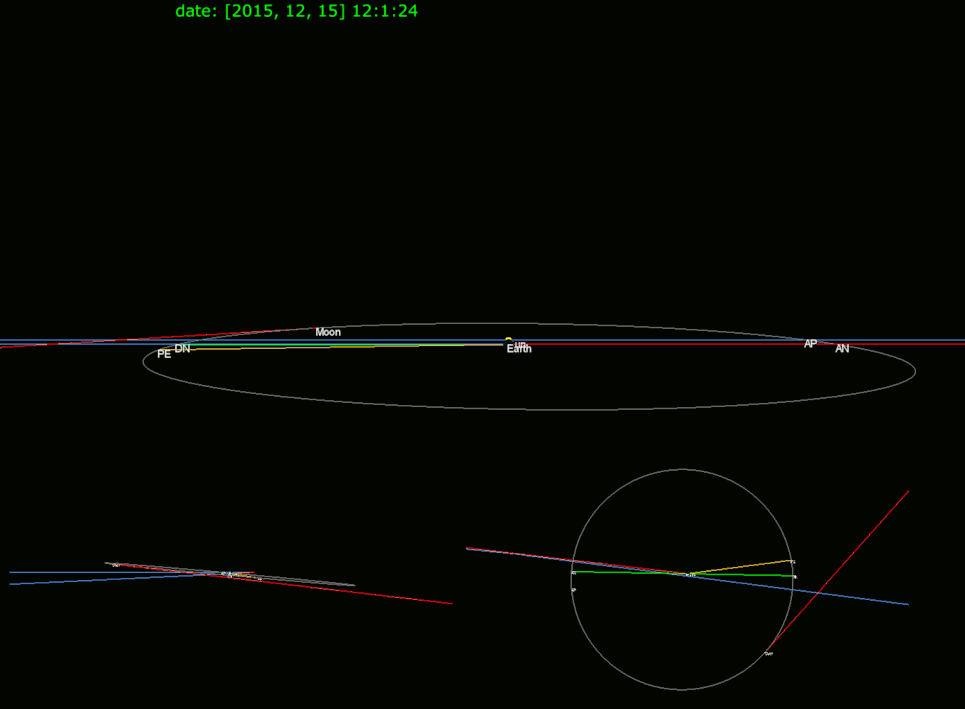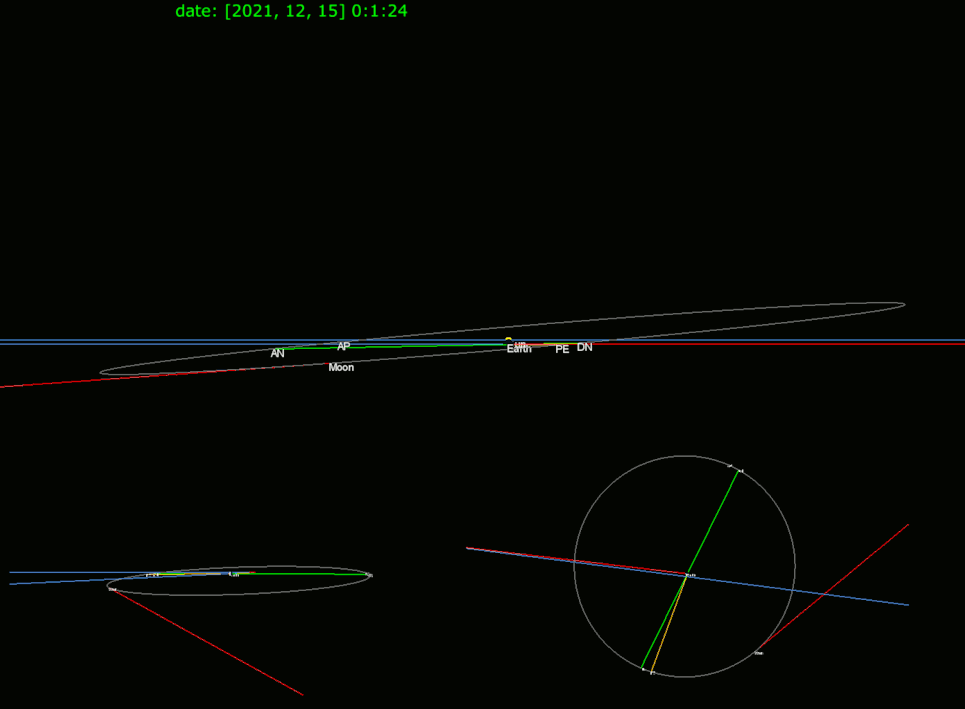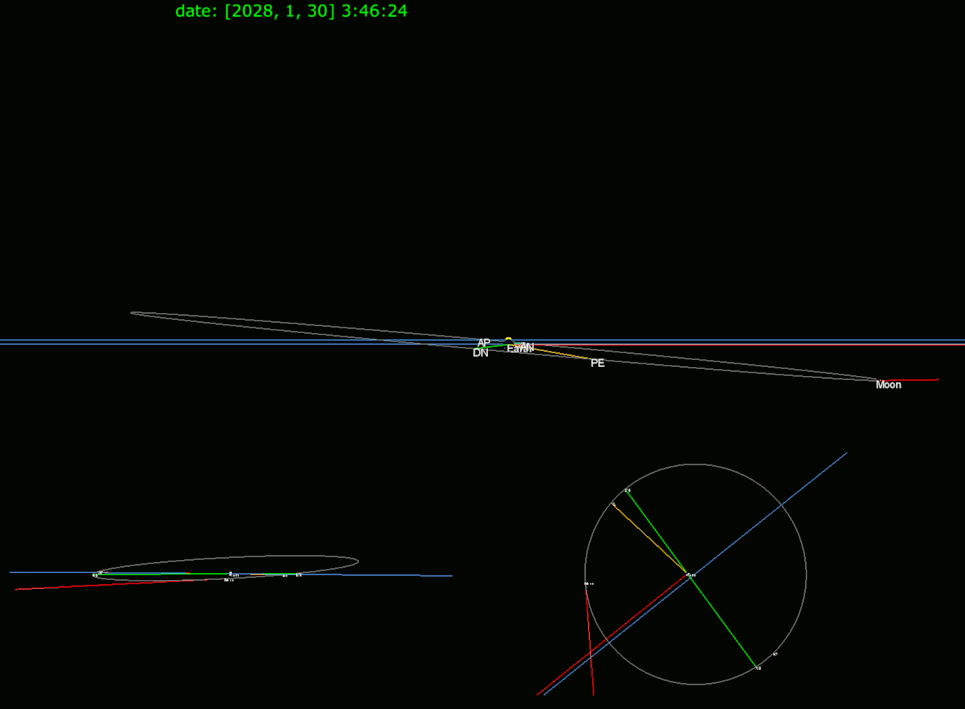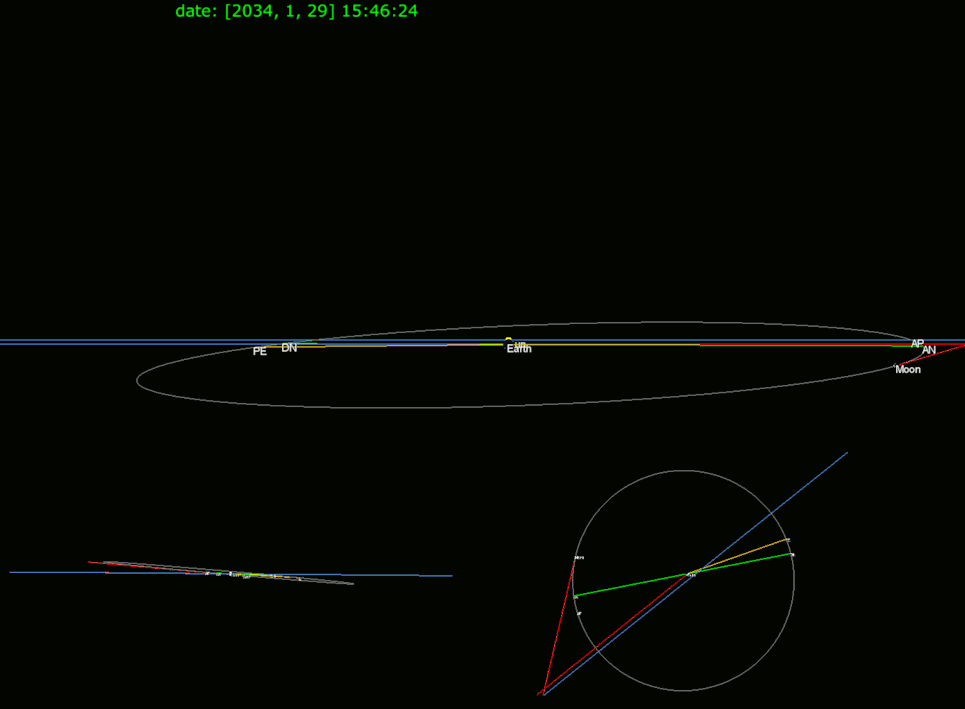
Saros cycle series 131 for lunar eclipses occurs at the moon's descending node, repeats ev8ery 18 years 11 and 1/3 days. It contains 72 member events, with 15 total eclipses, starting in 1950 and ending in 2202.
#### Saros 136
Just for an example, lets take a look at one complete solar cycle. Remember, soros cycles are broken up into types, one series each for Solar and for Lunar.
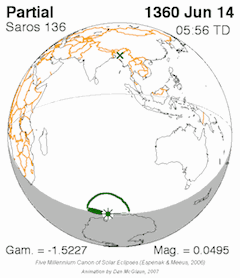
Solar Saros 136, repeating every 18 years, 11 days, contains 71 events. The [series](https://en.wikipedia.org/wiki/Saros_(astronomy) "Saros (astronomy)") started with partial solar eclipse on June 14, 1360, and reached a first annular eclipse on September 8, 1504. It was a hybrid event from November 22, 1612, through January 17, 1703, and total eclipses from January 27, 1721, through May 13, 2496. The series ends at member 71 as a partial eclipse on July 30, 2622, with the entire series lasting 1262 years. The longest eclipse occurred on June 20, 1955, with a maximum duration of totality at 7 minutes, 7.74 seconds. All eclipses in this series occurs at the Moon's descending node.[2](https://publish.obsidian.md/shanesql/2)([https://en.wikipedia.org/wiki/Solar_Saros_136#cite_note-2](https://en.wikipedia.org/wiki/Solar_Saros_136#cite_note-2))
(Don't worry about the globe map, just showing what a soros series looks like all together.)
This Soros cycle from my spreadsheet
### Do Eclipses Repeat Locations?
The scenario for a Saros series at the ascending node is similar except that gamma decreases as each successive eclipse shifts south of the previous one. The southern latitude trend in eclipse paths reverses to the north near the Northern Hemisphere summer solstice.
Saros series do not last indefinitely because the synodic, draconic, and anomalistic months are not perfectly commensurate with one another. In particular, the Moon's node shifts eastward by about 0.48° with each eclipse in a series. The following narrative describes the life cycle of a typical Saros series at the Moon's descending node. The series begins when the New Moon occurs ~17° east of the node. The Moon's umbral/antumbral shadow passes about 3500 km south of Earth and a small partial eclipse will be visible from high southern latitudes. One Saros period later, the umbra/antumbra passes ~250 km closer to Earth's geocenter (gamma increases) and a partial eclipse of slightly larger magnitude will result. After about 10 Saros cycles (~200 years), the first umbral/antumbral eclipse occurs near the Antipoide to the North Magnetic Pole of Earth. Over the course of the next 7 to 10 centuries, a central eclipse occurs every 18.031 years (= Saros), **but will be displaced northward by about 250 km with respect to Earth's center.** Halfway through this period, eclipses of long duration occur near the equator (mid-series eclipses may be of short duration if hybrid or nearly so). The last central eclipse of the series takes place at high northern latitudes. Approximately 10 more eclipses will be partial with successively smaller magnitudes. Finally, the Saros series ends 12 to 15 centuries after it began at the opposite pole.
Based on the above description, the path of each umbral/antumbral eclipse should shift uniformly north in latitude after every Saros period. As Fig. 2 shows, this is not always the case. Nine members from Saros 136 are plotted for the years 2117 through 2261. Although the paths of previous eclipses in this series were shifting progressively northward (Figure 1), the trend here is reversed and the paths shift south. This temporary effect is due to the tilt of the suns orbit above the earth with the passage of Saros 136 eclipses from the Northern Hemisphere's autumnal equinox through winter solstice. Note that the season for this group of eclipses runs from September through December. With each successive eclipse, Earth's Northern Hemisphere tips further and further away from the Sun's ecliptic.
[Saros 136](https://eclipsewise.com/solar/SEsaros/SEsaros136.html) is another example. The series is of particular interest because it is currently producing the longest total solar eclipses of the 20th and 21st centuries. Nine solar eclipses from Saros 136 are plotted above for the years 1937 through 2081. (Again, do not mind the Globe, just illustrating a series for now) The westward ~120° shift of each eclipse path is a consequence of the extra 8 hours in the length of the Saros period. The northward shift of each path is due to the progressive motion of the Moon with respect to its descending node at each eclipse.
[https://eclipsewise.com/solar/SEhelp/images/Saros136-large.pdf](https://eclipsewise.com/solar/SEhelp/images/Saros136-large.pdf)
The Saros cycle of 223 lunar (synodic) months (in Greek numerals, ΣΚΓ′) is in the Antikythera Mechanism made around 150 to 100 BC in Greece, as seen in the picture.

This number is one of a few inscriptions of the mechanism that are visible with unaided eye
#### Saros Return Charts

[https://www.youtube.com/watch?v=d1b4Eyz5O8g&ab_channel=MichelvanBiezen](https://www.youtube.com/watch?v=d1b4Eyz5O8g&ab_channel=MichelvanBiezen)
Every 18+ years, you have a pair of "Saros Return" eclipses. These are the eclipses that are Saros buddies for your solar and lunar prenatal eclipses. These Saros return charts are akin to progressing your prenatal eclipse charts into the future. They show how you are evolving as a "carrier" of the energies of the original eclipse charts, and the changing demands as you face the next 18 years. While I haven't studied these charts much, my gut instinct is they should be quite revealing.
c
An eclipses of the Sun can only take place at New Moon (Sun-Moon conjunction), and only if the Moon is near its orbital node (ascending, or descending). The eclipse zone is about +/- 18.5°, centered on the Moon's node [(eclipse of 1935 Jan 05 05:35 UT)](http://www.jgiesen.de/saros/eclipse1935.html). The Sun travels along the ecliptic at about 1° per day and requires about 37 days to cross through the eclipse zone centered on each node. New Moon occurs every 29 1/2 days and thus guarantees at least one eclipse during each of the Sun's node crossings. The period during which the Sun is near a node is called an eclipse season and there are two eclipse seasons each year. The saros arises from a natural harmony between three of the Moon's orbital periods
The periodicity and recurrence of eclipses is governed by the saros cycle, a period of approximately 6,585.3 days (18 years 11 days 8 hours). It was known to the Chaldeans as a period when lunar eclipses seem to repeat themselves, but the cycle is applicable to solar eclipses as well.
Saros series contain 69 to 86 eclipses. Every series begins with a number of partial eclipses near the north or south polar regions. The series will then produce several dozen central eclipses before ending with a group of partial eclipses near the opposite pole. Odd numbered Saros series occur at the ascending node of the Moon's orbit. They begin with a partial eclipse at high northern latitudes. Even numbered Saros series occur at the descending node of the Moon's orbit and start with a partial eclipse.
#### Saros and Inex
A number of different eclipse cycles were investigated by van den Bergh, but the most useful were the Saros and the Inex.
The Inex is equal to 358 synodic months (~29 years less 20 days), which is very nearly 388.5 draconic months.
358 Synodic Months = 10,571.9509 days = 10,571d 22h 49m
388.5 Draconic Months = 10,571.9479 days = 10,571d 22h 55m
Inex= 358 synodic months (10571.95 days)
m and n are integral numbers (zero, negative or positive).
The extra 0.5 in the number of draconic months means that eclipses separated by one Inex period occur at opposite nodes. Consequently, an eclipse visible from the Northern Hemisphere will be followed one Inex later by an eclipse visible from the Southern Hemisphere, and vice versa. The Inex is equal to ~383.67 anomalistic months, which is far from an integer number. Thus, eclipses separated by one Inex will very likely be of different types, especially if they are central (i.e., total or annular).
The mean time difference between 358 synodic months and 388.5 draconic months making up an Inex is only 6 min. In comparison, the mean difference between these two cycles in the Saros is 52 min. This means that after one Inex, the shift of the Moon with respect to the node (+0.04°) is much smaller than for the Saros (-0.48°). While a Saros series lasts 12 to 15 centuries, an Inex series typically lasts 225 centuries and contains about 780 eclipses. Source - [https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section108](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section108)
**anomalistic month** - The time required for the Moon to make one revolution around its orbit with respect to the perigee; the length of the mean anomalistic month as calculated for the year 2000 is 27.55455 days (27d 13h 18m 33s); the actual duration can vary by several days due to the gravitational perturbations of the Sun on the Moon's eccentric orbit. The number of anomalistic returns of the Sun (18.029) and the Moon (238.992) are nearly whole numbers.
Saros Numbers of Solar Eclipses 1999 to 2024
[http://www.jgiesen.de/saros/SarosNumbers.html](http://www.jgiesen.de/saros/SarosNumbers.html) **
**Total Solar Eclipses 1999 to 2024
A solar eclipse will be total if the new Moon is at perigee** (closest to the Earth, making it look larger) and, in most cases, the Sun is at apogee (farthest from the Earth, making it look smaller, around July 4).
**Annular Solar Eclipses 1999 to 2024**
An annular solar eclipse eclipse happens when the Moon is at **apogee** (farthest from the Earth, making it look smaller), and, in most cases, the Sun is at perihelion (closest to the Earth, making the solar disc appear larger, around January 3)
Saros series contain 69 to 86 eclipses. Every series begins with a number of partial eclipses near the north or south polar regions. The series will then produce several dozen central eclipses before ending with a group of partial eclipses near the opposite pole. Odd numbered Saros series occur at the ascending node of the Moon's orbit. They begin with a partial eclipse at high northern latitudes. Even numbered Saros series occur at the descending node of the Moon's orbit and start with a partial eclipse at high southern latitudes.
In (2008), there were 39 active Saros series numbered 117 to 155.
All Saros series begin and end with a number of partial eclipses. Among the 204 Saros series with members falling within the scope of the _[Canon](https://eclipsewise.com/pubs/5MCSE2.html)_ and _[Catalog](https://eclipsewise.com/pubs/5MKSE2.html)_, the number of partial eclipses in the initial phase ranges from 6 to 25. Similarly, the number of partial eclipses in the final phase varies from 6 to 24. The middle life of a Saros series is composed of umbral/antumbral eclipses (i.e., annular, total, or hybrid), which range in number from 39 to 59. Table 3 presents the statistical distribution in the number of umbral/antumbral eclipses in the Saros series represented in the _[Canon](https://eclipsewise.com/pubs/5MCSE2.html)_ and _[Catalog](https://eclipsewise.com/pubs/5MKSE2.html)_. - [https://eclipsewise.com/solar/SEhelp/SEperiodicity.html](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html)
Saros series are numbered according to the type of eclipse (lunar or solar). An odd numbered series (for solar eclipses) the Sun is near the ascending node, whereas in even numbered series it is near the descending node (this is reversed for lunar eclipse saros series). Generally, the ordering of these series determines the time at which each series peaks, which corresponds to when an eclipse is closest to one of the lunar nodes. After a complete saros cycle a new moon will take place at the same node of the orbit of the Moon, and under these circumstances another eclipse can occur.
#### One Saros Cycle Visualized
Visualization of a period of one saros cycle in 3D.
Saros cycle series 131
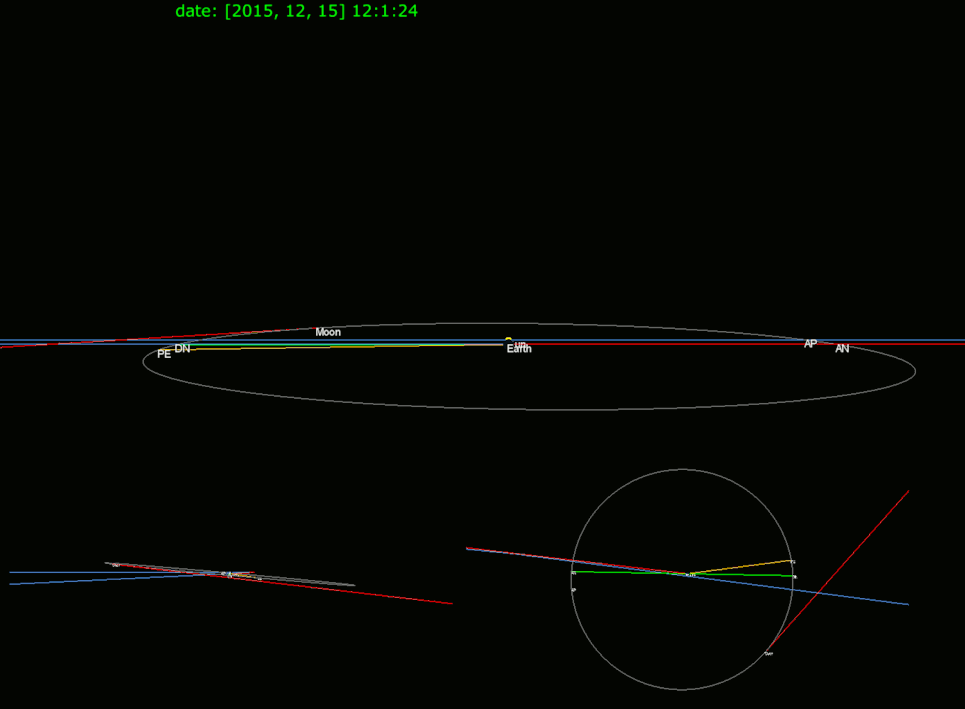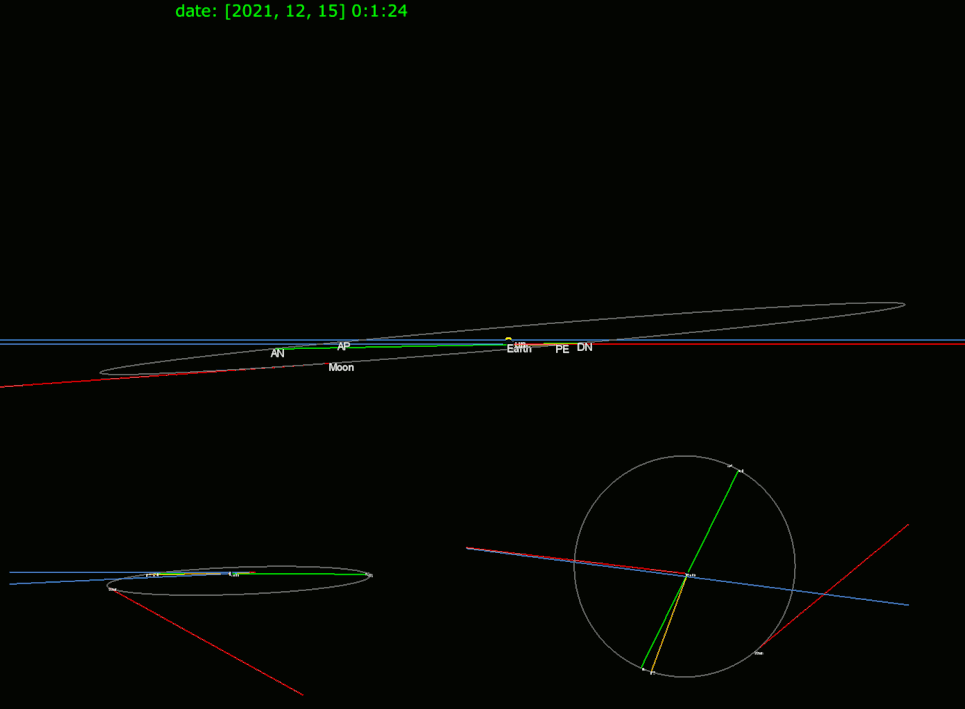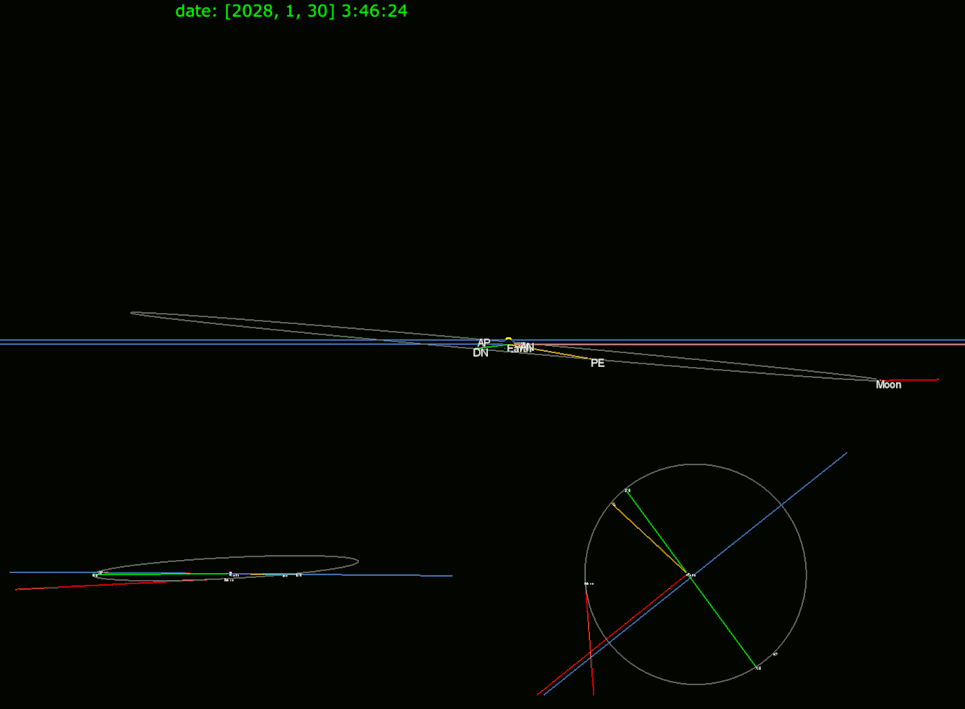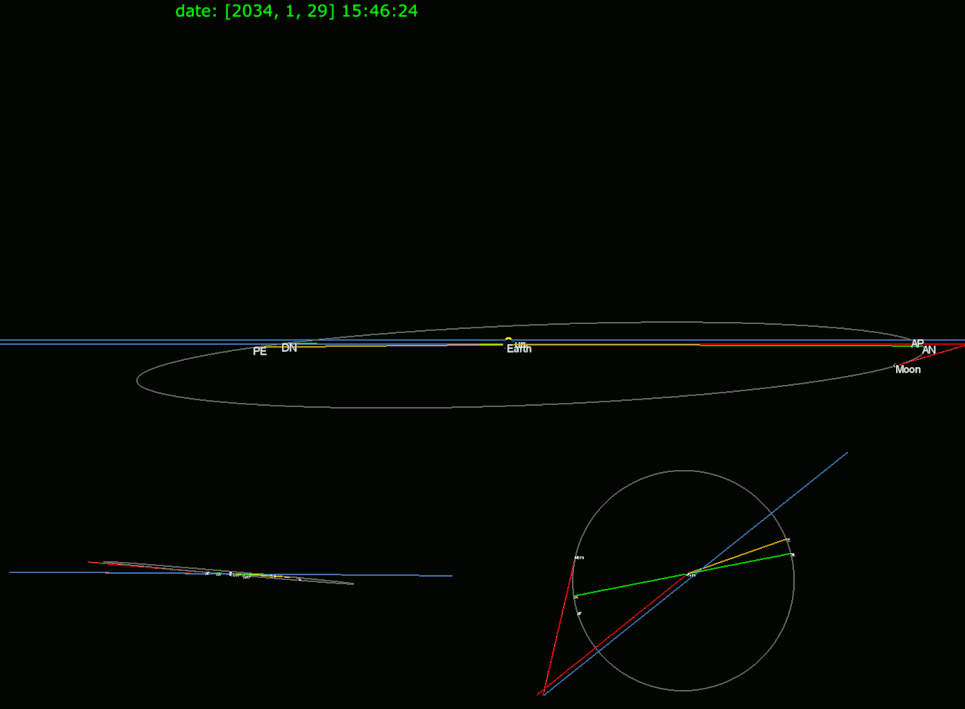
Saros cycle series 131 for lunar eclipses occurs at the moon's descending node, repeats every 18 years 11 and 1/3 days. It contains 72 member events, with 15 total eclipses, starting in 1950 and ending in 2202.
After one saros, the Moon will have completed roughly an integer number of synodic, draconic, and anomalistic periods (223, 242, and 239) and the Sun-Moon geometry will be nearly identical: the Moon will have the same phase and be at the same node and the same distance from the Earth. In addition, because the saros is close to 18 years in length (about 11 days longer), the Earth will be nearly the same distance from the Sun, and tilted to it in nearly the same orientation (same season). Given the date of an eclipse, one saros later a nearly identical eclipse can be predicted. During this 18-year period, about 40 other solar and lunar eclipses take place, but with a somewhat different geometry. One saros equaling 18.03 years is not equal to a perfect integer number of lunar orbits (Earth revolutions with respect to the fixed stars of 27.32166 days sidereal month), therefore, even though the relative geometry of the Earth–Sun–Moon system will be nearly identical after a saros, the Moon will be in a slightly different position with respect to the stars for each eclipse in a saros series. The axis of rotation of the Earth–Moon system exhibits a precession period of 18.59992 years
Given the date of an eclipse, one saros later a nearly identical eclipse can be predicted. During this 18-year period, about 40 other solar and lunar eclipses take place, but with a somewhat different geometry. One saros equaling 18.03 years is not equal to a perfect integer number of lunar orbits (Earth revolutions with respect to the fixed stars of 27.32166 days sidereal month therefore, even though the positions of the celestials will be nearly identical after a saros, the Moon will be in a slightly different position with respect to the stars for each eclipse in a saros series. The axis of rotation of the Moon system exhibits a precession period of 18.59992 years
As an example of a single saros series, this table gives the dates of some of the 72 lunar eclipses for saros series 131. [Shane's Spreadsheet]
This eclipse series began in AD 1427 with a partial eclipse at the southern edge of the Earth's shadow when the Moon was close to its descending node. In each successive saros, the Moon's orbital path is shifted northward with respect to the Earth's shadow, with the first total eclipse occurring in 1950. For the following 252 years, total eclipses occur, with the central eclipse in 2078. The first partial eclipse after this will occur in the year 2220, and the final partial eclipse of the series will occur in 2707. The total lifetime of lunar saros series 131 is 1280 years. [Solar saros 138](https://en.wikipedia.org/wiki/Solar_Saros_138 "Solar Saros 138") interleaves with this lunar saros with an event occurring every 9 years 5 days alternating between each saros series.
Any two eclipses separated by one Saros cycle share similar characteristics. [Eclipse Attributes Repeat with the Soros Cycle](https://publish.obsidian.md/shanesql/Eclipses#Eclipse%20Attributes%20Repeat%20with%20the%20Soros%20Cycle) They occur at the same node with the Moon at nearly the same distance from Earth and at the same time of year. Because the Saros period is not equal to a whole number of days, it has a remainder that causes the procession through the zodiac. The extra 1/3 day displacement means that The sky above earth must rotate an additional ~8 hours or ~120° with each cycle. For solar eclipses, this results in a shift of each succeeding eclipse path by ~120° west. Thus, a Saros series returns to approximately the same geographic region every three Saros periods (~54 years and 34 days). This triple Saros cycle is known as the Exeligmos. Figure 1 shows the path of totality for nine eclipses belonging to Saros 136. This series is of particular interest because it is currently producing the longest total eclipses of the 20th and 21st centuries. The westward migration of each eclipse path from 1901 through 2045 illustrates the consequences of the extra 1/3 day in the Saros period. The northward shift of each path is due to the progressive increase in gamma from -0.3626 (in 1901) to 0.2116 (in 2045). The color figure [Recent Eclipses of Saros 136](https://eclipsewise.com/solar/SEhelp/images/Saros136-large.pdf) shows nine eclipse paths from the series (1937 through 2081).
#### How Many Saros Families are Happening at Once?
As I've already said, eclipses only happen within 18 days of when the Sun is near one of the Moon's Nodes. These month long windows are called "eclipse seasons"; between 1 and 3 eclipses can happen each window. With eclipses happening every half Draconic year (173+ days) and 19 Draconic years to a Saros, it's obvious that not all eclipses are part of the same Saros Family. In fact, each eclipse season is a different Saros Family of solar eclipses and similarly for lunar eclipses.
In addition, as old Saros Families die out, new cycles begin to replace them and frequently overlap in time. Hence there can be multiple Saros Families active in a single eclipse season. In general, there will be 38-42 solar Saros Cycles active at any given time and a similar number of lunar Saros Cycles, each at different stages of their evolution. The total pattern of Saros Cycles is very complicated.
#### Why do these Saros Families Evolve over time?
If these 3 cycles repeated **exactly** every 6585.322 days, eclipses would repeat perfectly every Saros Cycle. However, these cycles are slightly out of sync with each other, causing the geometry of one eclipse to change slightly when its "Saros buddy" comes around 18+ years later. In particular, notice that 19 Draconic Years minus 223 Synodic Months is about 11 hours. This 11 hour gap is the key to explaining why Saros Families evolve over time.
If you have an eclipse A at one time, and eclipse B one Saros period later, the Sun and Moon will line up again to form a new or full moon. However, the Sun will not have returned to its original distance from the Node (the position of eclipse A), since it has 11 hours yet to travel before it completes that 19th Draconic Year. The Sun moves an average of 27 minutes of arc (roughly half a degree) in 11 hours, so eclipse B has drifted half a degree westward relative to the Node compared to A. Remember that the Sun and Moon must be within 18 degrees of the Node for an eclipse to happen. Because of this half-degree drift, Saros families have a beginning and an end, typically encompassing 70-80 consecutive eclipses before the family quits producing. This entire life cycle of a Saros Family lasts 12 to 14 centuries (70 or 80 times 18+ years).
Assume we are looking at a Saros Family of solar eclipses where the Sun is at the North Node. The first eclipse in the family will happen when the Sun is about 18 degrees east of the Node and hence the Moon is about 1.5 degrees north of the ecliptic. The Moon's shadow is so far north that it only grazes the Earth's surface briefly near the Arctic regions, forming a partial eclipse near the North Pole. The next eclipse 18+ years later will be a half-degree closer to the Node, bringing the shadow path farther south. The eclipse will be "deeper" (more of the Sun's disk is covered up), longer in duration, and cover more of the Earth's surface. In addition, the shadow path moves southward towards the equator. Eventually, after 10-11 eclipses, the Moon's umbra touches the Earth, causing a total (or annular) eclipse. The eclipses that follow will all be total (or annular), with the longest duration eclipses happening near the equator halfway through the Saros Family (after 35-40 eclipses, when the eclipse points are right at the Node). The second half of the Family is the mirror image of the first half. The eclipses continue their march towards the south as the eclipse points fall behind the Node. After a number of total eclipses, the Family finishes up with 10-11 partial eclipses around the South Pole. Eventually, the new moon is too far from the North Node, causing the Moon's shadow to completely miss the Earth, and the Saros Family comes to a close.
If the solar eclipses are at the South Node, the cycle is the same except that the eclipses start at the South Pole and march towards the North Pole.
A similar evolution occurs with the Saros Cycle for a family of lunar eclipses. If the Sun is at the North Node, the Moon is at the South Node. At the first eclipse of the Saros Family, the Moon is east of the South Node, hence south of the ecliptic, and just grazes the "lower" (southern) part of the Earth's shadow. Initially, the eclipses are an appulse (where the Moon passes only through the Earth's penumbra, the light part of the shadow). With each eclipse in the Saros Family, the eclipse point drifts towards the Node more, hence closer to the ecliptic and closer to the center of the Earth's shadow. As the eclipses eventually enter the Earth's umbra (the dark central part of the shadow), they become partial and then total lunar eclipses. In the second half of the Saros Family, the eclipse points fall behind the South Node and the Moon moves further north each time. Total eclipses turn to partial ones, then appulses. Finally, the full moon moves too far away from the Node and the Family stops. If the Moon is at the North Node, reverse these directions.
# 'Predicting' Eclipses Cycles, Patterns, and the Procession
The interaction and harmonics of the synodic, anomalistic, and draconic months not only determine how frequently eclipses occur, but they also control the geometric characteristics and classification of each eclipse. The commensurability of these periods over long time scales results in several important eclipse cycles, which are discussed in [Periodicity of Solar Eclipses](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html) and [Periodicity of Lunar Eclipses](https://eclipsewise.com/lunar/LEhelp/LEperiodicity.html).
[https://eclipse.gsfc.nasa.gov/SEhelp/moonorbit.html](https://eclipse.gsfc.nasa.gov/SEhelp/moonorbit.html)
### ECLIPSES AND THE MOON'S ORBIT
### Interval Between Two Successive Solar Eclipses
The time interval between any two successive solar eclipses can be either 1, 5, or 6 lunations (synodic months).
Earth will experience 11,898 eclipses of the Sun during the 5000-year period from -1999 to +3000 (2000 BCE to 3000 CE. As published in in both the _[Five Millennium Canon of Solar Eclipses: -1999 to +3000](https://eclipsewise.com/pubs/5MCSE2.html)_ and _[Five Millennium Catalog of Solar Eclipses: -1999 to +3000](https://eclipsewise.com/pubs/5MKSE2.html)_, the distribution of the 11,897 intervals between these eclipses is found in Table 1
The interaction and harmonics of the synodic, anomalistic, and draconic months not only determine how frequently eclipses occur, but they also control the geometric characteristics and classification of each eclipse. The commensurability of these periods over long time scales results in several important eclipse cycles, which are discussed in [Periodicity of Solar Eclipses](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html) and [Periodicity of Lunar Eclipses](https://eclipsewise.com/lunar/LEhelp/LEperiodicity.html).
### ECLIPSE FREQUENCY AND RECURRANCE
[https://www.strickling.net/saros.htm](https://www.strickling.net/saros.htm)
Having established the preliminary geometry for solar eclipses a question immediately arises. Why doesn't a solar eclipse occur at every new Moon? Since the Moon cycles through its phases every 29 1/2 days or one synodic month, one would expect an eclipse to occur during each conjunction with the Sun. If the Moon's orbit around the Earth were in the same plane as the Earth's around the Sun, this is precisely what would happen. However, the Moon's orbit is inclined 5° to the Earth's. Our planet's natural satellite passes through the ecliptic only twice a month at a pair of points called the nodes. The rest of the time the Moon is either above or below the plane of the Earth's orbit. Since an eclipse can only occur when the Sun, Moon and Earth lie in the same plane, these conditions are met when new Moon takes place at one of the nodes. Contrary to popular belief, solar eclipses are not at all rare. In fact, they're more common than lunar eclipses. An examination of the exterior tangents which delineate the Earth's umbra will substantiate this claim. An eclipse is possible only when the Moon is within that section of its orbit inscribed by the exterior tangents. However, the sunward arc of the Moon's orbit is clearly longer than the anti-sunward arc which passes through the shadow. The number of solar and lunar eclipses that occur are proportional to the lengths of these two arcs. Thus, solar eclipses out number lunar eclipses by almost 5 to 3. In this argument, the Earth's penumbral shadow has been ignored since penumbral lunar eclipses are essentially unobservable. In any one calendar year, there are at least two and as many as five solar eclipses. On the other hand, there can be no more than three lunar eclipses per year and it's quite possible to have none at all. Combining both solar and lunar eclipses, it's possible for one calendar year to contain a maximum of seven eclipses. However, they can only occur in the combinations of five solar and two lunar or four solar and three lunar. In either case, the solar eclipses must all be partial. As a point of interest, 1982 happened to be one of the rare years containing seven eclipses. What made it even more remarkable was the fact that all three lunar eclipses were total. This will not happen again until the year 2485 AD.
The previous discussion contradicts common experience because lunar eclipses are observed more frequently than solar eclipses. The conflict is resolved since solar eclipses are only visible from isolated regions of the Earth while lunar eclipses are visible from the entire night time hemisphere of our planet. An examination of the geometry of the nodes yields further clues on the subject of eclipse recurrence. Since the Sun and Moon both subtend significant angles, neither one has to be exactly at the nodes for an eclipse to occur. In addition, an observer's position on the surface of the Earth introduces a sizable parallax of 2° in ecliptic latitude. These factors make a solar eclipse possible whenever the Sun is within 18.5° of a node. The Sun travels along the ecliptic at about 1° per day and requires about 37 days to cross through the eclipse zone centered on each node. New Moon occurs every 29 1/2 days and thus guarantees at least one eclipse during each of the Sun's node crossings. The period during which the Sun is near a node is called an eclipse season and there are two eclipse seasons each year.
If the line of nodes were fixed in space, then eclipse seasons would occur six months apart and at the same time each year. Actually, the line of nodes slowly drifts westward at the rate of 19 degrees per year. As a result, eclipse seasons occur every 173.3 days. Two eclipse seasons constitute an eclipse year of 346.6 days. This is 18.6 days short of a solar year and is equal to the time required by the Sun to cross the same node twice. In order to find a periodicity in the mechanics of solar eclipses, we must search for a commensurability between the synodic month and the eclipse year. Fortunately, 19 eclipse years are almost exactly equal to 223 synodic months; they differ by only 11 hours. The coincidence is all the more remarkable when compared to a period known as the anomalistic month. This is the time required for the Moon to pass from perigee to perigee and is approximately 27 1/2 days. The anomalistic month is important because the Moon's geocentric distance is the primary factor determining the annular or total nature of a solar eclipse. As unlikely as it may seem, 239 anomalistic months are also equal to 223 synodic months to within 6 hours. This is the origin of the famous Saros cycle of 6585 1/3 days or 18 years. 11 days and 8 hours. Any two eclipses separated by one Saros cycle share very similar mechanical characteristics. They occur at the same node with the Moon at the same distance from Earth and at the same time of year. Because the Saros does not contain an integral number of days, its biggest drawback is that subsequent eclipses are visible from different parts of the globe. Although the 1/3 day displacement shifts the eclipse path 120° westward with each cycle, the series returns to the same geographic region every 3 Saroses or 56 years and 34 days.
A Saros series doesn't last indefinitely because the various periods are not perfectly commensurate with one another. In particular, 19 eclipse years are 1/2 day longer than the Saros. As a result, the node shifts eastward by about 0.5° with each cycle. A typical Saros series begins when new Moon occurs about 18 degrees east of a node. If the first eclipse occurs at the Moon's decending node, the Moon's umbral shadow will pass 3500 km below the Earth and a partial eclipse will be visible from the south polar region. On the following return, the umbra will pass about 300 km closer to the Earth and a partial eclipse of slightly larger magnitude will result. After ten or eleven Saros cycles (about 200 years), the first central eclipse will occur near the south pole of the Earth. Over the course of the next 950 years, a central eclipse will occur at each Saros but will be displaced northward by an average of 300 km. Halfway through this period, eclipses of long duration will occur near the equator. The last central eclipse of the series will occur near the north pole. The next ten eclipses will be partial with successively smaller magnitudes. Finally, the Saros series will end some 13 centuries after it began at the opposite pole. A typical series may be comprised of 70 to 80 eclipses, about 50 of which are central.
If a Saros series begins near the ascending node, the first eclipse will be partial from the northern polar region and the previous sequence of events is reversed. Since at least two solar eclipses occur every year, there are obviously many different Saros series in progress simultaneously. For instance, during the later half of the twentieth century, there are 41 individual series and 26 of them are producing central eclipses. As old series terminate, new ones are always beginning and take their places. To illustrate, the total solar eclipses of 1925, 1943. 1961, 1979, 1997, 2015 and 2033 are all members of Saros 120. The series began with a partial eclipse at the south pole in 915 AD. The 2033 event is the last central eclipse of the series. Note that the paths of the last four eclipses grow progressively broader as the umbral shadow cone passes closer to the limb of the Earth. The next eclipse in the series will be a partial eclipse in 2051. Saros 120 will end with a partial eclipse near the north pole in 2195.
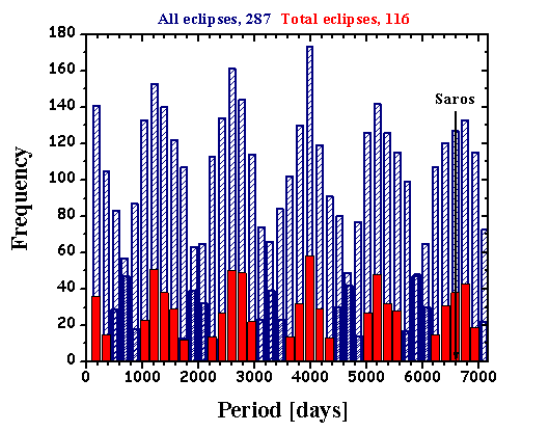
[https://www.researchgate.net/figure/Periods-of-a-repetition-of-solar-eclipses-calculated-from-model-data-The-red-color-is_fig4_258488563](https://www.researchgate.net/figure/Periods-of-a-repetition-of-solar-eclipses-calculated-from-model-data-The-red-color-is_fig4_258488563)
The fascinating math behind solar eclipses and the Saros cycle
[https://www.youtube.com/watch?v=hNvpVB4Ians](https://www.youtube.com/watch?v=hNvpVB4Ians)
The cycles do not coincide exactly. Calculating the misfit of the coincidences (year 2000):
|27.212221 d _242 = 6,585.357 d
29.530589 d_ 223 = 6,585.321 d
6,585.357 d - 6,585.322 d = 0.036 d
0.035 _360° / 27.212 = **0.478°**|29.5305889 d_ 358 = 10,571.951 d
27.2122208 d _388.5 = 10,571.948 d
10,571.951 d - 10,571.948 d = 0.003 d
0.003_ 360° / 27.212 = **0.040°**|
Assuming that a solar eclipse is still possible at a distance of 18.4° from a node ([example](http://www.jgiesen.de/saros/eclipse1935.html)), the number of saros periods in a series would be
2*18.4° / 0.478° = 77
In fact, each saros series lasts 12 to 15 centuries, and produces 69 to 86 eclipses, separated by one saros period (6585.32 days).
Inex series have a longer lifetime than saros series, because the shift of the moon with respect to the node of its orbit is much smaller for the inex (0.040° per cycle) than for the saros (0.478° per cycle), and the inex period (29 years) is longer than the saros (18 years).
Assuming again that a solar eclipse is still possible at a distance of 18.4° from a node, the number of inex periods in a series would be
2_18.4° / 0.040° = 920
which is a lifetime of 920_29 years = 26,680 years.
As Meeus ([Mathematical Astronomy Morsels III](http://www.jgiesen.de/saros/index.html#sources)) is pointing out, the lengths of the synodic, draconic and anomalistic months are subject to long time alterations, and the effective lifetime of an inex series cannot be calculated in this simple way.
A saros-inex panorama has been produced by Luca Quaglia and John Tilley in the form of a Microsoft Excel file [http://eclipse.gsfc.nasa.gov/SEsaros/SEpanorama.xls](http://eclipse.gsfc.nasa.gov/SEsaros/SEpanorama.xls). It shows 61775 solar eclipses from -11000 to +15000 organized by saros series (columns, -290 to 620) and inex series (rows, 60 to 98). The panorama is supplied on four worksheets. These can be printed and glued together to form a large display. Each cell of the spread sheet represents an eclipse of the Sun:
||
|---|
|The saros series 145 starts on 1639 Jan 4, and ends on 3009 Apr 17, consisting of 77. The series 146 starts on 1541 Sep 19, and ends on 2893 Dec 29, consisting of 80 eclipses.|
Type of eclipse: Partial (P), Annular (A), Total (T), annular/total (AT)
[Open: image-20240307010517965.png](https://publish.obsidian.md/shanesql/Attachments/5d087f9243731aeb2e315ed20c27304a_MD5.jpeg)
[Open: image-20240307010523218.png](https://publish.obsidian.md/shanesql/Attachments/5d79d29f30ca25cafeeff5042b2bcf22_MD5.jpeg)
[Open: image-20240307010526697.png](https://publish.obsidian.md/shanesql/Attachments/d1483fdff6d7c63e46c2cc79d03cad30_MD5.jpeg)
Van den Bergh (1955) found that the interval T of time between two solar (or lunar) eclipses can be established from the simple formula:
T = m_inex + n_saros
inex = 358 synodic months (10571.95 days)
saros = 223 synodic months (6585.32 day, 18 years and 10,11, or 12 days,and 8 hours )
m and n are integral numbers (zero, negative or positive).
The mean difference of time calculated between subsequent inex numbers
for saros 145 is 6,585.333 days,
for saros 146 is 6,585.336 days
expected value: 6,585.32 days
The mean interval of time calculated between the saros series 145 and 146 (at equal inex numbers)
is 10,572.32 days (about 28 years and 11 months)
(10,571.95 days expected)
The results do agree very well !
The Example shows a small part of the saros-inex panorama. Saros 145 and inex 38 is the total eclipse of 1999, Aug 11 (greatest magnitude at 11:03 UT, 45.1N 24.3E, visible in Europe):
[Open: image-20240307010826478.png](https://publish.obsidian.md/shanesql/Attachments/8b474c3931f65c0b32ebcfae218d5fe6_MD5.jpeg)
[Open: image-20240307010353662.png](https://publish.obsidian.md/shanesql/Attachments/8fad46258033c4d37bd2148863ffea4e_MD5.jpeg)
[Open: image-20240307010357915.png](https://publish.obsidian.md/shanesql/Attachments/0cb7d6666431478a576b3013945db6e4_MD5.jpeg)
0cb7d6666431478a576b3013945db6e4_MD5.jpeg
[Open: image-20240307010403301.png](https://publish.obsidian.md/shanesql/Attachments/0cb7d6666431478a576b3013945db6e4_MD5.jpeg)
0cb7d6666431478a576b3013945db6e4_MD5.jpeg
The Example shows a small part of the saros-inex panorama. Saros 145, inex 38 is the **total eclipse of 1999**, Aug 11 (greatest magnitude at 11:03 UT, 45.1N 24.3E, visible in Europe
[Open: image-20240307010411601.png](https://publish.obsidian.md/shanesql/Attachments/034bf9b71e4208d70c080d2cfdea9b55_MD5.jpeg)
To get to the next eclipse (in time) of 2000 Feb 05 (which is partial) we have to move 5 columns to the right and 8 rows upward:
5 inexes - 8 saroses = 5_358 - 8_223 = 6 lunations
[Open: image-20240307010716669.png](https://publish.obsidian.md/shanesql/Attachments/60067e133af5740b7a2580f652f62bad_MD5.jpeg)
60067e133af5740b7a2580f652f62bad_MD5.jpeg
[https://upload.wikimedia.org/wikipedia/commons/6/66/Tritos_saros_lunar_series_1000-2500.png](https://upload.wikimedia.org/wikipedia/commons/6/66/Tritos_saros_lunar_series_1000-2500.png)
The time interval "t" between any two solar eclipses can be found through an integer combination of Saros and Inex periods via the following relationship:
The direct application of the Saros-Inex panorama allows for the determination of eclipse dates in the past (or future); however, the application of the longer Saros-Inex combinations permit the rapid estimation of a number of eclipse characteristics without lengthy calculations. Table 6 lists several of the most useful periods.
The numbering system used for the Saros series was introduced by van den Bergh in his book _Periodicity and Variation of Solar (and Lunar) Eclipses_ (1955). He assigned the number 1 to a pair of solar and lunar eclipse series that were in progress during the second millennium BCE based on an extrapolation
from von Oppolzer's _Canon der Finsternisse_ (1887). - [https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section108](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section108)
### Soros and Cycles
The Saros cycle
- Strictly speaking, the name _Saros_ for this eclipse cycle is a misnomer as it was derived from an ancient Babylonians term to indicate the number 3600 (_šār_)
- The name was first coined in 1691 by the English astronomer Edmond Halley, who extracted it from a 10th-century Byzantine lexicon (q.v. Σάροι in Suda Σ 148) which identified it as a 222-month Babylonian cycle
- Ancient Babylonian texts simply referred to this cycle as the “18-Year Cycle” while Ptolemy of Alexandria (Almagest IV.2) referred to it as the περιοδικὸϲ χρόνοϲ (“Periodic Interval”
## How do you use the Saros in interpretting an Eclipse?
[https://eclipse.gsfc.nasa.gov/SEhelp/moonorbit.html](https://eclipse.gsfc.nasa.gov/SEhelp/moonorbit.html)
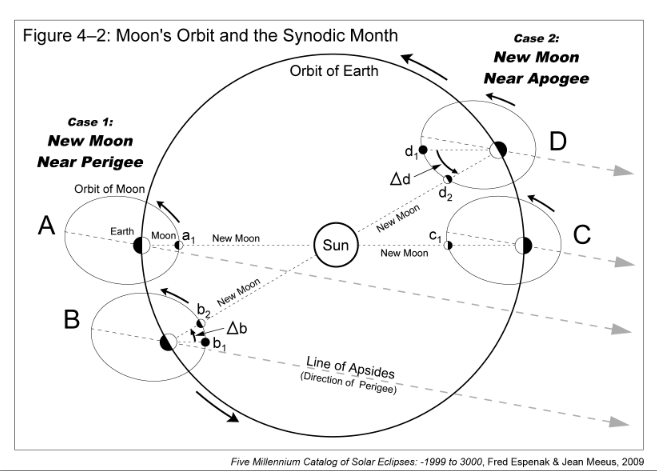
Most astrologers are used to thinking of individual eclipses as isolated events with no connections to other eclipses (except by aspect). We analyze each eclipse chart by itself and it never occurs to us to put each eclipse energy in a particular context.
However, the Babylonian astrologers found it was very important to study an eclipse in the context of the Saros Family that contains it. The entire cycle has a "personality" all its own that colors each individual chart. This Saros personality is based on the first eclipse in the Saros Family (a birthchart for the entire cycle, so to speak) and each eclipse in the Family is helping to advance this initial chart to the next level. This may help explain why all eclipses are not created equal -- there are about 80 different Families in action, each with a different pedigree.
It would be interesting to look at the chart for the last eclipse in a Saros Cycle as a way to determine the final outcome of the eclipses happening now. In astrology, future events sometimes reach back in time to the present in order to mold events to a future outcome. It's important to know where you're headed, not just where you came from. I suspect other eclipses that mark changes in the eclipse geometry, especially the first and last total eclipse in the cycle, may also shed some interesting light.
Finally, when looking at an upcoming eclipse, check out its Saros buddy from 18+ years ago. Family members in a Saros are connected and carry some of the same energy threads. Checking your "Saros return" every 18 years can provide illuminating information about long term cycles in your life.
You can study eclipses both as transits (the current eclipses and how they affect us) and natally as prenatal eclipses. These are described next.
Identifying the parts of various astronomical cycles such as the Saros cycle, lunar cycle, solar cycle, Metonic cycle, and the Inex involves understanding their duration, significance, and the way they interplay in both astronomical phenomena and historical timing. Here's a simplified overview of the process for each cycle:
Figure 4-9 plots the duration of the draconic month minus its mean value for 2008 through 2010. The shortest month over this 3-year period is 27.05115 days (27d 01h 14m), while the longest month is 27.38409 days (27d 09h 13m).
The most significant characteristic of this variation is that it is synchronized with the ascending node relative to the Sun's position along the ecliptic. The mean angle between the Sun and the ascending node (i.e., difference in mean longitude) is also plotted in Figure 4-9 (diagonal lines) to illustrate this relationship. The longitude difference at the start of each draconic month is plotted as a black dot. Longitude values can be read using the scale along the right side of the figure. The longest draconic months occur when the difference in the mean longitudes of the Sun and the ascending node is either 0° or 180°. In contrast, the shortest months occur when the angle between the Sun and the ascending node is either 90° or 270°.
The mean draconic month is 0.10944 day (2h 36m 36s) shorter than the sidereal month. Consequently, the lunar nodes slowly rotate west or retrograde (opposite the Moon's orbital motion) along the ecliptic at a rate of 0.05295° per day. One complete rotation of the ascending node about the ecliptic requires 18.6 years (6793.48 days) with respect to the fixed stars.
The length of the draconic month is strongly modulated by the position of the nodes with respect to the major axis of the Moon's orbit. The histogram in Figure 4-11 shows how the draconic month changes from 2008 through 2017. The 173-day alignment of the Sun with a node appears as the rapid oscillation in the month length. The quasi-sinusoidal envelopes surrounding the minima and maxima form two longer period oscillations. Over the 10-year period covered in this figure, the minimum month duration varies from 27.089 to 27.011 days (3.0 to 4.8 hours shorter than the mean). The maximum month duration ranges from 27.261 to 27.472 days (1.2 to 6.2 hours longer than the mean).
The difference in the mean longitudes of perigee and the ascending node appear as diagonal lines in Figure 4-11. This is the angle between these orbital parameters measured along the ecliptic. The greatest extremes in the draconic month occur when the angle between perigee and the ascending node is 0°. Likewise, the smallest extremes of the month take place when the difference in longitude is 180°. The mean rates of the major axis and the ascending node are 0.11140° east and 0.05295° west per day, respectively. Therefore, the mean period between alignments of the axis and node is 2190.4 days or 6.0 years. This period is clearly seen in Figure 4-11.
There are 67,111 draconic months during the 5000 years covered in this catalog. The shortest and longest months are 27.004 days (0.208 days or 5.0 hours shorter than the mean) and 27.487 days (0.275 days or 6.6 hours longer than the mean), respectively. A histogram of the distribution in the length of the draconic month over the five millennia appears in Figure 4-12 where the duration of individual draconic months have been binned into 30-min groups. The width and bifurcated symmetry of the distribution resemble the distribution for the lunation (synodic month) in Figure 4-4.
# Eclipse Attributes Repeat with the Soros Cycle
#### Eclipses as Transits
When you are studying how an eclipse will affect you, you set up a chart for the date and time of the middle of the eclipse (which may differ from the time of the new or full moon by a few minutes, curiously) for the location where you live. If you are looking at another place on the earth's surface, use that location instead. For instance, to see "How will the eclipse affect the US?", you would probably want to set up the chart for Washington, DC. You are looking for close connections between the eclipse chart and your own birthchart, such as close aspects from the eclipse to your chart. The more connections, the stronger and more broad-based the influence is for you. Also pay attention to which natal house contains the eclipse degree. The affairs of that house will likely be up for review and overhaul the next few months.
However, set up a second chart for the eclipse that started the Saros series that contains the current eclipse you are studying. I'd advise using where you live as the place for this chart. (Remember that the initial and final eclipses in a Saros series fall near the north or south pole, where almost all house systems fall apart -- it's nearly impossible to draw "the chart" for such an event.) This second chart describes the energies of the entire Saros, of which the current eclipse is just a member. It's the background energy that colors the current situation, much like the actions of the slower moving planets provide a background for the other transits. This background energy of the Saros chart will also make connections to your own birthchart which you can study. In particular, the house containing this initial eclipse point will probably have some deeper connection affecting the house containing the current eclipse.
As a practical matter when studying these eclipse charts, it's best to stick to the "major" aspects, namely conjunction, square and opposition. Trines and sextiles are perhaps important, though many astrologers would ignore them in this context. Also, it's best to use tight orbs for these aspects, perhaps as little as 2 to 5 degrees.
[Transits of Venus](https://publish.obsidian.md/shanesql/assets/Database+Tabs/Transits+of+Venus)
Equivalence ##
Soros cycle is equal to :
- 239 anomalistic months (to within a couple hours)
- 242 draconic months (to within a couple hours)
- 6,585.321347 solar days
- 18.029 years
- 223 synodic months
- 241.999 draconic months
- 18.999 eclipse years (38 eclipse seasons)
- 238.992 anomalistic months
- 241.029 sidereal months
Inex= 358 synodic months (10571.95 days)
Saros = 223 synodic months (6585.32 days, 18 years and 10 or 11 days and 8 hours )
m and n are integral numbers (zero, negative or positive).
The interaction and harmonics of the synodic, anomalistic, and draconic months not only determine how frequently eclipses occur, but they also control the geometric characteristics and classification of each eclipse. The commensurability of these periods over long time scales results in several important eclipse cycles, which are discussed in [Periodicity of Solar Eclipses](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html) and [Periodicity of Lunar Eclipses](https://eclipsewise.com/lunar/LEhelp/LEperiodicity.html).
### 1.3 Solar Eclipse Repetition


A solar eclipse will be **total** if the new Moon is at **perigee** (closest to the Earth, making it look larger) and the sun is at apogee (farthest from the Earth, making it look smaller, around January 3)
[**Diagram showing the apogees of the Moon**](http://www.jgiesen.de/saros/apogee.html)
Another way to represent:
[https://t.me/c/1614757092/84830](https://t.me/c/1614757092/84830)
Eclipses separated by 1, 5 or 6 lunations are usually quite dissimilar. They are often of unlike types (i.e., partial, annular, total or hybrid) with diverse Sun-Moon-Earth alignment geometries, and with different lunar orbital characteristics (i.e., longitude of perigee and longitude of ascending node). More importantly, these short periods are of no value as predictors of future eclipses because they do not repeat in any recognizable pattern.
A simple eclipse repetition cycle can be found by requiring that certain orbital parameters be repeated. The Moon must be in the new phase with the same longitude of perigee and same longitude of the ascending node. These conditions are met by searching for an integral multiple in the Moon's three major periods-the synodic, anomalistic and draconic months. A fourth condition might require that an eclipse occur at approximately the same time of year to preserve the axial tilt of Earth and thus, the same season, as well as the distance from the Sun.
Two eclipses separated by one Saros cycle share very similar characteristics. They occur with the Moon at nearly the same distance from Earth and at the same time of year. They also occur at the same node with the Moon at nearly the same distance from Earth and the same time of year due to a harmonic in three cycles of the Moon's orbit . [https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section102](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#section102)
The main drawback of the cycles lies in the fact that after each eclipse the time of maximum obscuration is shifted by nearly 8 hours so that successive eclipses are about 120° apart in longitude and thus often not visible from a fixed position on Earth. This means The Sky must rotate above the earth an additional ~8 hours or ~120º with each cycle. (15° an hour times 8 hours is 120° of movement on the coordinate system of the celestial). For solar eclipses, this results in the shifting of each successive eclipse path by ~120º westward. Completing tis circle on map you can conclude Saros series returns to approximately the same geographic region every 3 Saros Cycles (54 years and 34 days). [Saros Inex Panorma](https://publish.obsidian.md/shanesql/Eclipses#Saros%20Inex%20Panorma)
This extra 8 hours is exactly the reason the cycles are not easy and straight forward to map.
This ensures that the next cycle will not exactly overlap with the previous one, but be offset by that 8 hours, or 1/3 of a day. This is the reason for the second cycle within the greater cycle.
The total duration of a 651 year astronomical year describes the time it takes for this series of repeating eclipses to catch up with itself again, by appearing 8 hours later each time. You can see this starts to add up and explains the visualization from before. [Eclipses](https://publish.obsidian.md/shanesql/Attachments/LibraryBackup/Eclipse+Backup#Saros%20Inex%20Panorma)
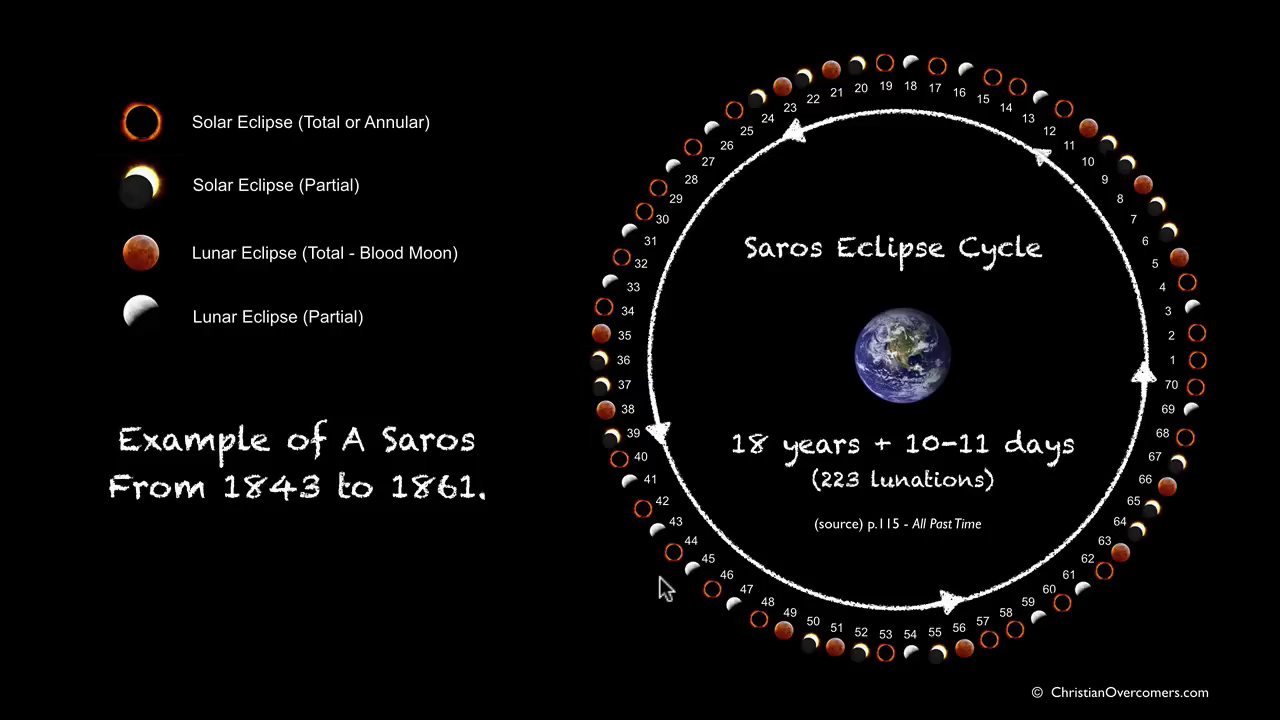
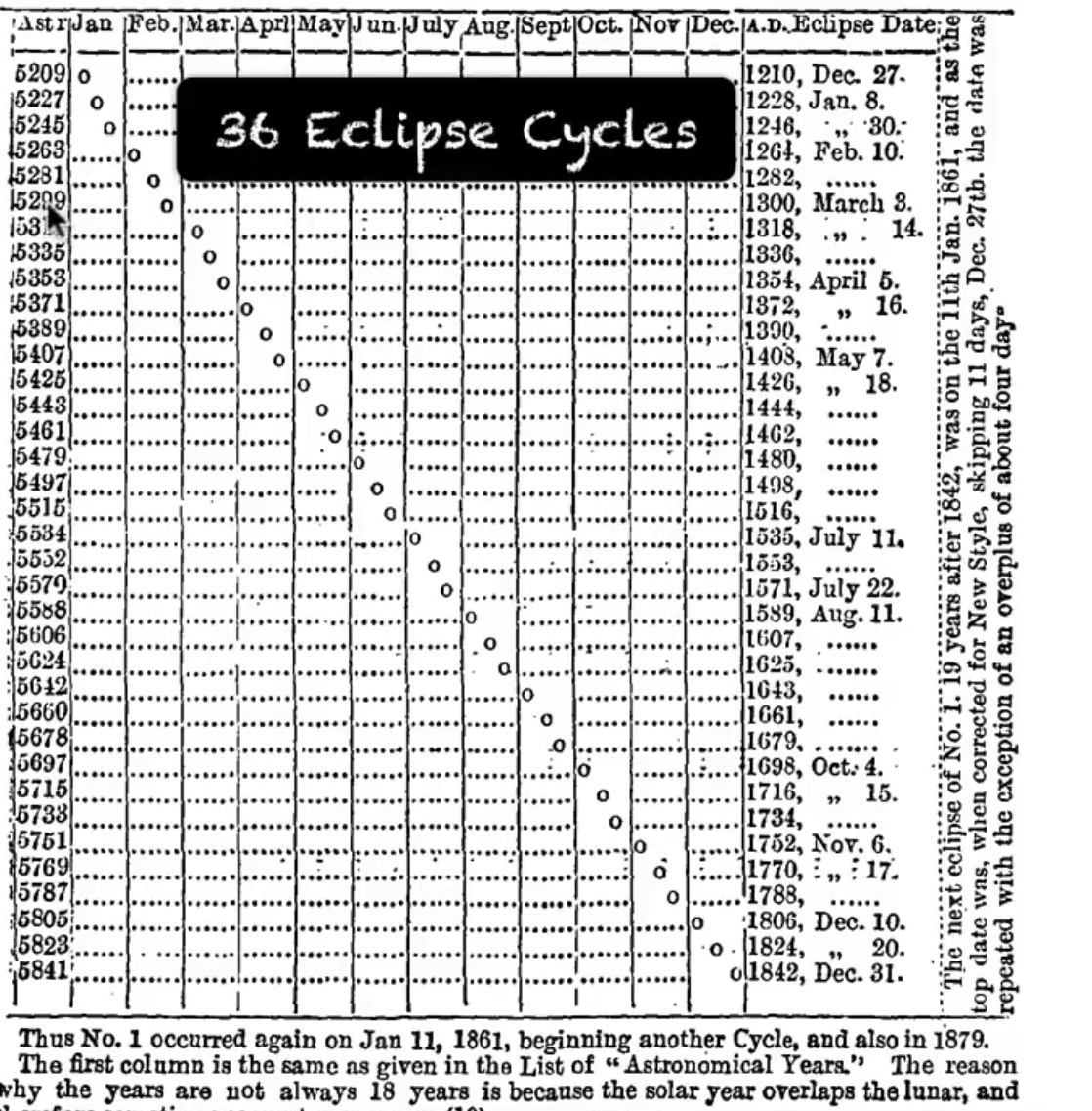
(These are all central eclipses)
Some examples of the Cyclical Nature of the attributes of Eclipses. After a given lunar or solar eclipse, after 9 years and 5+1⁄2 days (a half saros, or sar) an eclipse will occur that is lunar instead of solar, or vice versa, with similar properties. For example, if the Moon's penumbra partially covers the southern limb of the Earth during a solar eclipse, 9 years and 5+1⁄2 days later a lunar eclipse will occur in which the Moon is partially covered by the southern limb of the Earth's penumbra. Likewise, 9 years and 5+1⁄2 days after a total solar eclipse or an annular solar eclipse occurs, a total lunar eclipse will also occur. This 9-year period is referred to as a **sar**.
An exeligmos (Greek: ἐξελιγμός — turning of the wheel) is a period of 54 years, 33 days that can be used to predict successive eclipses with similar properties and location. For a solar eclipse, after every exeligmos a solar eclipse of similar characteristics will occur in a location close to the eclipse before it. For a lunar eclipse the same part of the earth will view an eclipse that is very similar to the one that occurred one exeligmos before it (see main text for visual examples). The exeligmos is an eclipse cycle that is a triple saros, three saroses (or saroi) long, with the advantage that it has nearly an integer number of days so the next eclipse will be visible at locations and times near the eclipse that occurred one exeligmos earlier. In contrast, each saros, an eclipse occurs about eight hours later in the day or about 120° to the west of the eclipse that occurred one saros earlier.
Thus, the Saros is useful for organizing eclipses into families or series. Each series typically spans hundreds of years and contains 70 or more eclipses. Every saros series begins with a number of partial eclipses near one of Earth's polar regions. The series will then produce several dozen central eclipses before ending with a group of partial eclipses near the opposite pole.
- Source [https://eclipsewise.com/solar/SEhelp/SEpanorama.html](https://eclipsewise.com/solar/SEhelp/SEpanorama.html) _For more information, see [Periodicity of Solar Eclipses](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html)._
- S_ource [https://webspace.science.uu.nl/~gent0113/eclipse/eclipsecycles.htm]_
The [Saros](https://eclipsewise.com/solar/SEhelp/SEsaros.html) arises from a harmonic between three of the Moon's orbital cycles. All three periods are subject to slow variations over long time scales, but their current values (2000 CE) are:
```
Synodic Month (New Moon to New Moon) = 29.530589 days = 29d 12h 44m 03s
Anomalistic Month (perigee to perigee) = 27.554550 days = 27d 13h 18m 33s
Draconic Month (node to node) = 27.212221 days = 27d 05h 05m 36s
```
One Saros is equal to 223 synodic months, however, 239 anomalistic months and 242 draconic months are also equal (within a few hours) to this same period:
```
223 Synodic Months = 6585.3223 days = 6585d 07h 43m
239 Anomalistic Months = 6585.5375 days = 6585d 12h 54m
242 Draconic Months = 6585.3575 days = 6585d 08h 35m
```
Lunar periodicities refer to the recurring cycles of the Moon's motion relative to different reference points in space. These periodicities have profound implications for predicting and interpreting celestial events such as eclipses. Understanding these cycles provides insights into the mechanics of the Earth-Moon system and aids astronomers in forecasting astronomical phenomena.
Sidereal Month:
The sidereal month is defined as the time it takes for the Moon to complete one orbit around the Earth relative to the distant stars. It has a duration of approximately 27.321662 days. This period is crucial for calculating the Moon's position in the sky with respect to the fixed background of stars.
Synodic Month:
The synodic month is defined as the time between successive occurrences of the same lunar phase, such as from one new moon to the next. It is approximately 29.530589 days long and is measured relative to the Sun. This period is essential for understanding the phases of the Moon and predicting lunar and solar eclipses.
Tropical Month:
The tropical month is the time it takes for the Moon to return to the same position relative to the vernal equinox. It is slightly shorter than the sidereal month due to the precession of the Earth's axis, with a duration of approximately 27.321582 days. This periodicity is significant in studies related to the Earth's axial tilt and its effect on celestial observations.
Anomalistic Month:
The anomalistic month is defined as the time between successive passages of the Moon through perigee, the point in its orbit closest to Earth. It lasts approximately 27.554550 days and is essential for predicting variations in the Moon's apparent size and brightness during its orbit.
Draconic Month:
The draconic month is the time it takes for the Moon to return to the same position relative to its ascending node, the point where it crosses the plane of the Earth's orbit around the Sun. It has a duration of approximately 27.212221 days and is crucial for predicting lunar nodes and eclipses.
With a period of approximately 6,585.32 days (~18 years 11 days 8 hours), the Saros is valuable tool in investigating the periodicity and recurrence of eclipses. It was first known to the Chaldeans as an interval when lunar eclipses repeat, but the Saros is applicable to solar eclipses as well.
[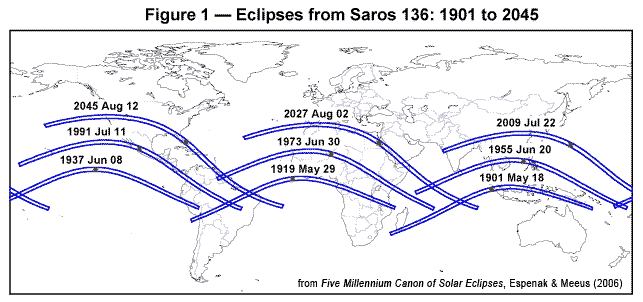](https://eclipsewise.com/solar/SEhelp/images/Fig1b.gif)
#### 1.2 Interval Between Two Successive Solar Eclipses
The time interval between any two successive solar eclipses can be either 1, 5, or 6 lunations (synodic months).
Earth will experience 11,898 eclipses of the Sun during the 5000-year period from -1999 to +3000 (2000 BCE to 3000 CE [2](https://publish.obsidian.md/shanesql/2)([https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#fn-2](https://eclipsewise.com/solar/SEhelp/SEperiodicity.html#fn-2))). As published in in both the _[Five Millennium Canon of Solar Eclipses: -1999 to +3000](https://eclipsewise.com/pubs/5MCSE2.html)_ and _[Five Millennium Catalog of Solar Eclipses: -1999 to +3000](https://eclipsewise.com/pubs/5MKSE2.html)_, the distribution of the 11,897 intervals between these eclipses is found in Table 1
## The Moon's Two Shadows
An eclipse of the Sun (or solar eclipse) can _only_ occur at New Moon when the Moon passes between Earth and Sun. If the Moon's shadow happens to fall upon Earth's surface at that time, we see some portion of the Sun's disk covered or 'eclipsed' by the Moon. Since New Moon occurs every 29 1/2 days, you might think that we should have a solar eclipse about once a month. Unfortunately, this doesn't happen because the Moon's orbit around Earth is tilted 5 degrees to Earth's orbit around the Sun. As a result, the Moon's shadow usually misses Earth as it passes above or below our planet at New Moon. At least twice a year, the geometry lines up just right so that some part of the Moon's shadow falls on Earth's surface and an eclipse of the Sun is seen from that region.
The Moon's shadow actually has two parts:
### 1. Penumbra
- The Moon's faint outer shadow.
- Partial solar eclipses are visible from within the penumbral shadow.
### 2. Umbra
- The Moon's dark inner shadow.
- Total solar eclipses are visible from within the umbral shadow.##
## 1.5 Gamma and Saros Series
Gamma changes monotonically throughout any single Saros series. The change in gamma is larger when Earth is near its aphelion (June to July) than when it is near perihelion (December to January). For odd numbered series (ascending node), gamma decreases, while for even numbered series (descending node), gamma increases. This simple rule describes the current behavior of gamma, but this has not always been the case. The eccentricity of Earth's orbit is presently 0.0167, and is slowly decreasing. It was 0.0181 in the year -2000 and will be 0.0163 in +3000. In the past when the eccentricity was larger, there were Saros series in which the trend in gamma reversed for a few cycles before resuming its original direction. These instances occur near perihelion when the Sun's apparent motion is highest and may, in fact, overtake the eastward shift of the node. The resulting effect is a relative shift west of the node after one Saros cycle instead of the usual eastward shift. Consequently, gamma reverses direction.
The most unusual case of this occurs in Saros series 0. It began in -2955 with 11 partial eclipses, followed by 1 total, 1 hybrid, and 4 annulars. Gamma increased with each eclipse until it reversed direction with the second annular. It continued to decrease and the series began to once again produce partial eclipses. With the third partial eclipse, gamma resumed its original northward shift. The series went on to produce 45 more annular eclipses before ending in the year -1675 after 7 partial eclipses.
Among several hundred Saros series examined (-34 to 247), there are many other examples of temporary shifts in the monotonic nature of gamma, although none as bizarre as Saros 0. Some series have two separate reversals in gamma (e.g., series 15, 34, and 52) or even three (e.g., series -5 and 13). The most recent eclipse with a gamma reversal was in 1674 (Saros 107). The next and last in the _[Five Millennium Canon](https://eclipsewise.com/pubs/5MCSE2.html)_ and _[Five Millennium Catalog](https://eclipsewise.com/pubs/5MKSE2.html)_ will occur in 2290 (Saros 165). In past millennia, the gamma reversals were more frequent because Earth's orbital eccentricity was larger.
## Eclipse Source Applets and Programs
[http://www.geoastro.de/planets/](http://www.geoastro.de/planets/)
[https://www.researchgate.net/publication/378229923_Eclipses_A_Brief_History_of_Celestial_Mechanics_Astrometry_and_Astrophysics](https://www.researchgate.net/publication/378229923_Eclipses_A_Brief_History_of_Celestial_Mechanics_Astrometry_and_Astrophysics)
The fascinating math behind solar eclipses and the Saros cycle
[https://www.youtube.com/watch?v=hNvpVB4Ians](https://www.youtube.com/watch?v=hNvpVB4Ians)
Position of the Sun by Spreadsheet
[http://www.geoastro.de/sunpos/day.html#download](http://www.geoastro.de/sunpos/day.html#download)
Movement of a star
[https://www.walter-fendt.de/html5/aen/starposition_en.htm](https://www.walter-fendt.de/html5/aen/starposition_en.htm)
Solar Calculation Details
[https://gml.noaa.gov/grad/solcalc/calcdetails.html](https://gml.noaa.gov/grad/solcalc/calcdetails.html)
Sphere view
[http://www.jgiesen.de/planets/index.html](http://www.jgiesen.de/planets/index.html)
List of software
[http://www.jgiesen.de/GeoAstro/GeoAstro.htm](http://www.jgiesen.de/GeoAstro/GeoAstro.htm)
Heliocentric and geocentric motion of the bright planets
[http://www.jgiesen.de/geocentric/index.html](http://www.jgiesen.de/geocentric/index.html)
Astro applets:
[http://www.jgiesen.de/astro/index.htm](http://www.jgiesen.de/astro/index.htm)
Basics
[http://www.jgiesen.de/elevazmoon/basics/index.htm](http://www.jgiesen.de/elevazmoon/basics/index.htm)
astronomy,Java,applet,sun,earth,moon,day,night,azimuth,elevation,sunrise,sunset,altitude,horizon,GHA,declinationh,moon,day,night,azimuth,elevation,sunrise,sunset,altitude,horizon,GHA,declination
[http://jgiesen.de/SME/](http://jgiesen.de/SME/)
JavaScript computing the Julian Day,
the Declination of the Sun, the Equation of Time, and the Length of Day
[http://www.jgiesen.de/astro/astroJS/decEoT/index.htm](http://www.jgiesen.de/astro/astroJS/decEoT/index.htm)
Polar moonrise and sunrise anywhere o earth
[http://www.jgiesen.de/sunmoonpolar/](http://www.jgiesen.de/sunmoonpolar/)
Sky Map Applet
showing the bright stars and planets (Mercury, Venus, Mars, Jupiter, Saturn)
[http://www.jgiesen.de/skymap/index.html](http://www.jgiesen.de/skymap/index.html)
Analemma Sundial Applet
[http://www.jgiesen.de/analemma/index.htm](http://www.jgiesen.de/analemma/index.htm)
Day & Night Applet
[http://www.jgiesen.de/TNApplet/DN/index.html](http://www.jgiesen.de/TNApplet/DN/index.html)
Eclipse Magnitude and Obscuration
[http://www.jgiesen.de/eclipse/index.html](http://www.jgiesen.de/eclipse/index.html)
The Total Solar Eclipse of 1999 August 11 in Europe.
[http://www.jgiesen.de/SME/eclipse99/index.htm](http://www.jgiesen.de/SME/eclipse99/index.htm)
astronomy,Java,applet,sun,earth,moon,day,night,azimuth,elevation,sunrise,sunset,altitude,horizon,GHA,declination
[http://jgiesen.de/SME/](http://jgiesen.de/SME/)
Geocentric RetroGrade [http://www.jgiesen.de/geocentric/index.html](http://www.jgiesen.de/geocentric/index.html)
Geocentric motion of the bright planets
[http://www.jgiesen.de/geocentric/motion.html](http://www.jgiesen.de/geocentric/motion.html)
## Total solar eclipses over the 5000
A heatmap showing the frequency of total solar eclipses over the 5000 years from 2000 BCE to 3000 CE. Includes versions without the color key and without the continent outlines.
This is a heatmap showing the density of solar eclipse paths over the Earth during the 5000-year period between 2000 BCE and 3000 CE. It uses the list of eclipses calculated by Fred Espenak and Jean Meeus and published in 2006 as the Five Millennium Canon of Solar Eclipses. The paths of the 3742 eclipses classified in the Canon as either T (total) or H (hybrid or total-annular) were drawn into a global map with a pixel resolution of 4 minutes (1/15 of a degree) of latitude and longitude. The pixels counted the eclipse paths as they were drawn, and each pixel location ended up experiencing anywhere from one to 35 eclipses.
It's tempting to make statements about particular locations based on the map, but that wouldn't be valid. Because the rotation speed of the Earth isn't perfectly uniform, the longitudes of the paths are increasingly uncertain for times farther in the future and the distant past. Rather than trying to estimate the longitude corrections, the map (and the Canon) use Terrestrial Time, a uniform astronomical timescale that ignores the variable rotation of the Earth. Thus, the latitude and shape of each path is true, while the longitude is only accurate on a fictitious Earth that turns with the perfection of an atomic clock at sea level.
What we _can_ do is draw a number of statistical inferences about the geographical distribution of total eclipses. For example, more total eclipses happen in the northern hemisphere than in the southern, which is mostly the result of the Earth's slightly lopsided elliptical orbit around the Sun. Eclipses are more likely in the summer, just because the Sun is up longer then. Summer in the northern hemisphere happens when the Earth is near aphelion, its farthest distance from the Sun for the year, and this makes the Sun a bit smaller in the sky, giving the Moon a better chance of covering it completely. This latitude bias is shown clearly in the graph below.
It's evident from the heatmap that a total solar eclipse can happen absolutely anywhere on Earth. In fact, there isn't a single pixel in the map that isn't visited by at least one eclipse – not a single goose egg in any of the 14.6 million points sampled by the map.
The map can also be used to estimate, on average, the time between two total eclipses at particular places. Across the entire map, the average number of eclipses seen by a pixel is 13.66. Dividing 5000 years by this figure produces an average of 366 years between eclipses. This number is almost exactly halfway between the estimates of 360 by Russel et al. in their 1926 astronomy textbook and 375 by Jean Meeus in a 1982 paper published in the Journal of the British Astronomical Association.
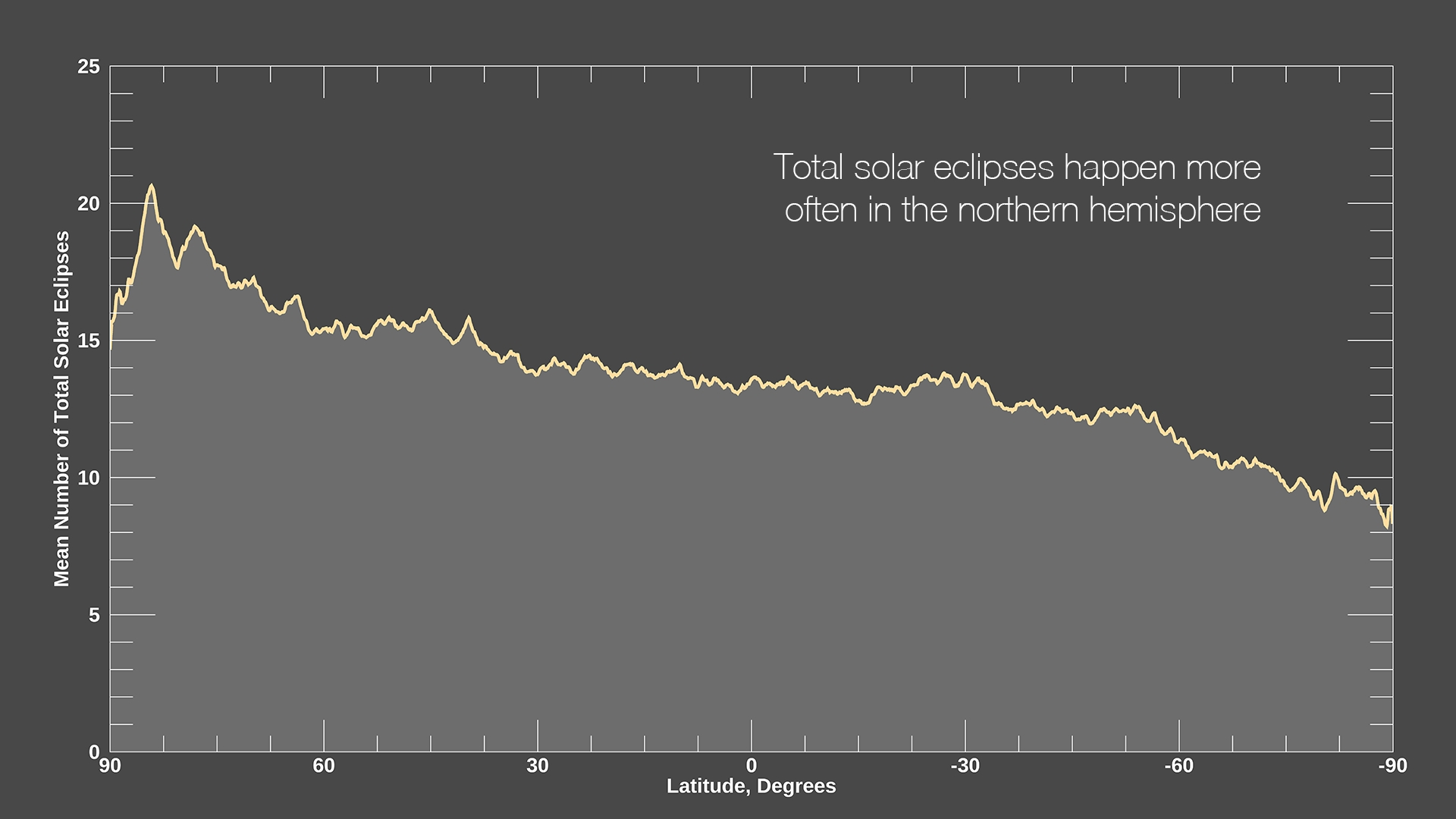
A graph of the average number of total eclipses experienced at each latitude in the eclipse frequency heatmap. The average was calculated for each scanline of the heatmap.
# NO ONE PREDICTS ECLIPSES
All data is derived from the solar and lunar cycle combined into the 651 year great astronomical year. The only thing they could possibly claim is the map which we plot it on. Since all maps are projections of the celestial longitude and latitude, we have to use their map. They use that map to derive the path, using GPS coordinates, and then expand or shrink that path according to a ratio that coincides with the cycle of the moon and the appearance of the umbra.
If you follow the links you end up at this model: Which explicitly bases it on Keplerian ellipses, so we are back at the geocentric start anyway.
However, if you were to aggrgate real data and use that to hypothetically say when another part of that same cycle were to repeat..... well you would use all these.
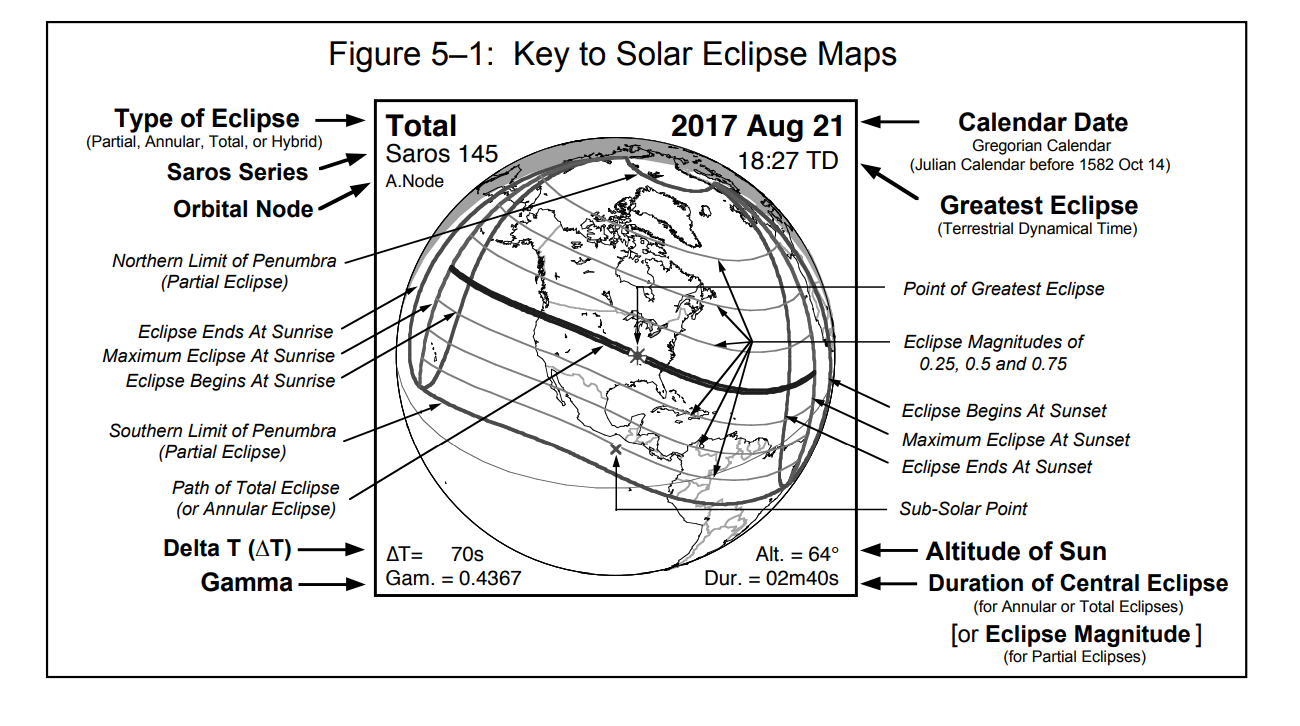
### The 'Shadow'
Do you need the Moon's MASS to predict its path and eclipses? NO. It is all based on optics. Mass deduced by apparent size. Apparent size being reduced to 0.53 Degrees. Let us not forget that the apparent angular size does not mean we know the exact mass of the moon. Remember how covariant scaling works?

[https://eclipse.gsfc.nasa.gov/SEcat5/beselm.html](https://eclipse.gsfc.nasa.gov/SEcat5/beselm.html)
And just to be clear, lets read a note from Fred. He didn't even use the supposed heliocentric ephemeras corrections, as miniscule as they were.
> The center of figure of the Moon does not coincide exactly with its center of mass. The magnitude of this difference is ~0.5 arcsec and is much smaller than the uncertainty in the edges of the penumbral and umbral shadows because of diffusion by Earth’s atmosphere (Sect. 1.5). **Thus, the difference between center-of-figure and center-of-mass positions has no practical impact on the present work** - [https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf](https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf)
Sure, they can change the mass as an indirect factor of the angular resolution of the moon. They do this in a couple different ways, and it predicts slightly different results. In the words of Fred,
> "There is to _right or wrong_ answer in the above calculations. They simply use different assumptions for calculating the duration of totality at a given point and they have different levels of accuracy. "
But how do you predict the size of the shadow?
> In the early 1700s, Philippe de La Hire made a curious observation about Earth’s umbra. The predicted radius of the shadow needed to be enlarged by about 1/41 in order to fit timings made during a lunar eclipse (La Hire 1707). Additional observations over the next two centuries revealed that the shadow enlargement was somewhat variable from one eclipse to the next. According to Chauvenet (1891): “This fractional increase of the breath of the shadow was given by Lambert as 1/40, and by Mayer as 1/60. Beer and Maedler found 1/50 from a number of observations of eclipses of lunar spots in the very favorable eclipse of December 26, 1833.” Chauvenet adopted a value of 1/50, which has become the standard enlargement factor for lunar eclipse predictions published by many national institutes worldwide. The enlargement enters into the definitions of the penumbral and umbral shadow radii as follows.
[https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf](https://eclipse.gsfc.nasa.gov/5MCLE/5MKLE-214173.pdf)
So, the prediction of the enlargement of the shadow is entirely observational based. No
Even though this is mapped on a globe, try not to let it bother you. All Maps are equal...
Remember these circles are equal.
#### Besselian Elements
Besselian Elements: To define the Besselian elements of an eclipse, a plane is passed through the Earth's center, fixed perpendicular to the axis of the Moon's shadow. This plane, called the fundamental plane, has an X-Y rectangular coordinate system constructed with its origin at the geocenter. The axes of this system are oriented with north in the positive Y direction and east in the positive X direction. The Z axis is perpendicular to the fundamental plane and parallel to the shadow axis.
The X-Y coordinates of the shadow axis can now be expressed in units of the Earth's equatorial radius. The radii of the penumbral and umbral shadows on the fundamental plane are identified as L1 and L2, respectively. The direction of the shadow axis on the celestial sphere is defined by its declination 'd' and ephemeris hour angle 'µ'. Finally, the angles which the penumbral and umbral shadow cones make with the shadow axis are expressed as f1 and f2, respectively. These eight parameters and their variations over time serve as the only input needed to characterize a solar eclipse. They may be tabulated at hourly intervals or expressed as a series of third-order polynomials over the several-hour period of the eclipse.
The eight Besselian elements needed to characterize a solar eclipse can be summarized as follows:
- x, y: Cartesian coordinates of the lunar shadow axis in the Fundamental Plane (in units of the Earth's equatorial radius)
- L1, L2: Radii of the Moon's penumbral and umbral/antumbral shadows in the Fundamental Plane (in units of the Earth's equatorial radius)
- d: Declination of the Moon's shadow axis on the celestial sphere
- µ: Hour angle of the Moon's shadow axis on the celestial sphere
- f1, f2: Angles of the penumbral and umbral/antumbral shadow cones with respect to the axis of the lunar shadow
Details for actual eclipse calculations using the Besselian elements can be found in the references listed below.
#### Shadow prediction
[https://www.britannica.com/science/eclipse/Prediction-and-calculation-of-solar-and-lunar-eclipses](https://www.britannica.com/science/eclipse/Prediction-and-calculation-of-solar-and-lunar-eclipses)
The problem may be divided into two parts. The first is to find out when an eclipse will occur, the other to determine when and where it will be visible.
[](https://cdn.britannica.com/31/131-004-C9E4BD5D/motions-sphere-Sun-and-the-Moon.jpg)
[figure illustrating the apparent motions of the Sun and the Moon on the celestial sphere](https://cdn.britannica.com/31/131-004-C9E4BD5D/motions-sphere-Sun-and-the-Moon.jpg)
Apparent motions of the Sun and the Moon on the celestial sphere (_see_ the text).(more)
For this purpose it is convenient first to consider [Earth](https://www.britannica.com/place/Earth) as fixed and to suppose an observer is looking out from its centre. To this observer, labeled O in the figure of the celestial sphere, the [Sun](https://www.britannica.com/place/Sun) and Moon appear projected on the [celestial sphere](https://www.britannica.com/science/celestial-sphere). While this sphere appears to rotate daily, as measured by the positions of the stars, around the [axis](https://www.britannica.com/dictionary/axis) PP′ (Earth’s axis of rotation), the Sun’s disk, S, appears to travel slowly along the great circle EE′ (the ecliptic), making a complete revolution in one year. At the same time, the Moon’s disk, M, travels along its own great-circle path, LL′, once during a lunar month. The angular diameters of the Sun’s and the Moon’s disks, S and M, are each about 0.5° but vary slightly.
Every month, the Moon’s disk moving along its path, LL′, will overtake the more slowly moving Sun once, at the moment of the new moon. Usually the Moon’s disk will pass above or below the Sun’s disk. Overlapping of the two results in an [eclipse](https://www.britannica.com/dictionary/eclipse) of the Sun, which can happen only when the new moon occurs at the same time that the Sun is near the ascending node or descending node, [nodeascnd] and [nodedescd], respectively, of the Moon’s orbit. Because the nodes are 180° apart, eclipses occur in the so-called eclipse seasons, six months apart.
In the figure of the celestial sphere, the projection of Earth’s [umbra](https://www.britannica.com/science/umbra-eclipse) is shown as a disk, U, at the distance of the Moon’s orbit. At that distance the shadow’s disk subtends an angle of about 1.4°; its centre is always opposite the Sun’s disk and travels along the [ecliptic](https://www.britannica.com/science/ecliptic), EE′. A [lunar eclipse](https://www.britannica.com/science/lunar-eclipse) occurs whenever the shadow’s disk overlaps the Moon’s disk; this happens only when the shadow’s disk is near one of the nodes and the Sun is near the opposite node. The Sun’s passage through the lunar nodes is thus the critical time for both solar and lunar [eclipses](https://www.britannica.com/dictionary/eclipses). The plane of the Moon’s path, LL′, is not fixed, and its nodes move slowly along the ecliptic in the direction indicated by the arrows, making a complete revolution in about 19 years. The interval between two successive passages of the Sun through one of the nodes is termed an eclipse year, and, since the Moon’s node moves so as to meet the advancing Sun, this interval is about 18.6 days less than a tropical (or ordinary) year.
[](https://cdn.britannica.com/32/132-004-E4C16744/node-orbit-observer-Moon-Earth-Conditions-lunar.jpg)
[graph depicting the ascending node of the Moon's orbit as seen from an observer on Earth](https://cdn.britannica.com/32/132-004-E4C16744/node-orbit-observer-Moon-Earth-Conditions-lunar.jpg)
Ascending node of the Moon's orbit as seen from an observer on Earth (the centre of the celestial sphere). Conditions necessary for a solar eclipse (top) and a lunar eclipse (bottom) are shown. (_See_ the text.)(more)
In the figure of the Moon’s ascending node, this region is depicted as seen from the centre of the [celestial](https://www.britannica.com/dictionary/celestial) sphere and is shown much enlarged. The node is kept fixed, and the apparent motions of the Sun and the Moon (top portion of the figure) are shown relative to the node. To the observer on Earth at the centre of the sphere, the Sun’s disk will travel along the ecliptic, EE′, and the Moon’s disk along its designated path, LL′. The Sun is so distant compared with the size of Earth that, from all places on Earth’s surface, the Sun is seen nearly in the same position as it would be from the very centre. On the other hand, the Moon is relatively near, and so its projected position on the celestial sphere is different for various places of observation on Earth. In fact, it may be displaced as much as 1° from the position in which it is seen from the centre of Earth. If the radius of the Moon’s disk is enlarged by 1°, a “Moon circle,” C, is obtained that encloses all possible positions of the Moon’s disk seen from anywhere on Earth. Conversely, if any disk of the Moon’s size is placed inside this Moon circle, there is a place on Earth from which the Moon is seen in that position.
Accordingly, an eclipse of the Sun occurs somewhere on Earth whenever the Moon overtakes the Sun in such a position that the Moon circle, C, passes over the Sun’s disk; when the latter is entirely covered by the Moon circle, the eclipse will be total or annular. From the top portion of the figure of the Moon’s ascending node, it is evident that a [solar eclipse](https://www.britannica.com/science/solar-eclipse) will take place if a new moon occurs while the Sun moves from position S1 to position S4. This period is the eclipse season; it starts 19 days before the Sun passes through a lunar node and ends 19 days thereafter. There are two complete eclipse seasons, one at each node, during a calendar year. Because there is a new moon every month, at least one solar eclipse, and occasionally two, occurs during each eclipse season. A fifth solar eclipse during a calendar year is possible because part of a third eclipse season may occur at the beginning of January or at the end of December.
The bottom portion of the figure of the Moon’s [ascending](https://www.britannica.com/dictionary/ascending) node illustrates the condition necessary for a lunar eclipse. If a [full moon](https://www.britannica.com/science/full-Moon-lunar-phase) occurs within 13 days of the passage of the Sun though a lunar node—and thus of the Earth’s umbral disk, U, through the opposite node—the Moon will be eclipsed. (In the figure the umbral disk passes through the ascending node.) Most eclipse seasons, but not all, will thus also contain a lunar eclipse. When two eclipse seasons and a partial third season fall in a calendar year, there may be three lunar eclipses in that year. Eclipses of the Sun are evidently more frequent than those of the Moon. Solar eclipses, however, can be seen from only a very limited region of Earth, whereas lunar eclipses are visible from an entire hemisphere.
During a solar eclipse the shadow cones—the umbra and penumbra—of the Moon sweep across the face of Earth (_see_ the figure of an eclipse of the Sun), while, at the same time, Earth is rotating on its axis. Within the narrow area covered by the umbra, the eclipse is total. Within the wider surrounding region covered by the [penumbra](https://www.britannica.com/science/penumbra-eclipse), the eclipse is partial.
Astronomical [ephemerides](https://www.britannica.com/science/ephemeris), or tables, that are published annually for the year ahead provide maps tracing the paths of the more important eclipses in considerable detail, as well as data for accurate calculation of the times of contact at any given observing location on Earth. Calculations are made some years ahead in [Terrestrial Time](https://www.britannica.com/science/Ephemeris-Time) (TT), which is defined by the orbital motion of Earth and the other planets. At the time of the eclipse, the correction is made to [Universal Time](https://www.britannica.com/science/Universal-Time) (UT), which is defined by the rotation of Earth and is not rigorously uniform.
Modern computers make it possible to predict solar eclipses several years ahead with high accuracy. By means of the same calculational methods, eclipses can be “predicted backward” in time. The generation of the times and observational locations for ancient eclipses has been valuable in historical and scientific research (_see below_ [Eclipses in history](https://www.britannica.com/science/eclipse/Eclipsing-binary-stars#ref11214)).
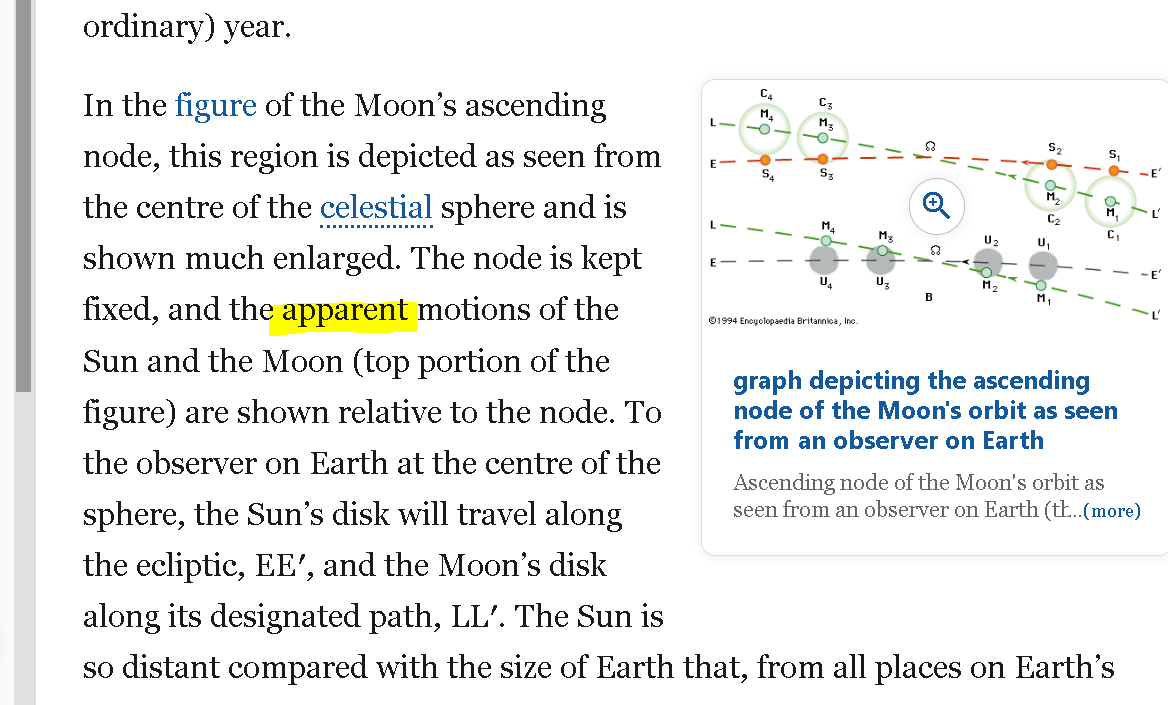
#### LUNAR LIMB IS NOT USED IN ALL MAPS
[https://eclipsewise.com/solar/SEhelp/SEradius.html](https://eclipsewise.com/solar/SEhelp/SEradius.html)
#### Mean Lunar Radius 'k'
A fundamental parameter used in the prediction of solar eclipses is the Moon's mean radius 'k', expressed in units of Earth's equatorial radius. The actual radius of the Moon varies as a function of position angle and libration due to the irregularity of the lunar limb profile. From 1968 through 1980, the United States Naval Observatory Nautical Almanac Office (USNO) used two separate values for k in their eclipse predictions. The larger value (k=0.2724880), representing a mean over lunar topographic features, was used for all penumbral (i.e., exterior) contacts and for annular eclipses. A smaller value (k=0.272281), representing a mean minimum radius, was reserved exclusively for umbral (i.e., interior) contact calculations of total eclipses (Explanatory Supplement, 1974). Unfortunately, the use of two different values of k for central eclipses introduces a discontinuity in the case of hybrid or annular-total eclipses.
In August 1982, the International Astronomical Union (IAU) General Assembly adopted a value of k=0.2725076 for the mean lunar radius. This value is currently used by USNO for all solar eclipse predictions (Fiala and Lukac, 1983) and is believed to be the best mean radius, averaging mountain peaks and low valleys along the Moon's rugged limb. The adoption of one single value for k eliminates the discontinuity in the case of annular-total (i.e., hybrid) eclipses and ends confusion arising from the use of two different values. However, the use of even the best 'mean' value for the Moon's radius introduces a problem in predicting the character and duration of central eclipses, particularly total eclipses. A total eclipse can be defined as an eclipse in which the Sun's disk is completely occulted by the Moon. This cannot occur so long as any photospheric rays are visible through deep valleys along the Moon's limb (Meeus, Grosjean and Vanderleen, 1966). But the use of the IAU's mean k guarantees that some annular or annular-total eclipses will be misidentified as total. A case in point is the eclipse of 1986 Oct 03. The Astronomical Almanac for 1986 identified this event as a total eclipse of 3 seconds duration when in it was in fact a beaded annular eclipse. Clearly, a smaller value of k is needed since it is more representative of the deepest lunar valley floors, hence the minimum solid disk radius, and ensures that an eclipse is truly total.
Of primary interest to most observers are the times when central eclipse begins and ends (second and third contacts, respectively) and the duration of the central phase. When the IAU's mean value for k is used to calculate these times, they must be corrected to accommodate low valleys (total) or high mountains (annular) along the Moon's limb. The calculation of these corrections is not trivial, but is essential, especially if one plans to observe near the path limits (Herald, 1983). For observers near the central line of a total eclipse, the limb corrections can be closely approximated by using a smaller value of k, which accounts for valleys along the profile.
Solar eclipse predictions appearing on [EclipseWise.com](https://eclipsewise.com/eclipse.html "Home") use the IAU's accepted value of k (k=0.2725076) for all penumbral (exterior) contacts. However, the predictions here depart from IAU convention by adopting the smaller value for k (k=0.272281) for all central (interior) contacts. This is done in order to avoid eclipse type misidentification and to predict central durations that are closer to the actual durations observed at total eclipses.
Using this smaller value of k for central eclipses is consistent with predictions published in [**Thousand Year Canon of Solar Eclipses**](https://eclipsewise.com/pubs/TYCSE.html) and [**Five Millennium Canon of Solar Eclipses**](https://eclipsewise.com/solar/SEpubs/5MCSE.html). Consequently, the smaller k produces shorter central durations and narrower paths for total eclipses when compared with calculations using the IAU value for k. Similarly, the smaller k predicts longer central durations and wider paths for annular eclipses.
#### Duration of Total Eclipse with Different Values of k
A recent example of eclipse predictions for the total solar eclipse of 2017 August 21 using different values of k can be seen in the following table. They show the predicted duration of totality on the central line in Shawnee National Forest, Illinois (89°, 15' 24" W & 37° 38' 12" N). This is near the position where the total eclipse lasts the longest along the entire eclipse path.
1. 2 minutes 44.3 seconds from [USNO](http://aa.usno.navy.mil/data/docs/Eclipse2017.php) (using IAU value of k [k=0.2725076])
2. 2 minutes 40.3 seconds from [EclipseWise.com - 2017 Total Solar Eclipse](https://eclipsewise.com/solar/SEnews/TSE2017/TSE2017.html) (using smaller value of k [k=0.272281])
3. 2 minutes 41.5 seconds from [Eclipse Bulletin: Total Solar Eclipse of 2017](https://eclipsewise.com/pubs/TSE2017.html) (using actual lunar limb profile)
4. 2 minutes 41.4 seconds from [2017 Interactive Eclipse Map (X. Jubier)](http://xjubier.free.fr/en/site_pages/solar_eclipses/TSE_2017_GoogleMapFull.html) (using actual lunar limb profile)
_There is to right or wrong answer in the above calculations__._ **They** simply use different assumptions for calculating the duration of totality at a given point and they have different levels of accuracy. That being said, 3) and 4) are the most precise predictions. They are in near agreement in spite of the fact that they were calculated totally independent of each other with completely different software.
[http://xjubier.free.fr/en/site_pages/solar_eclipses/5MCSE/xSE_Five_Millennium_Canon.html](http://xjubier.free.fr/en/site_pages/solar_eclipses/5MCSE/xSE_Five_Millennium_Canon.html)
[https://www.eclipsewise.com/solar/SEanim400/2022_04_30_PSE_400px.gif](https://www.eclipsewise.com/solar/SEanim400/2022_04_30_PSE_400px.gif)
NASA removed the easy to understand catalogues it seems.
## Catalog of Solar Eclipses of Saros 135
```
TD of
```
Seq. Rel. Calendar Greatest Luna Ecl. Ecl. Sun Path Central
Num. Num. Date Eclipse ΔT Num. Type Gamma Mag. Lat Long Alt Width Dur.
s ° ° ° km
07934 -34 1331 Jul 05 22:46:38 427 -8268 Pb 1.5532 0.0063 67.8N 12.6E 0
07975 -33 1349 Jul 16 05:25:45 396 -8045 P 1.4782 0.1384 68.8N 98.7W 0
08016 -32 1367 Jul 27 12:05:47 366 -7822 P 1.4043 0.2679 69.7N 149.3E 0
08057 -31 1385 Aug 06 18:51:40 339 -7599 P 1.3352 0.3878 70.6N 35.3E 0
08097 -30 1403 Aug 18 01:41:42 313 -7376 P 1.2697 0.5006 71.3N 80.4W 0
08137 -29 1421 Aug 28 08:38:54 288 -7153 P 1.2101 0.6025 71.8N 161.6E 0
08177 -28 1439 Sep 08 15:42:20 265 -6930 P 1.1555 0.6947 72.1N 41.6E 0
08217 -27 1457 Sep 18 22:54:59 243 -6707 P 1.1083 0.7737 72.1N 80.8W 0
08257 -26 1475 Sep 30 06:15:14 222 -6484 P 1.0676 0.8411 71.9N 154.9E 0
08297 -25 1493 Oct 10 13:43:35 202 -6261 P 1.0334 0.8969 71.4N 28.8E 0
08338 -24 1511 Oct 21 21:19:49 184 -6038 A+ 1.0058 0.9416 70.7N 98.6W 0
08380 -23 1529 Nov 01 05:04:11 167 -5815 An 0.9846 0.9119 61.7N 122.8E 9 - 08m09s
08421 -22 1547 Nov 12 12:54:24 152 -5592 A 0.9683 0.9106 55.5N 4.7W 14 1419 08m59s
08462 -21 1565 Nov 22 20:49:55 138 -5369 A 0.9564 0.9092 51.4N 130.5W 16 1220 09m37s
08503 -20 1583 Dec 14 04:48:39 127 -5146 A 0.9471 0.9083 48.5N 104.1E 18 1116 10m03s
08544 -19 1601 Dec 24 12:50:31 116 -4923 A 0.9402 0.9078 46.6N 21.5W 19 1051 10m14s
08586 -18 1620 Jan 04 20:51:05 94 -4700 A 0.9321 0.9081 45.0N 146.5W 21 976 10m13s
08630 -17 1638 Jan 15 04:51:53 67 -4477 A 0.9242 0.9090 44.0N 88.9E 22 907 10m00s
08676 -16 1656 Jan 26 12:48:10 40 -4254 A 0.9122 0.9106 43.2N 34.1W 24 820 09m38s
08722 -15 1674 Feb 05 20:41:35 19 -4031 A 0.8979 0.9129 42.8N 155.7W 26 736 09m09s
08767 -14 1692 Feb 17 04:26:56 8 -3808 A 0.8765 0.9159 42.4N 85.6E 28 644 08m36s
08812 -13 1710 Feb 28 12:07:29 9 -3585 A 0.8509 0.9194 42.5N 31.2W 31 562 08m00s
08857 -12 1728 Mar 10 19:38:56 10 -3362 A 0.8172 0.9233 42.8N 144.6W 35 485 07m25s
08903 -11 1746 Mar 22 03:02:49 12 -3139 A 0.7771 0.9277 43.5N 104.7E 39 419 06m51s
08948 -10 1764 Apr 01 10:17:15 15 -2916 A 0.7288 0.9323 44.2N 2.5W 43 361 06m20s
08994 -09 1782 Apr 12 17:24:47 17 -2693 A 0.6745 0.9370 45.1N 107.1W 47 311 05m51s
09039 -08 1800 Apr 24 00:24:00 13 -2470 A 0.6125 0.9417 45.7N 151.3E 52 269 05m27s
09084 -07 1818 May 05 07:15:49 12 -2247 A 0.5440 0.9464 45.8N 52.5E 57 233 05m05s
09129 -06 1836 May 15 14:01:39 5 -2024 A 0.4700 0.9509 45.1N 44.4W 62 203 04m47s
09173 -05 1854 May 26 20:42:53 7 -1801 A 0.3918 0.9551 43.3N 140.1W 67 178 04m32s
09216 -04 1872 Jun 06 03:20:03 -1 -1578 A 0.3095 0.9590 40.5N 124.8E 72 157 04m20s
09258 -03 1890 Jun 17 09:55:05 -6 -1355 A 0.2246 0.9625 36.5N 29.3E 77 140 04m09s
09300 -02 1908 Jun 28 16:29:51 8 -1132 A 0.1389 0.9655 31.4N 67.2W 82 126 04m00s
09342 -01 1926 Jul 09 23:06:02 24 -909 A 0.0538 0.9680 25.6N 165.1W 87 115 03m51s
09385 00 1944 Jul 20 05:43:13 27 -686 A -0.0314 0.9700 19.0N 95.7E 88 108 03m42s
09425 01 1962 Jul 31 12:25:33 34 -463 Am -0.1130 0.9716 12.0N 5.7W 84 103 03m33s
09465 02 1980 Aug 10 19:12:21 51 -240 A -0.1915 0.9727 4.6N 108.9W 79 100 03m23s
09504 03 1998 Aug 22 02:07:11 63 -17 A -0.2644 0.9734 3.0S 145.4E 75 99 03m14s
09544 04 2016 Sep 01 09:08:02 70 206 A -0.3330 0.9736 10.7S 37.8E 70 100 03m06s
09584 05 2034 Sep 12 16:19:28 81 429 A -0.3936 0.9736 18.2S 72.6W 67 102 02m58s
Catalog of Solar Eclipses of Saros 135
TD of
Seq. Rel. Calendar Greatest Luna Ecl. Ecl. Sun Path Central
Num. Num. Date Eclipse ΔT Num. Type Gamma Mag. Lat Long Alt Width Dur.
s ° ° ° km
09624 06 2052 Sep 22 23:39:10 98 652 A -0.4480 0.9734 25.7S 175.0E 63 106 02m51s
09666 07 2070 Oct 04 07:08:57 136 875 A -0.4950 0.9731 32.8S 60.4E 60 110 02m44s
09707 08 2088 Oct 14 14:48:05 177 1098 A -0.5349 0.9727 39.7S 56.0W 57 115 02m38s
09748 09 2106 Oct 26 22:37:40 219 1321 A -0.5671 0.9725 45.9S 174.1W 55 119 02m32s
09789 10 2124 Nov 06 06:36:34 263 1544 A -0.5921 0.9724 51.6S 66.8E 53 123 02m26s
09830 11 2142 Nov 17 14:43:08 309 1767 A -0.6117 0.9727 56.4S 52.4W 52 124 02m19s
09872 12 2160 Nov 27 22:58:32 351 1990 A -0.6247 0.9734 60.1S 171.6W 51 123 02m12s
09915 13 2178 Dec 09 07:20:02 392 2213 A -0.6338 0.9745 62.4S 69.9E 50 118 02m03s
09959 14 2196 Dec 19 15:47:09 434 2436 A -0.6387 0.9761 63.1S 48.6W 50 111 01m53s
10003 15 2215 Jan 01 00:16:36 478 2659 A -0.6427 0.9783 62.3S 168.0W 50 101 01m41s
10047 16 2233 Jan 11 08:49:17 525 2882 A -0.6447 0.9811 60.0S 70.4E 50 88 01m28s
10092 17 2251 Jan 22 17:21:41 573 3105 A -0.6480 0.9844 56.9S 53.2W 49 72 01m12s
10137 18 2269 Feb 02 01:53:06 624 3328 A -0.6529 0.9883 53.2S 178.2W 49 54 00m54s
10183 19 2287 Feb 13 10:21:25 677 3551 A -0.6613 0.9926 49.4S 56.3E 48 34 00m35s
10228 20 2305 Feb 24 18:46:09 732 3774 A -0.6732 0.9973 45.7S 69.3W 47 13 00m13s
10273 21 2323 Mar 08 03:05:10 788 3997 H -0.6906 1.0023 42.4S 166.1E 46 11 00m11s
10318 22 2341 Mar 18 11:18:20 847 4220 H -0.7137 1.0075 39.8S 42.6E 44 36 00m36s
10364 23 2359 Mar 29 19:24:46 908 4443 T -0.7429 1.0128 37.9S 79.3W 42 64 01m02s
10408 24 2377 Apr 09 03:25:10 971 4666 T -0.7779 1.0180 37.1S 160.2E 39 96 01m28s
10452 25 2395 Apr 20 11:17:15 1037 4889 T -0.8203 1.0230 37.7S 41.9E 35 134 01m52s
10495 26 2413 Apr 30 19:03:57 1104 5112 T -0.8677 1.0274 40.0S 75.0W 30 183 02m13s
10538 27 2431 May 12 02:43:30 1173 5335 T -0.9214 1.0310 44.8S 170.4E 22 267 02m27s
10581 28 2449 May 22 10:19:15 1244 5558 T -0.9790 1.0328 54.4S 59.1E 11 567 02m24s
10624 29 2467 Jun 02 17:48:24 1318 5781 P -1.0425 0.9315 64.5S 50.8W 0
10666 30 2485 Jun 13 01:16:18 1393 6004 P -1.1075 0.8095 65.4S 172.3W 0
10708 31 2503 Jun 25 08:40:22 1471 6227 P -1.1759 0.6800 66.4S 66.7E 0
10749 32 2521 Jul 05 16:04:53 1551 6450 P -1.2445 0.5492 67.4S 54.7W 0
10790 33 2539 Jul 16 23:27:49 1632 6673 P -1.3148 0.4143 68.3S 176.3W 0
10830 34 2557 Jul 27 06:54:08 1716 6896 P -1.3827 0.2835 69.3S 60.9E 0
10870 35 2575 Aug 07 14:21:38 1802 7119 P -1.4499 0.1541 70.1S 62.9W 0
10910 36 2593 Aug 17 21:53:04 1890 7342 Pe -1.5141 0.0303 70.8S 171.9E 0
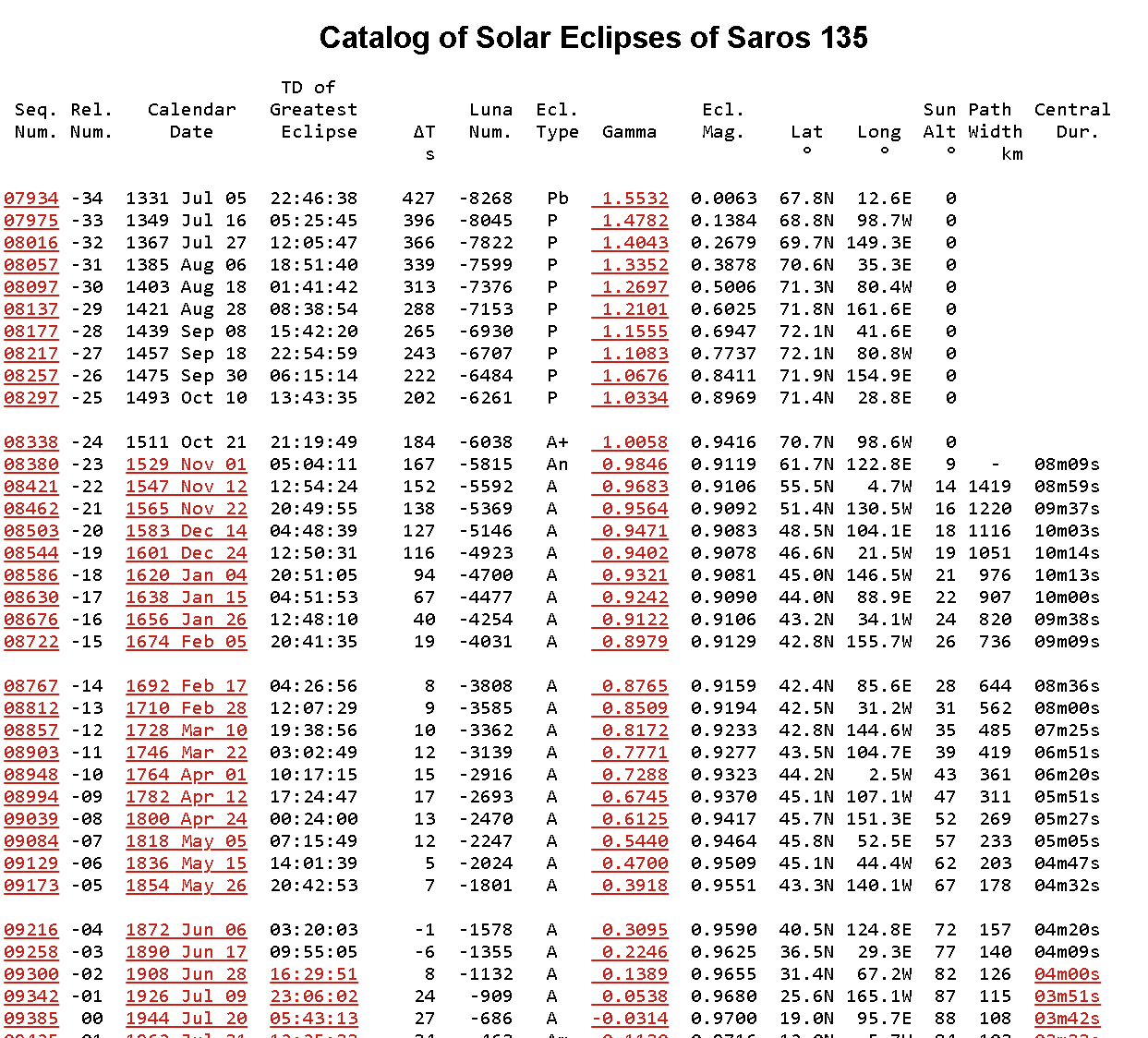

Links to this page
[Eclipse Sources](https://publish.obsidian.md/shanesql/Eclipse+Sources)
[Sun and Moon](https://publish.obsidian.md/shanesql/Sun+and+Moon)